A uniform rod of mass m and length l is taken. Find the gravitational field intensity at point P at distance d which is on the perpendicular bisector of the rod as shown in Fig.
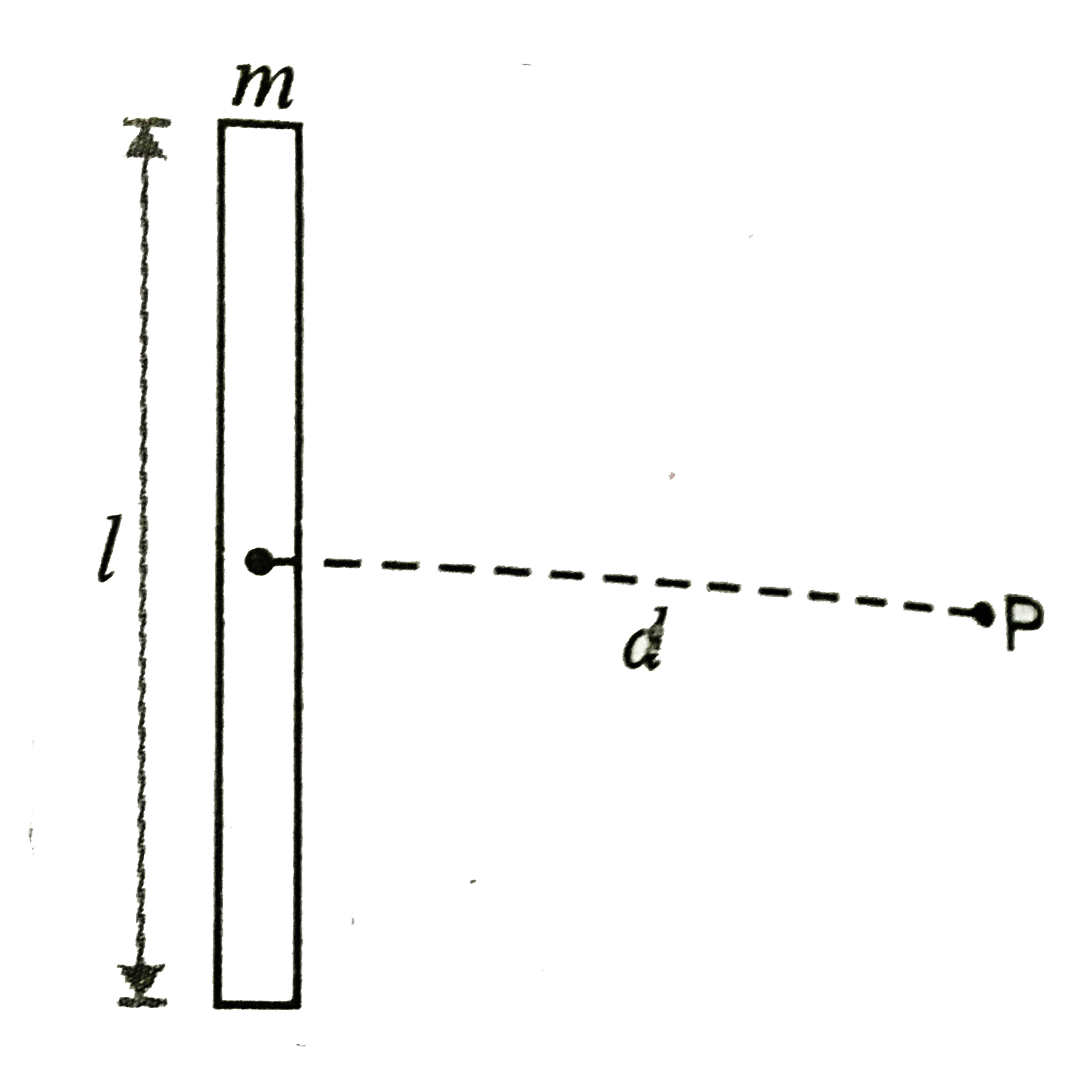
4Gmd√4d2+l2
2Gmd√l2+4d2
2√2Gmd√l2+4d2
none of the above
The correct Answer is:B
Consider element of the rod of width x as shown in Fig.
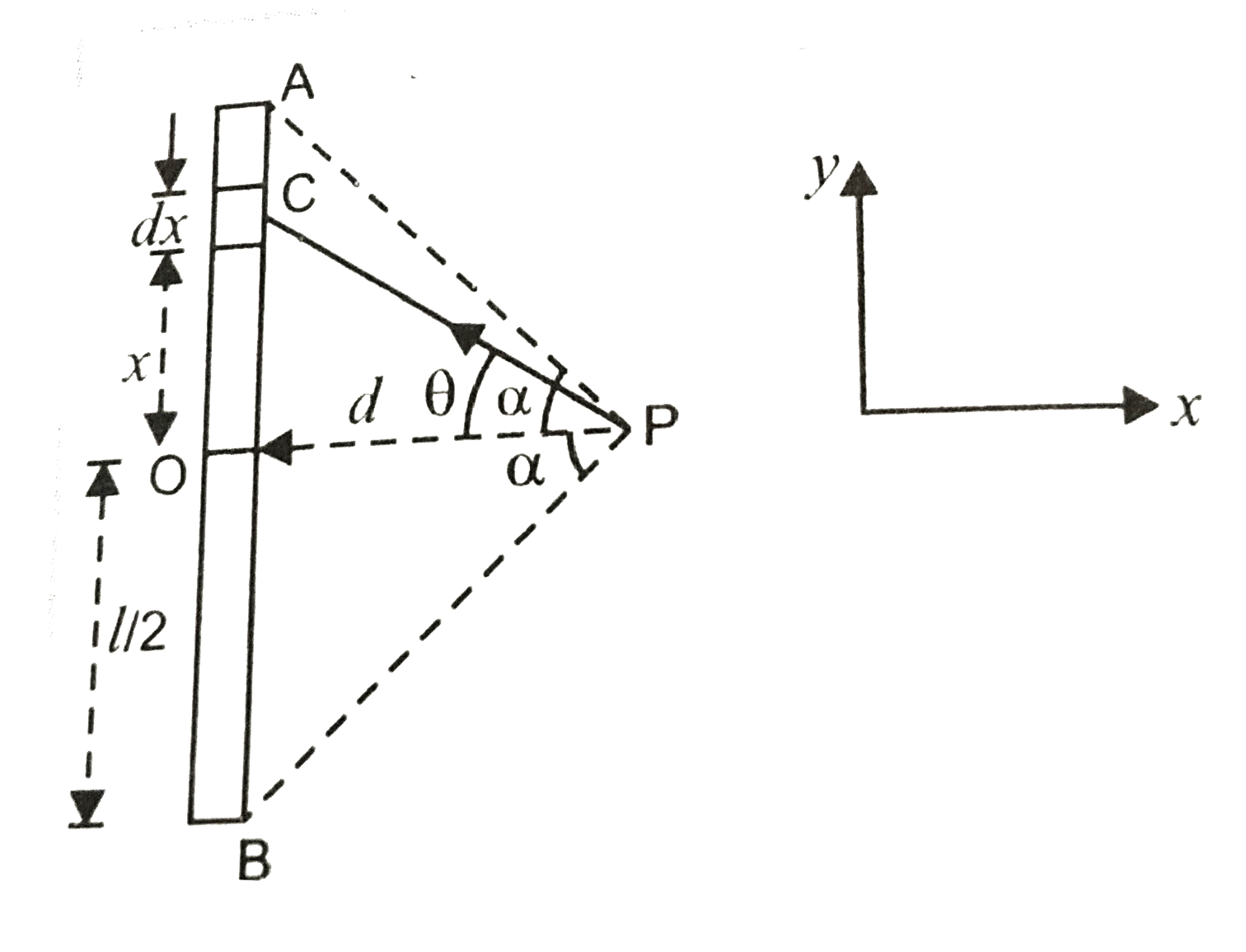
Mass of element, dm=mldx
Gravitaitonal field intensity at P due to this element is
dI=Gdmx2+d2=G(x2+d2)×mldx along PC
In δOPC,x=dtanθ and dx=dsec2θdθ
∴x2+d2=d2(tan2θ+1)=d2sec2θ
∴dI=Gmldsec2θdθd2sec2θ=Gmlddθ
resolving d→I into two rectangular components we have dIcosθ acts along PO and dIsinθ acts perpendicular to PO.
If we find the gravitaitonal field due to other elements of the rod at P and resolve them into two rectangular componets, we note that the components of gravitational field intensity perpendicular to OP will cancel out and components of gravitational intensity along PO will be added up. Therefore, total gravitational intensity at P due to entire rod is
I=∫+α−αdIcosθ=∫+α−αGmldcosθdθ
=Gmld[2sinα]=2Gmld×l/2√(l/2)2+d2
=2Gmd√l2+4d2
Similar Questions
A uniform rod of mass M and length L lies on a frictionless horizontal plane. A particle of same mass M moving with speed v0 perpendicular to the length of the rod strikes the end of the rod and sticks to it. Find the velocity of the center of mass and the angular velocity about the center of mass of (rod + particle) system.
View SolutionA uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B perpendicular to the rod in horizontal direction. Speed of the point A of the rod after giving impulse is:
View Solution
Knowledge Check
A thin uniform rod of mass M and length L. Find the radius of gyration for rotation about an axis passing through a point at a distance ofL4 from centre and perpendicular to rod.
A√748LB√548LC√724LD√1924LA uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B, perpendicular to the rod in the horizontal direction. Speed of particlem P at a distance l6 from the centre towards A of the rod after time t=πml12J is.
A2JmBJ√2mCJmD√2JmA uniform rod of mass M and length L carrying a charge q uniformly distributed over its length is rotated with constant velocity w about its mid point perpendicular to the rod. Its magnetic moment is
AqωL2/6BqωL2/12CqωL2/24DqωL2/48
The radius of gyration of a uniform rod of mass m and length l about an axis perpendicular to its length and at distance l4 from its one end will be
View SolutionA uniform rod of mass m and length L is fixed to an axis, making an angle θ with it as shown in the figure. The rod is rotated about this axis so that the free end of the rod moves with a uniform speed ‘v’. Find the angular momentum of the rod about the axis. Is the angular momentum of the rod about point A constant?
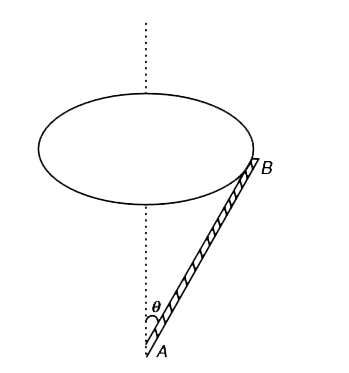
View SolutionA uniform rod of mass M and length a lies on a smooth horizontal plane. A particle of mass m moving at a speed v perpendicular to the length of the rod strikes it at a distance a4 from the centre and stops after the collision. Find a. the velocity of the cente of the rod and b. the angular velocity of the rod abut its centre just after the collision.
View SolutionA uniform rod of mass M and length l is hinged at point O and is free to rotate on a horizontal smooth surface. Point O is at a distance of l4 from one end of the rod. A sharp impulse P0=2√130kgm/s is applied along the surface at one end of the rod as shown in figure [tanθ=97]
(a) Find the angular speed of the rod immediately after the hit
(b) Find the impulse on the rod due to the hinge.

View SolutionFind the potential energy of the gravitational interation of a point mass of mass m and a thin uniform rod of mass M and length l, if they are located along a straight line such that the point mass is at a distance 'a' from one end. Also find the force of their interaction.
View Solution
