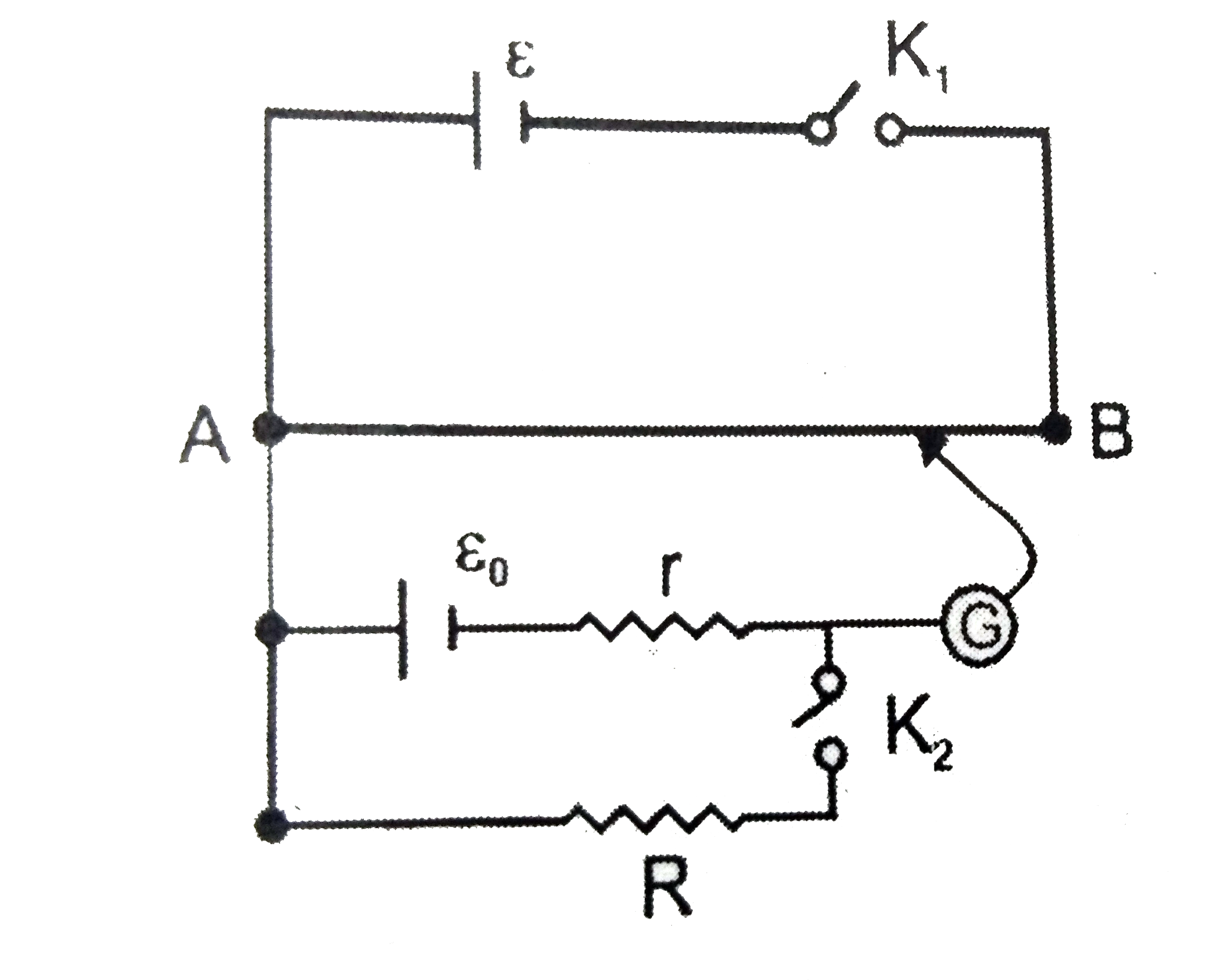
A potentiometer circuit has been setup for finding. The internal resistance of a given cell. The main battery used a negligible internal resistance. The potentiometer wire itself is 4m long. When the resistance, R, connected across the given cell, has value of
(i) Infinity 9.5Ω,
(ii) the 'balancing length' , on the potentiometer wire are found to be 3m and 2.85m, respectively.
The value of internal resistance of the cell is
0.25Ω
0.95Ω
0.5Ω
0.75Ω
The correct Answer is:C
To find the internal resistance of the given cell using the potentiometer method, we can follow these steps:
Step 1: Understand the given data
- The potentiometer wire length is 4m.
- When the resistance R is infinite, the balancing length L1 is 3m.
- When the resistance R is 9.5Ω, the balancing length L2 is 2.85m.
- The EMF of the cell and the main battery is 2V (as inferred from the context).
Step 2: Use the formula for internal resistance
The formula for internal resistance r of the cell is given by:
r=(L1L2−1)R
Where:
- L1 is the balancing length when R is infinite.
- L2 is the balancing length when R is 9.5Ω.
- R is the external resistance connected across the cell.
Step 3: Substitute the values into the formula
Substituting the known values into the formula:
r=(3m2.85m−1)×9.5Ω
Step 4: Calculate L1L2
Calculating L1L2:
L1L2=32.85≈1.0526
Step 5: Calculate L1L2−1
Now, calculate L1L2−1:
L1L2−1≈1.0526−1=0.0526
Step 6: Calculate the internal resistance r
Now, substitute this back into the equation for r:
r=0.0526×9.5≈0.5Ω
Final Answer
The internal resistance of the cell is approximately 0.5Ω.
---
- The potentiometer wire length is 4m.
- When the resistance R is infinite, the balancing length L1 is 3m.
- When the resistance R is 9.5Ω, the balancing length L2 is 2.85m.
- The EMF of the cell and the main battery is 2V (as inferred from the context).
The formula for internal resistance r of the cell is given by:
- L1 is the balancing length when R is infinite.
- L2 is the balancing length when R is 9.5Ω.
- R is the external resistance connected across the cell.
Substituting the known values into the formula:
Calculating L1L2:
Now, calculate L1L2−1:
Now, substitute this back into the equation for r:
The internal resistance of the cell is approximately 0.5Ω.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Section - C
1 Videos
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - AIIMS Questions
36 Videos
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Section B - Assertion Reasoning
28 Videos
- ATOMIC PHYSICSBOOK - A2ZCHAPTER - ATOMIC PHYSICSEXERCISE - Section D - Chapter End Test
30 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
A potentiometer circuit with potentiometer wire 10 m long, and an ideal main battery of e.m.f 8 V, has been set up for finding the internal resistance of a given cell. When the resistance R, connected across the given cell, has values of (i) infinity, (ii) 10 omega , the balancing lengths, on the potentiometer wire are found to be 2.4 m and x respectively. If the internal resistance of the cell is 2 omega, the value of x is
View SolutionWhen a balance point is obtained in a potentiometer for finding the internal resistance of a cell, the current through the potentiometer wire is due to
View Solution
Knowledge Check
A potentiometer circuit has been set up for finding the internal resistance of a given cell. The main battery, used across the potentiometer wire , has an emf of 2.0 v and a negligible internal resistance .The potentiometer wire itself is 4 m long . When the resistance , R connected across the given cell, has values of (i) Infinity , (ii) 9.5Ω the balancing length's on the potentiometer wire are found to be 3 m and 2.85 m , respectively . The value of internal resistance of the cell is .
A0.25ΩB0.95ΩC0.5ΩD0.75ΩThe internal resistance of a cell by potentiometer is given by
Ar1=R1(l1−l2l2)Br1=R1(E1−V1V1)Cboth 'a' and 'b'Dneither 'a' nor 'b'In an experiment to find the internal resistance of a cell by a potentiometer, a balance was obtained for 50 cm length of the potentiometer wire, with a cell of e.m.f. 2V. When the cell was shunted by a resistance of 2Ω, the balancing length of the potentiometer wire was 40 cm. What was the internal resistance of the cell ?
A0.25ΩB0.75ΩC0.5ΩD1Ω
The internal resistance of a cell is determined by using a potentiometer. In an experimetn, an external resistance of 60Ω is used across the given cell. When the key is closed, the balance length on the potentiometer decreases form 72 cm to 60 cm. calculate the internal resistance of the cell.
View SolutionA potentiometer wire is 10 m long and has a resistance of 18Ω. It is connected to a battery of emf 5 V and internal resistance 2Ω. Calculate the potential gradient along the wire.
View SolutionA potentiometer wire has a length of 4 and a resistance of 8 ohm. It is connected in series with a cell of e.m.f. 2 V and internal resistance 2 ohm. The potential drop per unit length of the wire is
View SolutionA potentiometer wire has length 10m and resistance 10Ω. It is connected to battery of EMF 11 volt and internal resistance 1Ω, then the potential gradient in the wire is
View SolutionA potentiometer wire has length 10 m and resistance 20Ω . A 2. 5 V battery of negligible internal resistance is connected across the wire with an 80Ω series resistance. The potential gradient on the wire will be
View Solution
