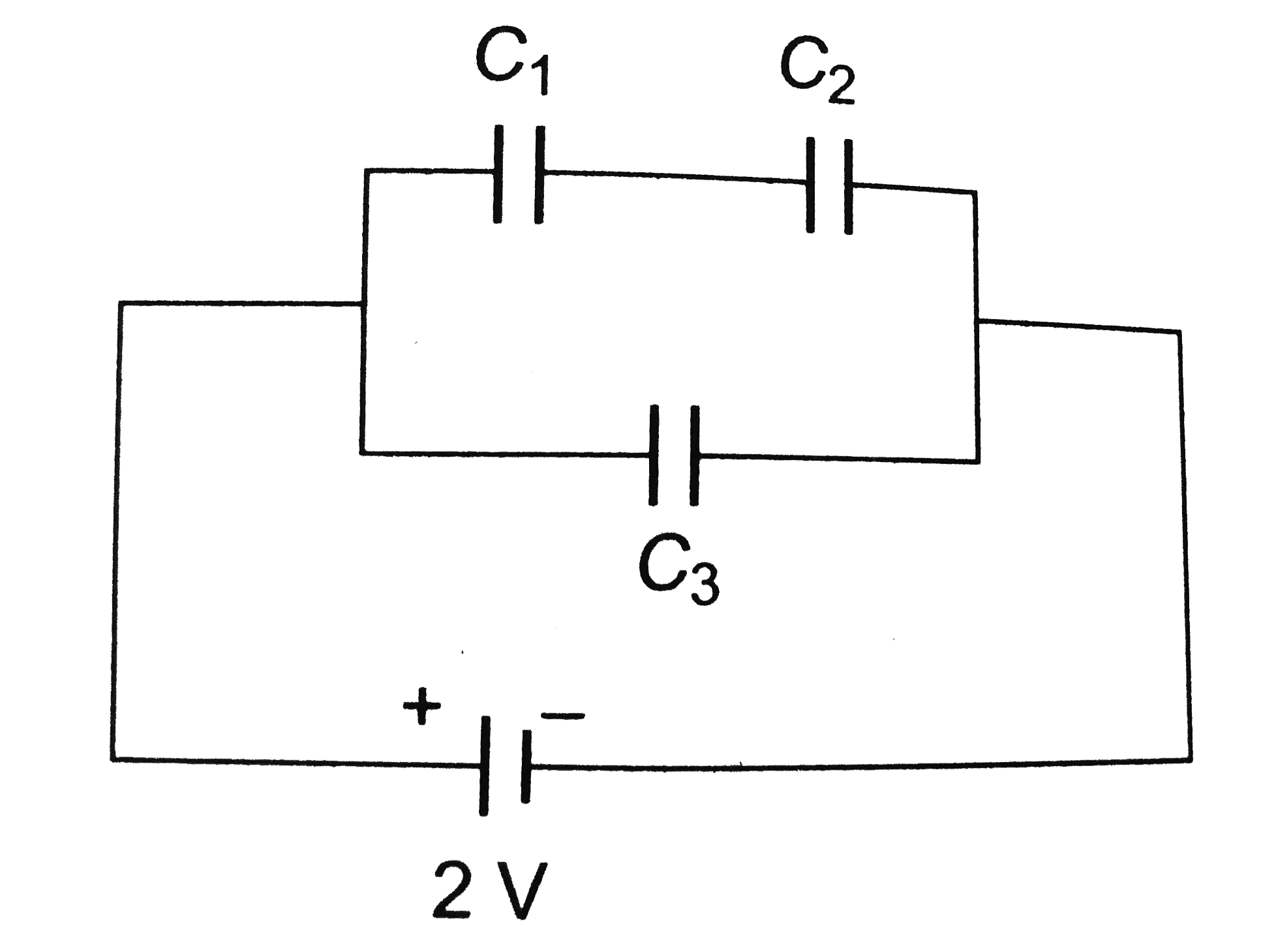
Two capacitors C1=2μF and C2=6μF in series, are connected in parallel to a third capacitor C3=4μF. This arrangement is then connected to a battery of e.m.f =2V, as shown in the figure. How much energy is lost by the battery in charging the capacitors
22×10−6J
11×10−6J
(323)×10−6J
(163)×10−6J
The correct Answer is:B
Ceq=C1C2C1+C2+C3=2×62+6+4=5.5μF
Energy supplied (E)=QV=CV2=22×10−6J
P.E. stored
(U)=12CeqV2=12×5.5×(2)2=11×10−6J
⇒ Energy lost =E−U=11×10−6J
Topper's Solved these Questions
- ELECTRIC POTENTIAL & CAPACITANCEBook:A2ZChapter:ELECTRIC POTENTIAL & CAPACITANCEExercise:Problems Based On Mixed Concepts
Explore 44 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBook:A2ZChapter:ELECTRIC POTENTIAL & CAPACITANCEExercise:Section B - Assertion Reasoning
Explore 17 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBook:A2ZChapter:ELECTRIC POTENTIAL & CAPACITANCEExercise:Grouping Of Capacitors
Explore 48 Videos
- ELECTRIC CHARGE, FIELD & FLUXBook:A2ZChapter:ELECTRIC CHARGE, FIELD & FLUXExercise:Section D - Chapter End Test
Explore 29 Videos
- ELECTROMAGNETIC INDUCTIONBook:A2ZChapter:ELECTROMAGNETIC INDUCTIONExercise:Section D - Chapter End Test
Explore 30 Videos
Similar Questions
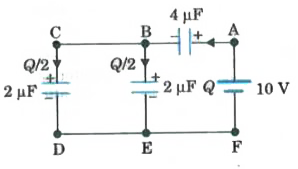
Three capacitors of capacitances 2μF,2μFand4μF are connected to a battery of 10V as shown in the figure. Calculate the effective capacitance of the circuit and charge on each capacitor.
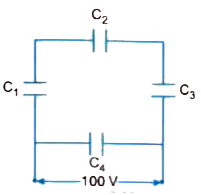
View SolutionA network of four capacitors, each of capacitance 15μF,is connected across a battery of 100 V as shown in the figure. Find the net capacitance and the charge on the capacitor C4.
View Solution
Knowledge Check
Two capacitors C1=3μF and C2=6μF in series, are connected in parallel to a third capacitor C3=4μF. This arrangement is then connected to a battery of e.m.f., =30V, as shown. The energy lost by the battery in charging the capacitors
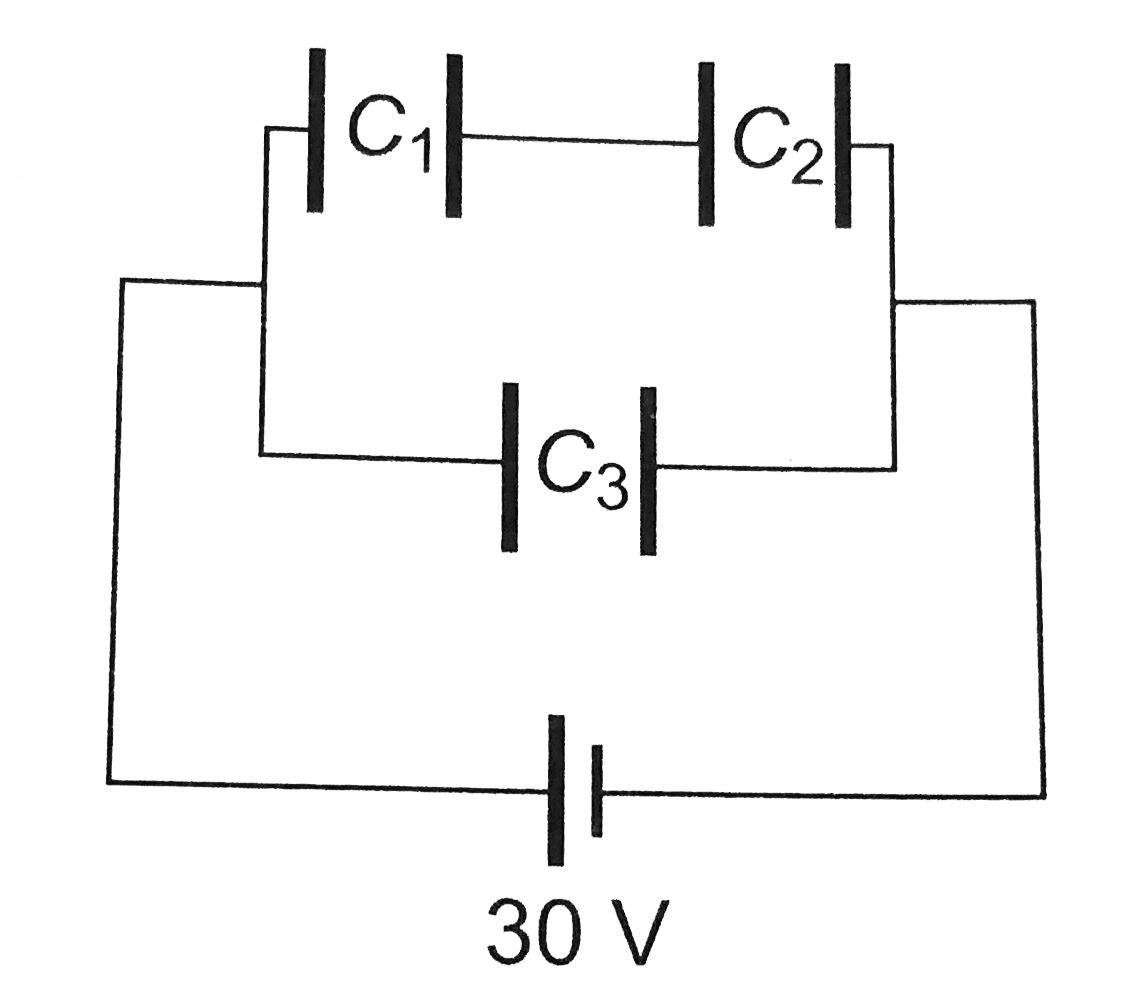 A900μJB1800μJC2700μJD3600μJ
A900μJB1800μJC2700μJD3600μJTwo capacitors 2μF and 4μF are connected in parallel. A third capacitor of 6μFis connected in series. The combination is then connected across a 12V battery. The voltage across 2μF capacitor is:
A2VB6VC8VD1VTwo capacitrors of 2μFand4μF are connected in parallel. A third capacitor of 6μF is connected in series. The combaination is connected across a 12 V battery. The voltage across 2μF capacitor is
A2 VB8 VC6 VD1 V
Two capacitors C1 and C2 are connected to a battery of 6V as shown in Fig. Find the charge on each capacitor.
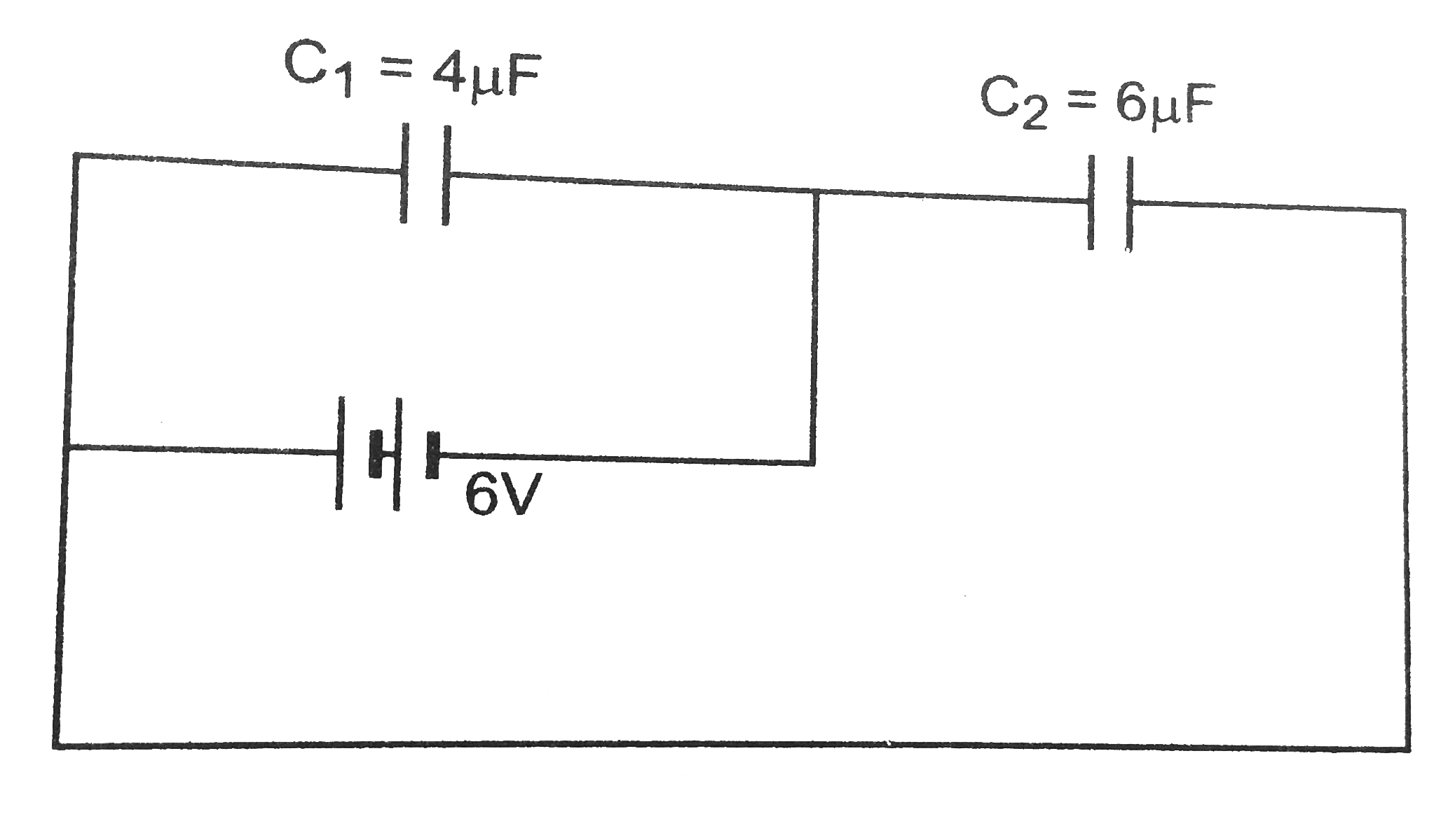
View SolutionThree capacitors having capanctances 20μF,30μF and 40μF are conneceted in series with a 12V battary. Find the charge on each of the capacitors. How much work has been done by the battery in charging the capacitors?
View SolutionFour capacitors of capacitances 2 μF and 1μF are connected across a battery of 10V as shown in the figure (q). Calculate the net capacitance of the combination and net charge across each capacitor.
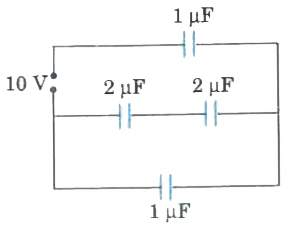
View SolutionTwo capacitors 2μFand4μF are connected in parallel. A third capacitor of 6μF capacity is connected in series. The combination is connected across a 12 V battery. The voltage across a 2μF capacitor is

View SolutionTwo capacitors, one of 4μF and the other of 5μF, are connected in parallel and then charged on a 100 V supply. The charge on each capacitor is respectively
View Solution