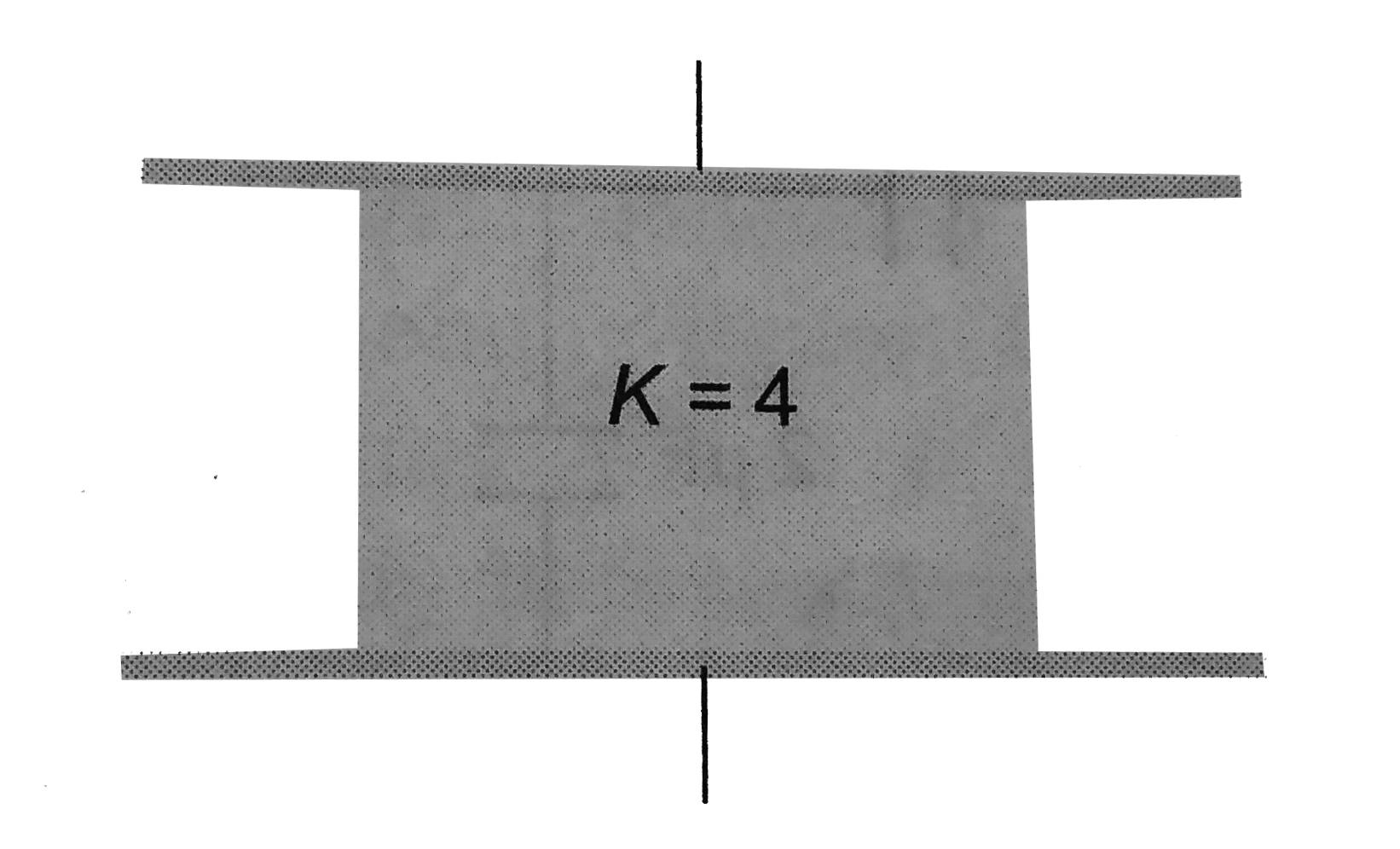
Consider a parallel plate capacitor of $10 μ F$ with air filled in the gap between the plates. Now one half of the space between the plates is filled with a dielectric of dielectric constant $4$ , as shown in the figure. The capacity of the capacitor charges to

A

$25 μ F$

B

$20 μ F$

C

$40 μ F$

D

$5 μ F$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

$C_{1} = \frac{ε_{0} (\frac{A}{4})}{d}, C_{2} = \frac{K ε_{0} (\frac{A}{2})}{d}, C_{3} = \frac{ε_{0} (\frac{A}{4})}{d}$
$C_{e q} = C_{1} + C_{2} + C_{3} = (\frac{K + 1}{2}) \frac{ε_{0} A}{d}$
$= (\frac{4 + 1}{2}) \times 10 = 25 μ F$

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Consider a parallel plate capacitor of $10 μ F$ with air filled in the gap between the plates. Now one half of the space between the plates is filled with a dielectric of dielectric constant $4$ , as shown in the figure. The capacity of the capacitor charges to

A $25 μ F$
B $20 μ F$
C $40 μ F$
D $5 μ F$
Consider a disconnected. Plate capacitor of capacity $10 μ$ with air filled in the gap between the plates. Now one-half of the space between the plates is filled with a dielectric of dielectric constant $4$ as shown in .The capacity of the capacitor changes to
.
A $25 μ F$
B $20 μ F$
C $40 μ F$
D $5 μ F$
A parallel plate air capacitor has capacitance C. Half of space between the plates is filled with dielectric of dielectric constant K as shown in figure . The new capacitance is C . Then

A $C = C [\frac{K}{K + 1}]$
B $C' = C [\frac{2 K}{K + 1}]$
C $C' = \frac{2 C}{K + 1}$
D $C' = C [1 + \frac{K}{2}]$

A2Z-ELECTRIC POTENTIAL & CAPACITANCE-Grouping Of Capacitors

Home
Ask Doubt
Profile