A capacitor when filled with a dielectric K=3 has charge Q0, voltage V0 and field E0. If the dielectric is replaced with another one having K=9, the new values of charge, voltage and field will be respectively
3Q0,3V0,3E0
Q0,3V0,3E0
Q0,V03,3E0
Q0,V03,E03
The correct Answer is:D
To solve the problem step by step, we will analyze the effects of replacing the dielectric in the capacitor and derive the new values of charge, voltage, and electric field.
Step 1: Understand the initial conditions
- The initial dielectric constant K1=3.
- The initial charge on the capacitor Q0.
- The initial voltage across the capacitor V0.
- The initial electric field E0.
Step 2: Determine the capacitance with the initial dielectric
The capacitance C1 of a capacitor filled with a dielectric is given by:
C1=K1⋅C0
where C0 is the capacitance without the dielectric. Thus,
C1=3⋅C0
Step 3: Analyze the situation when the dielectric is replaced
When the dielectric is replaced with another one having K2=9, the new capacitance C2 becomes:
C2=K2⋅C0=9⋅C0
Step 4: Conservation of charge
Since the battery is disconnected, the charge on the capacitor remains constant. Therefore, the charge after replacing the dielectric is still:
Q2=Q0
Step 5: Relate charge, capacitance, and voltage
The relationship between charge, capacitance, and voltage is given by:
Q=C⋅V
For the initial conditions:
Q0=C1⋅V0
For the new conditions:
Q2=C2⋅V2
Since Q2=Q0, we have:
Q0=C2⋅V2
Step 6: Solve for the new voltage V2
Substituting C2 into the equation:
Q0=(9⋅C0)⋅V2
From the initial condition, we know Q0=(3⋅C0)⋅V0. Thus, we can equate:
(3⋅C0)⋅V0=(9⋅C0)⋅V2
Cancelling C0 (assuming it is not zero):
3⋅V0=9⋅V2
Solving for V2:
V2=39⋅V0=13V0
Step 7: Calculate the new electric field E2
The electric field E in a capacitor is related to the voltage and the distance d between the plates:
E=Vd
Thus, the new electric field E2 can be calculated as:
E2=V2d=13V0d=13E0
Summary of Results
- The new charge Q2=Q0
- The new voltage V2=13V0
- The new electric field E2=13E0
Final Answer
The new values of charge, voltage, and electric field are:
- Charge: Q0
- Voltage: 13V0
- Electric Field: 13E0
- The initial dielectric constant K1=3.
- The initial charge on the capacitor Q0.
- The initial voltage across the capacitor V0.
- The initial electric field E0.
The capacitance C1 of a capacitor filled with a dielectric is given by:
C1=K1⋅C0
where C0 is the capacitance without the dielectric. Thus,
C1=3⋅C0
When the dielectric is replaced with another one having K2=9, the new capacitance C2 becomes:
C2=K2⋅C0=9⋅C0
Since the battery is disconnected, the charge on the capacitor remains constant. Therefore, the charge after replacing the dielectric is still:
Q2=Q0
The relationship between charge, capacitance, and voltage is given by:
Q=C⋅V
For the initial conditions:
Q0=C1⋅V0
For the new conditions:
Q2=C2⋅V2
Since Q2=Q0, we have:
Q0=C2⋅V2
Substituting C2 into the equation:
Q0=(9⋅C0)⋅V2
From the initial condition, we know Q0=(3⋅C0)⋅V0. Thus, we can equate:
(3⋅C0)⋅V0=(9⋅C0)⋅V2
Cancelling C0 (assuming it is not zero):
3⋅V0=9⋅V2
Solving for V2:
V2=39⋅V0=13V0
The electric field E in a capacitor is related to the voltage and the distance d between the plates:
E=Vd
Thus, the new electric field E2 can be calculated as:
E2=V2d=13V0d=13E0
- The new charge Q2=Q0
- The new voltage V2=13V0
- The new electric field E2=13E0
The new values of charge, voltage, and electric field are:
- Charge: Q0
- Voltage: 13V0
- Electric Field: 13E0
Topper's Solved these Questions
- ELECTRIC POTENTIAL & CAPACITANCEBOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Grouping Of Capacitors
48 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Capacitor Circuits
52 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Capacitor
32 Videos
- ELECTRIC CHARGE, FIELD & FLUXBOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Section D - Chapter End Test
29 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - A2ZCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
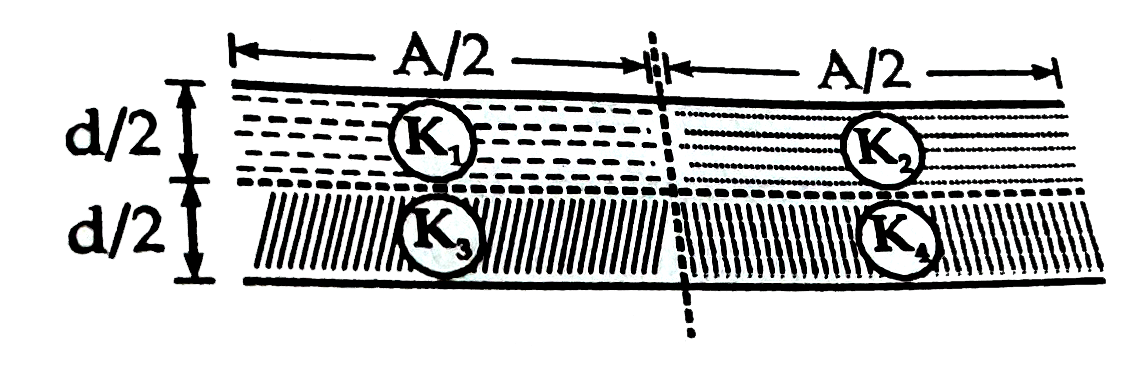
The space between parallel plate capacitors is filled with four dielectrics of equal dimensions but of dielectric constants K1,K2,K3andK4 respectively. If K is the dielectric constant of a single dielectric that must be filled between capacitor plates to have the same capacitance between A and B. Then we must have
View SolutionA parallel- plate capacitor, fileld with a dielectric of dielectric consatnt k, is charged to a potential V0. It is now disconnected from the cell and the slab is removed. If it now discharges, with time constant τ, through a resistance then find time after which the potential difference across it will be V0 ?
View Solution
Knowledge Check
A parallel plate capacitor filled with a material of dielectric constant K is charged to a certain voltage and is isolated. The dielectric material is removed. Then
(a) The capacitance decreases by a factor K
(b) The electric field reduces by a factor K
(c ) The voltage across the capacitor increases by a factor K
(d) The charge strored in the capacitor increases by a factor KAa and b are trueBa and c are trueCb and c are trueDb and d are trueA parallel plate capacitor filled with a material of dielectric constant K is charged to a certain voltage and is isolated. The dielectric material is removed. Then
(a) The capacitance decreases by a factor K
(b) The electric field reduces by a factor K
( c) The voltage across the capacitor increases by a factor K
(d) The charge stored in the capacitor increases by a factor K.Aa and b are trueBa and c are trueCb and c are trueDb and d are trueA parallel plate capacitor with a dielectric slab with dielectric constant k=3 filling the space between the plates is charged to potential V and isolated. Then the dielectric slab is drawn out and another dielectric slab of equal thickness but dielectric constant k'=2 is introduced between the plates. The ratio of the energy stored in the capacitor later to that initially is:
A2:3B3:2C4:9D9:4
A parallel plate capacitor having a separation between the plates d, plate area A and material with dielectric constant K has capacitance C0. Now one-third of the material is replaced by another material with dielectric constant 2K, so that effectively there are two capacitors one with area 1/3A, dielectric constant 2K and another with area 2/3A and dielectric constant K. If the capacitance of this new capacitor is C then CC0is
View SolutionA capacitor has some dielectric between its plates, and the capacitor is connected to a DC source. The battery is now disconnected and then the dielectric is removed. State whether the capacitance, the energy stored in it, electric field, charge stored and the voltage will increase ro remain constant.
View Solutionparallel plate capacitor is filled by a dielectric whose dielectric constant varies with the applies voltage (V) as K = 2V. A similar capacitor with no dielectric is charged to V0=78 volt.It is then connected to the uncharged capacitor with the dielectric.Find the final voltage on the capacitors
View SolutionA parallel plate capacitor A is filled with a dielectric whose dielectric constant varies with applied voltage as K = V. An identical capacitor B of capacitance C0 with air as dielectric is connected to voltage source V0=30V and then connected to the first capacitor after disconnecting the voltage source. The charge and voltage on capacitor.
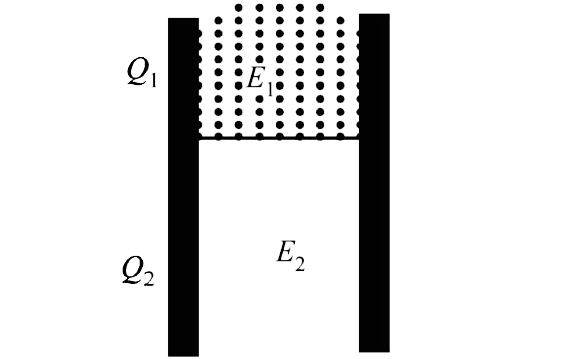
View SolutionA parallel plate capacitor has a dielectric slab of dielectric constant K between its plates that covers 1/3 of the area of its plates, as shown in the figure. The total capacitance of the capacitor is C while that of the portion with dielectric in between is C1. When the capacitor is charged, the plate area covered by the dielectric gets charge Q1 and the rest of the area gets charge Q2. The electric field in the dielectric is E1 and that in the other portion is E2. Choose the correct option/options, ignoring edge effects.
View Solution