Four idenbtial charges +50μC each are placed, one at each corner of a square of side 2m. How much external energy is required to bring another charge of +50μC from infinity to the centre of the square
(Given 14πε0=9×109Nm2C2)
64J
41J
16J
10J
The correct Answer is:A
To solve the problem of how much external energy is required to bring a charge of +50μC from infinity to the center of a square formed by four identical charges of +50μC each, we can follow these steps:
Step 1: Understand the Configuration
We have four charges, each of +50μC, placed at the corners of a square with a side length of 2m. We need to find the potential energy when a fifth charge of +50μC is brought to the center of the square.
Step 2: Calculate the Distance from the Center to a Corner
The distance from the center of the square to any corner can be calculated using the Pythagorean theorem. The center of the square divides the diagonal into two equal parts. The length of the diagonal d of the square is given by:
d=√(22+22)=√8=2√2m
Thus, the distance r from the center to a corner is:
r=d2=2√22=√2m
Step 3: Calculate the Electric Potential at the Center
The electric potential V at the center due to one charge is given by:
V=k⋅qr
where k=14πϵ0=9×109Nm2/C2 and q=50×10−6C.
For four charges, the total potential Vtotal at the center is:
Vtotal=4⋅k⋅qr=4⋅9×109⋅50×10−6√2
Step 4: Calculate the Potential Energy of the Fifth Charge
The potential energy U when bringing the fifth charge q′=50μC to the center is given by:
U=q′⋅Vtotal
Substituting q′:
U=50×10−6⋅4⋅9×109⋅50×10−6√2
Step 5: Simplify the Expression
Calculating the above expression:
U=50×10−6⋅4⋅9×109⋅2500×10−12√2
=50×10−6⋅4⋅22.5×10−3√2
=50×10−6⋅90×10−3⋅1√2
=4500×10−9√2≈3183.1×10−9J≈3.18J
Step 6: Final Calculation
Upon calculating, we find that the external energy required to bring the charge from infinity to the center of the square is approximately:
U≈64J
Final Answer
The external energy required is 64J.
We have four charges, each of +50μC, placed at the corners of a square with a side length of 2m. We need to find the potential energy when a fifth charge of +50μC is brought to the center of the square.
The distance from the center of the square to any corner can be calculated using the Pythagorean theorem. The center of the square divides the diagonal into two equal parts. The length of the diagonal d of the square is given by:
d=√(22+22)=√8=2√2m
Thus, the distance r from the center to a corner is:
r=d2=2√22=√2m
The electric potential V at the center due to one charge is given by:
V=k⋅qr
where k=14πϵ0=9×109Nm2/C2 and q=50×10−6C.
Vtotal=4⋅k⋅qr=4⋅9×109⋅50×10−6√2
The potential energy U when bringing the fifth charge q′=50μC to the center is given by:
U=q′⋅Vtotal
Substituting q′:
U=50×10−6⋅4⋅9×109⋅50×10−6√2
Calculating the above expression:
U=50×10−6⋅4⋅9×109⋅2500×10−12√2
=50×10−6⋅4⋅22.5×10−3√2
=50×10−6⋅90×10−3⋅1√2
=4500×10−9√2≈3183.1×10−9J≈3.18J
Upon calculating, we find that the external energy required to bring the charge from infinity to the center of the square is approximately:
U≈64J
The external energy required is 64J.
Topper's Solved these Questions
- ELECTRIC POTENTIAL & CAPACITANCEBook:A2ZChapter:ELECTRIC POTENTIAL & CAPACITANCEExercise:Capacitor
Explore 32 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBook:A2ZChapter:ELECTRIC POTENTIAL & CAPACITANCEExercise:Capacitor With Dielectric
Explore 32 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBook:A2ZChapter:ELECTRIC POTENTIAL & CAPACITANCEExercise:Electric Potential Energy
Explore 22 Videos
- ELECTRIC CHARGE, FIELD & FLUXBook:A2ZChapter:ELECTRIC CHARGE, FIELD & FLUXExercise:Section D - Chapter End Test
Explore 29 Videos
- ELECTROMAGNETIC INDUCTIONBook:A2ZChapter:ELECTROMAGNETIC INDUCTIONExercise:Section D - Chapter End Test
Explore 30 Videos
Similar Questions
Four point charges of +1μc each are placed on the four corners of a square of side 1 m. Calculate the electric potential at the centre of the square.
View SolutionFour equal point harges each 16μC are placed on the four corners of a square of side 0.2 m. Calculate the force on any one of the charges.
View Solution
Knowledge Check
Four equal charges Q are placed at the four corners of a square of each side is 'a'. Work done in removing a charge −Q from its centre to infinity is
AzeroB√2q2π∈0aC√2qπ∈0aDq2π∈0aCharge of 5μC each are placed at the corners of an equilateral triangle of side 10 cm. Then the force on each charge is :-
A78 NB39 NC29 ND22.5 NFor charges A,B,C and D are plasced at the four corners of a squre of a side a. The energy required to take the charges C and D to infinity (they are also infinitely separated from each other )is
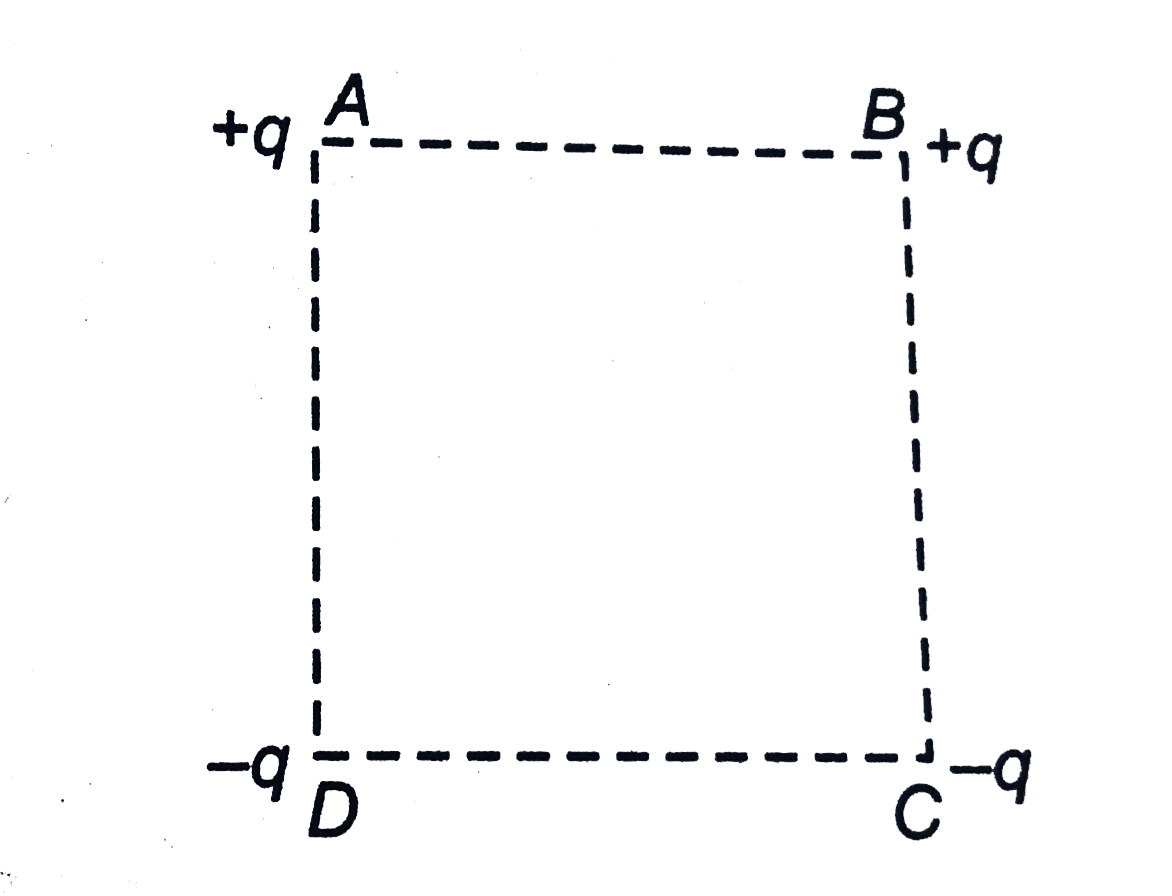 Aq24πε0aB2q2πε0aCq24πε0a(√2+1)Dq24πε0a(√2−1)
Aq24πε0aB2q2πε0aCq24πε0a(√2+1)Dq24πε0a(√2−1)
Four charges are placed at the corners of a square of side 10 cm as shown in figure. If q is 1μC, then what will be electric field intensity at the centre of the square?

View SolutionThe electric field due to a charge at a distance of 3 m from it is 500NC−1. The magnitude of the charge is
[14πε0=9×109N−m2/C2]
View SolutionThree charges ,each of +4μC ,are placed at the corners A,B,C of a square ABCD of side 1m.The electric field at the centre O of a square ABCD of side 1m.The electric field at the centre O of the square is
View SolutionFour charges of 1mC,2mC,3mC and −6mC are placed one at each corner of the square of side 1m.The square lies in the x−y plane with its centre at the origin
View SolutionThree particles, each having a charge of 10μC are placed at the coners of an equilateral triangle of side 10cm. The electrostatic potential energy of the system is (Given 14πε0=9×109N−m2/C2)
View Solution
