In the figure, O is the centre of the circle and B is a point of contact. Seg OE seg AD, AB = 12, AC = 8, find (i) AD (ii) DC (iii) DE.
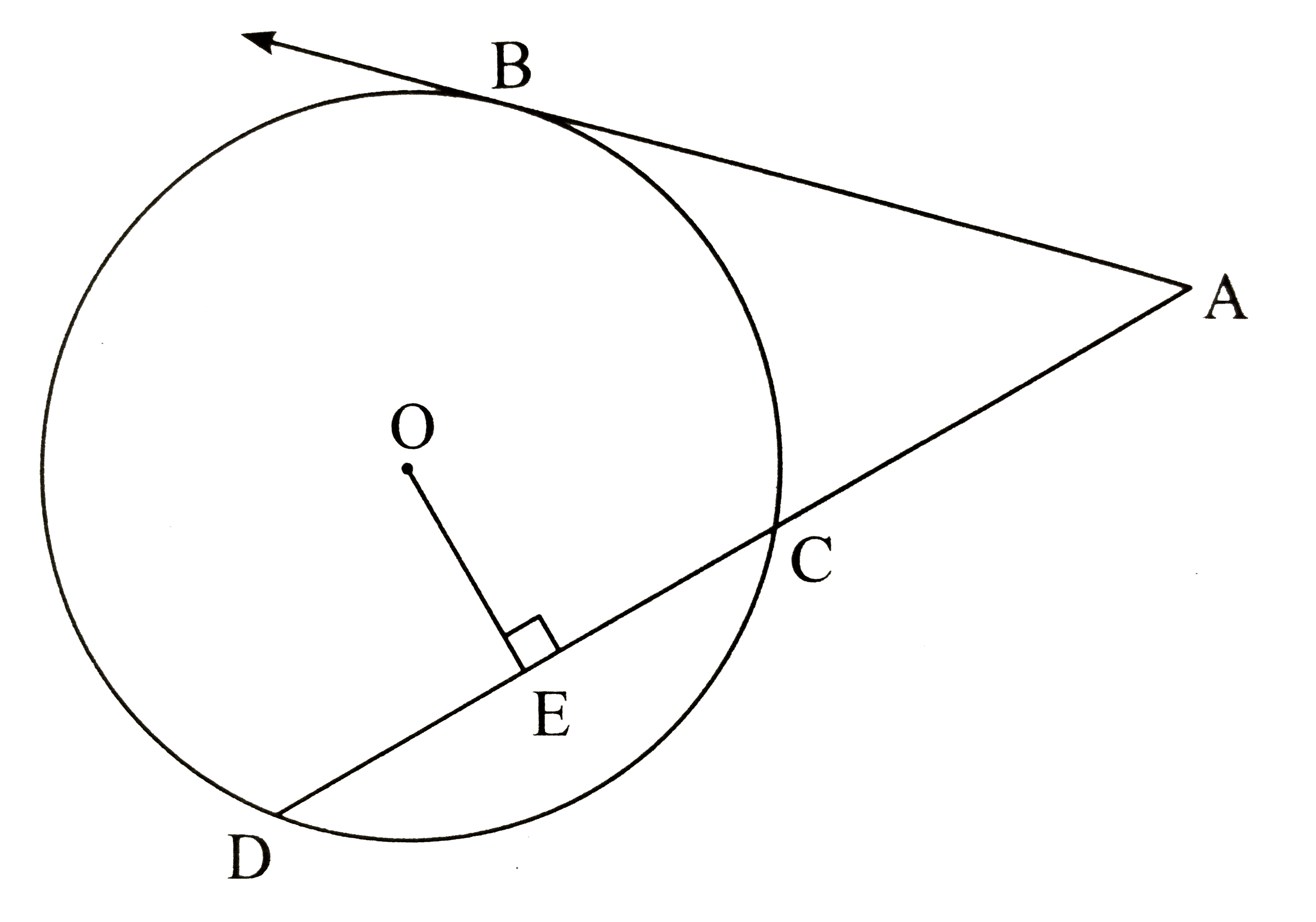
The correct Answer is:(1) 18, (2) 10, (3) 5
Ray AB is tangent to the circle at point B. Line ACD us secant intersecting the circle at points C and D.
by tangent secant segments theorem,
seg OE chord CD
Perpendicular drawn from the centre of the circle to the chord bisects the chord.
Topper's Solved these Questions
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - PROBLEM SET 3
50 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - Challenging Questions
10 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - PRACTICE SET 3.4
15 Videos
- CHALLENGING QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE ) Solve any one of the following subquestions :
1 Videos
- COORDINATE GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - COORDINATE GEOMETRY EXERCISE - Assignment 6.5
13 Videos
Similar Questions
In the figure above, O is the centre of the circle CD is a tangent to the circle at E. OE bisects the chord AB at F. AB = 16 cm and EF = 2 cm. Find the length of DE (in cm).
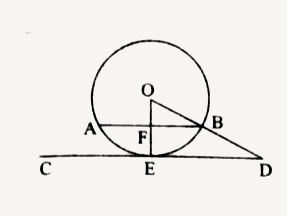
View Solution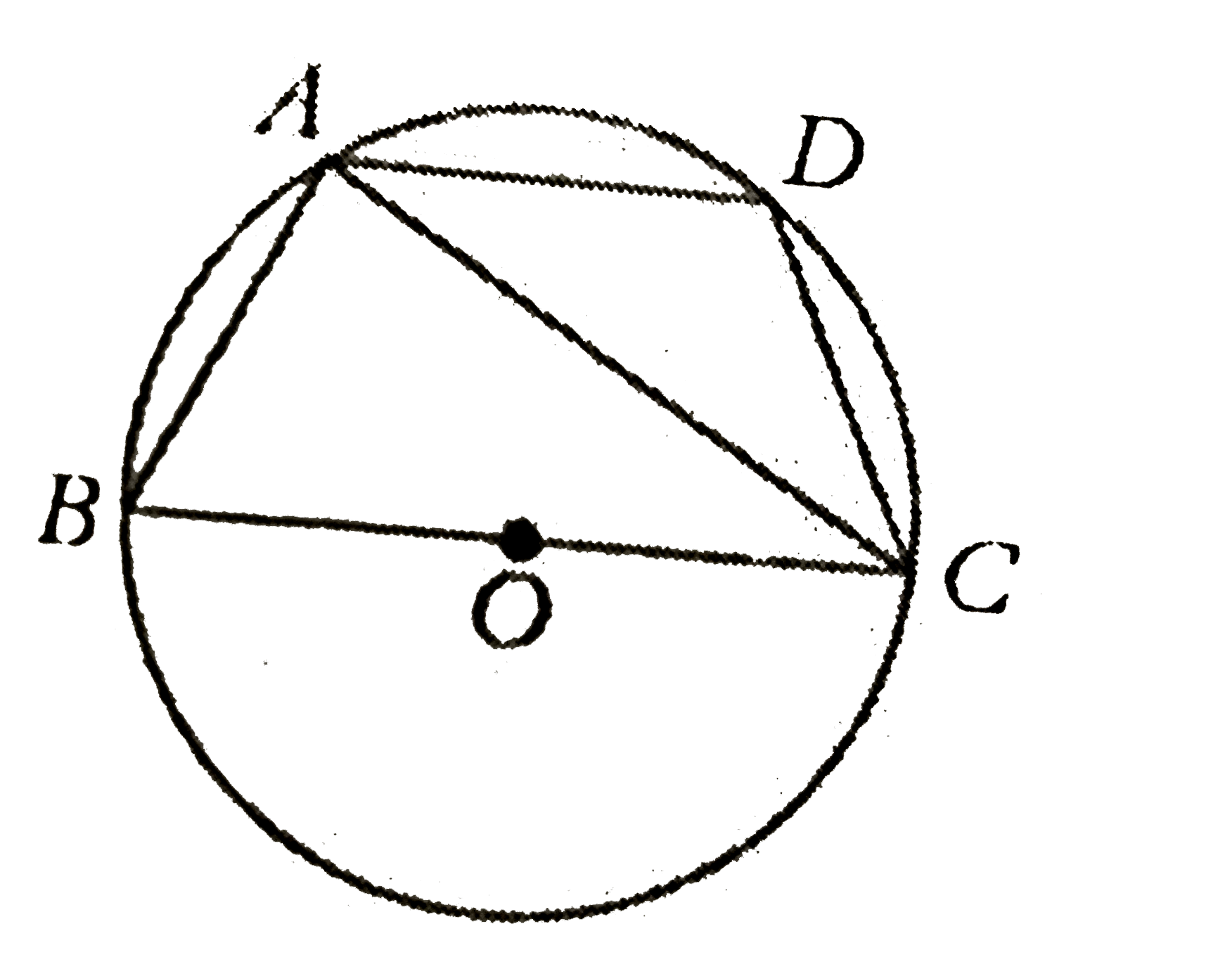
In the above figure, O is the centre of the circle AB,AD and CD are the chords . If ∠ADC=130∘ then fid ∠ACB.
View Solution
Knowledge Check
In the given figure, O is the centre of the circle. Given that OD = OE = 3 cm and AD = 4 cm. Find the length of the longest chord.
 A6 cmB8 cmC10 cmD9 cm
A6 cmB8 cmC10 cmD9 cm
In the adjoining figure, M is the centre of the circle and seg AB is a diameter. Seg MS⊥ chord AD, seg MT ⊥ chord AC, ∠DAB≅∠CAB.
Prove that : chord AD≅ chord AC.
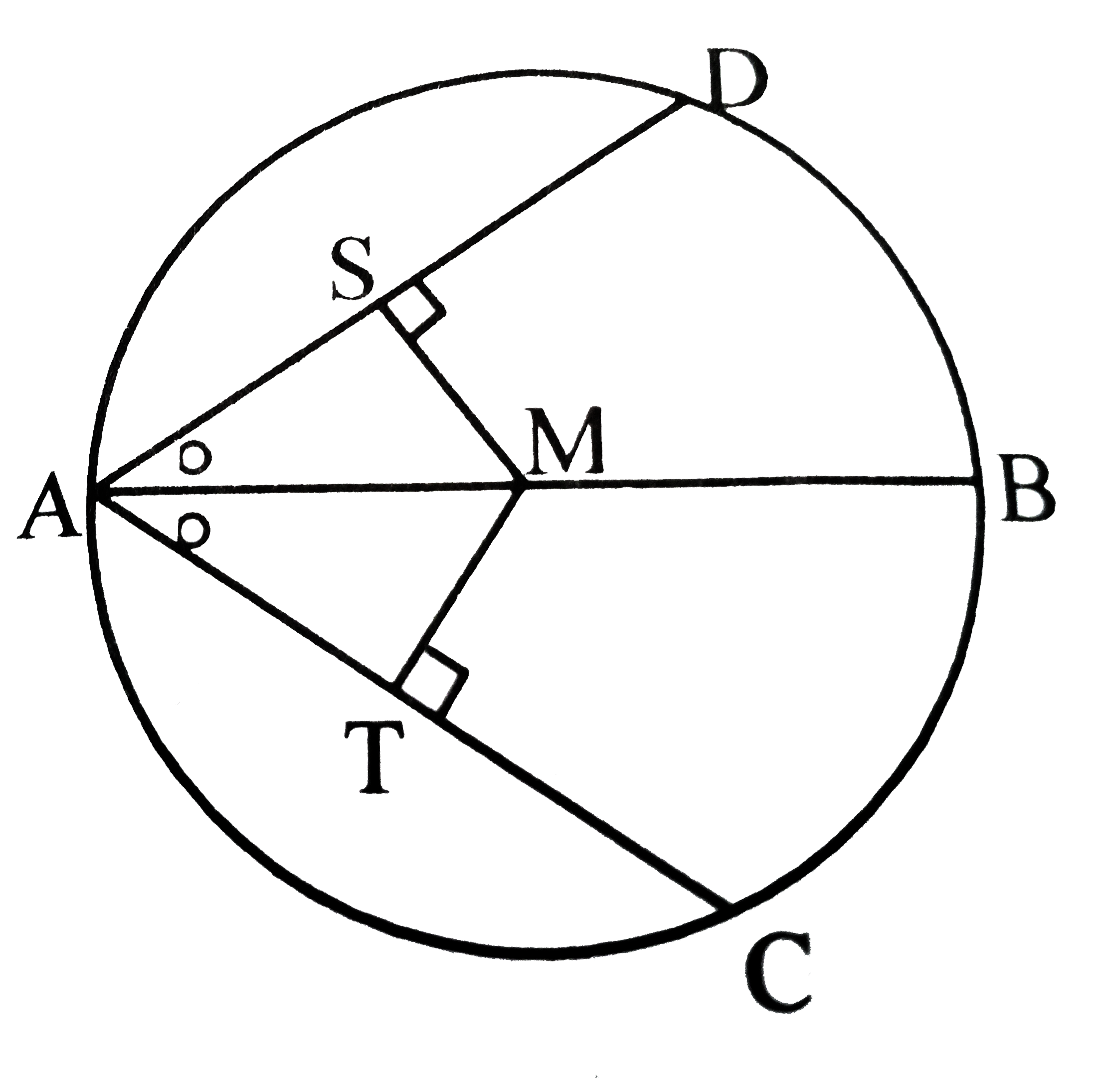
View SolutionIn the figure, O is the centre of the circle . Seg AB is the diameter at the point C on the circle the tangent CD is drawn. Line BD is a tangent to the circle at point B. Show that seg OD ∣∣ chord AC.
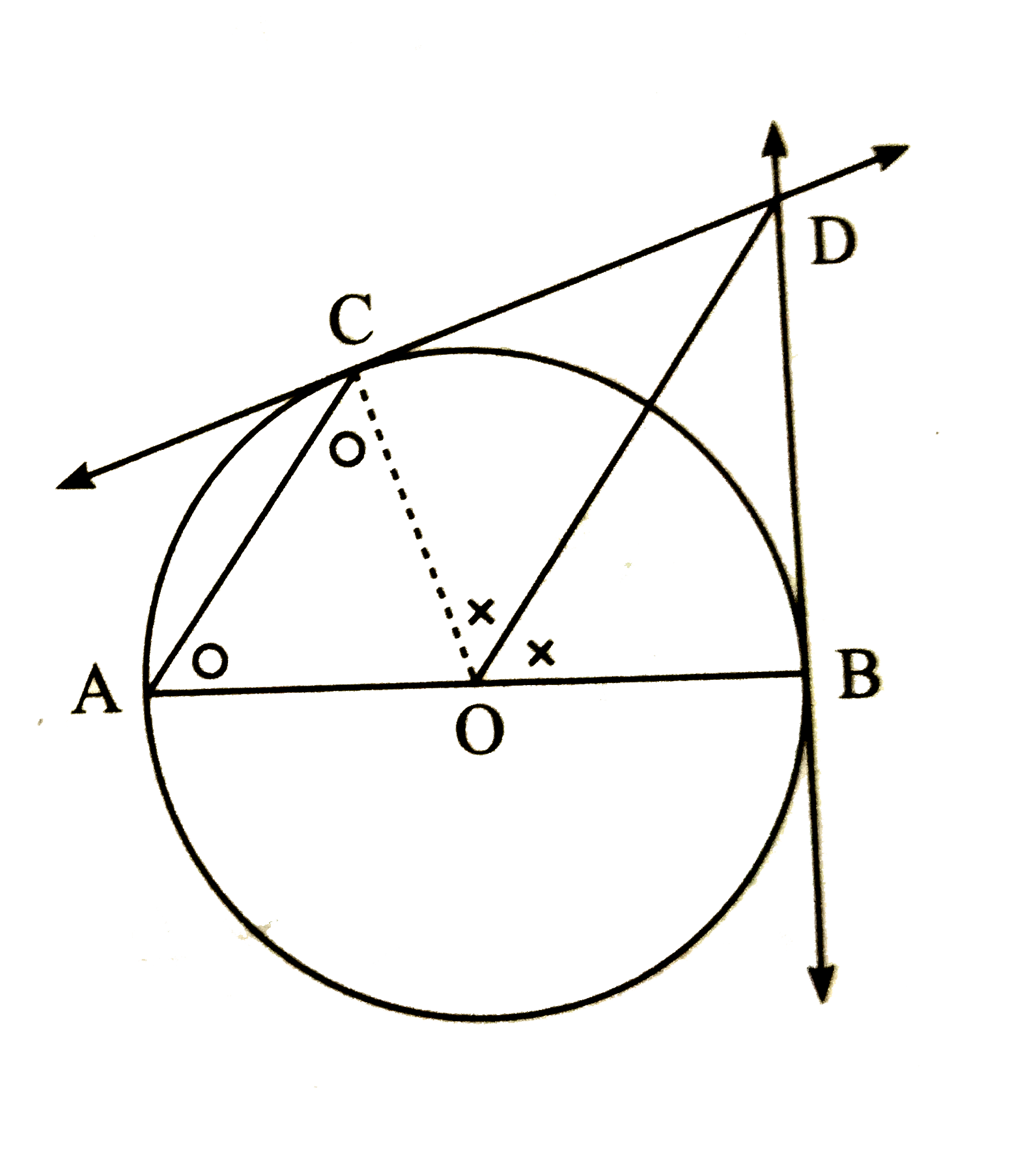
View SolutionSolve any two of the following subquestions :
In the figure , O is the centre of the circles and AP is the tangent to the circle at point A . Ray AF is the bisector of ∠BAC
Prove : seg AP≅ seg PE<br>
View SolutionIn the figure, O is the centre of the circle of the circle. Seg AB, seg AC are tangent segments. Radius of the circle is r and l(AB)=r. Prove that, □ABOC is a square.
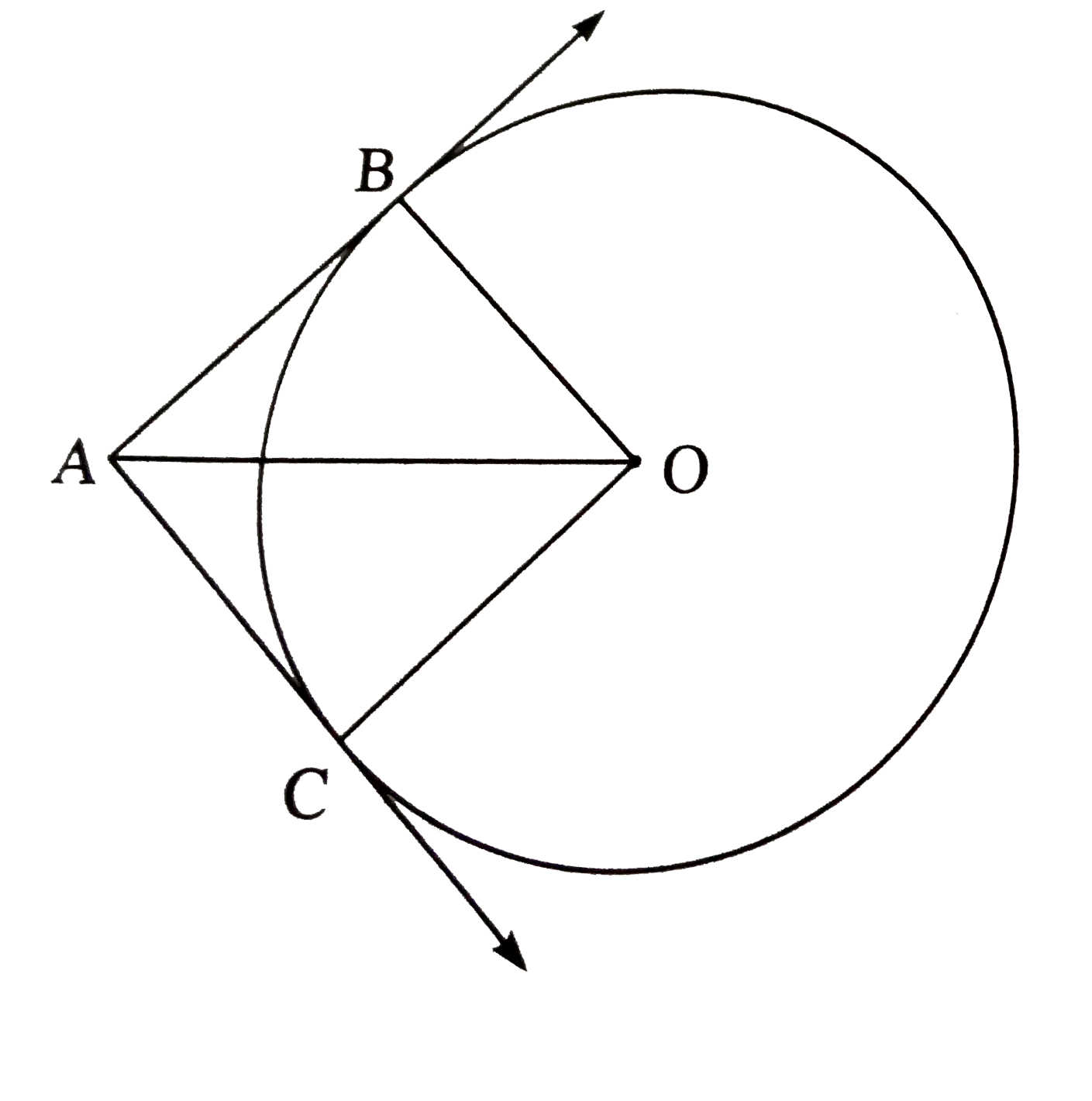
View Solution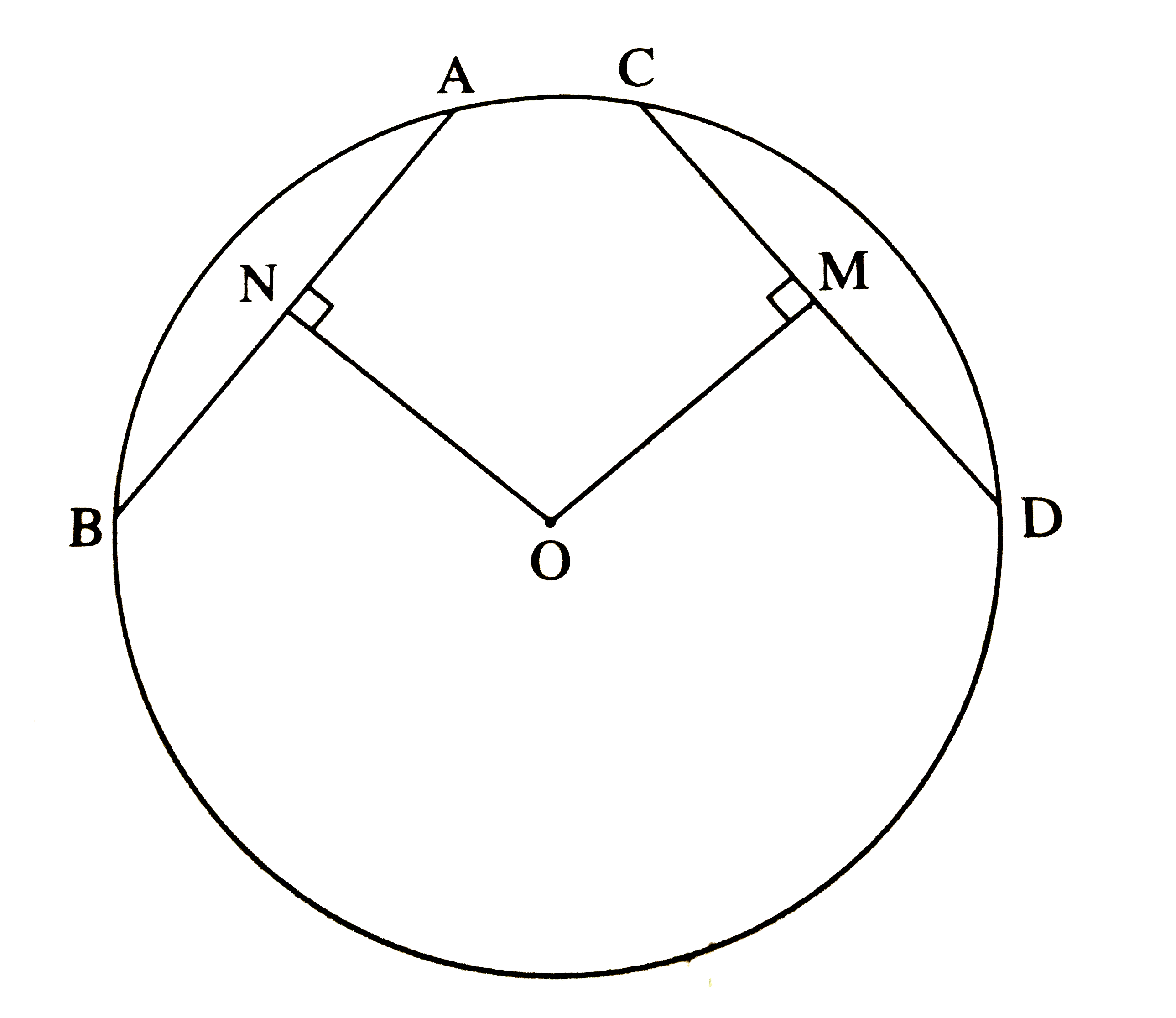
In the figure , O is the center of the circle.chord AB and CD are congruent . If seg ON ⊥ chord AB and seg OM ⊥ chord CD and OM =4cm , then find ON . Justify your answer .
View SolutionIf in the figure AB=a,AC=4cm, while D is the circle of the circle and D is a points betbeen O and B such that AD⊥BC.The length (in cm) of OD is
View SolutionIn the figure D is a point on BC such that ∠ABD=∠CAD. If AB=5cm,AD=4cm and AC=3cm. Find (i) BC (ii) DC (iii) A(ΔACD):AIΔBCA).
View Solution
