निम्नलिखित में A, B, C, D, E, R तथा R को पहचानिये-
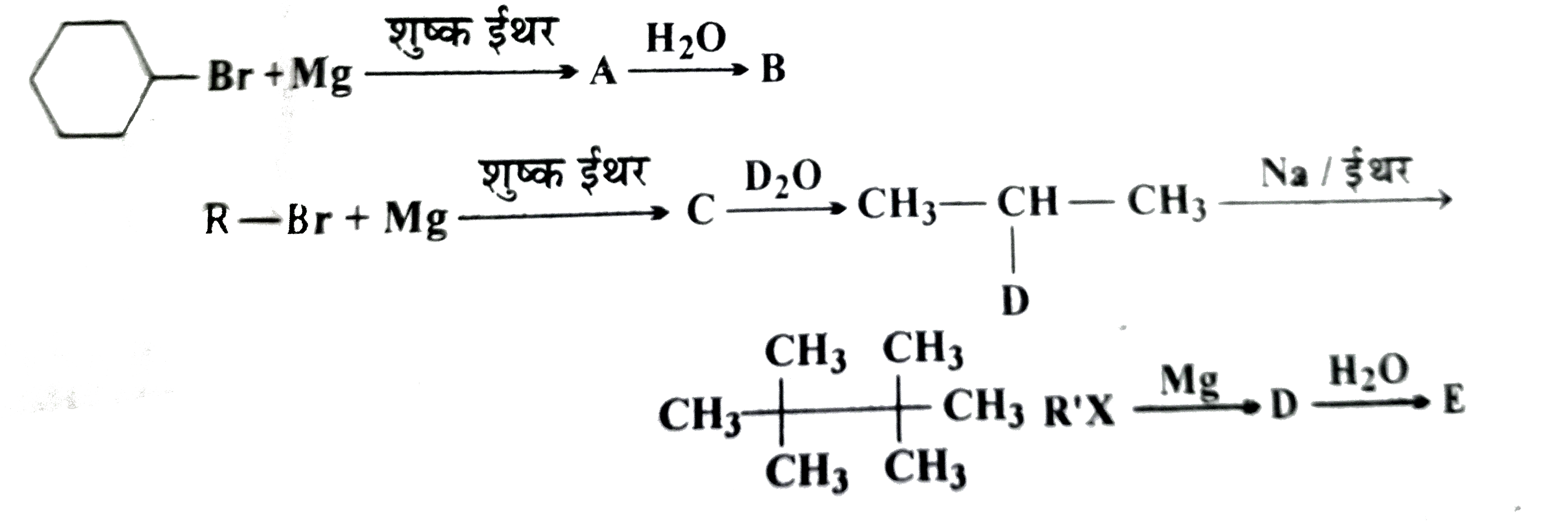
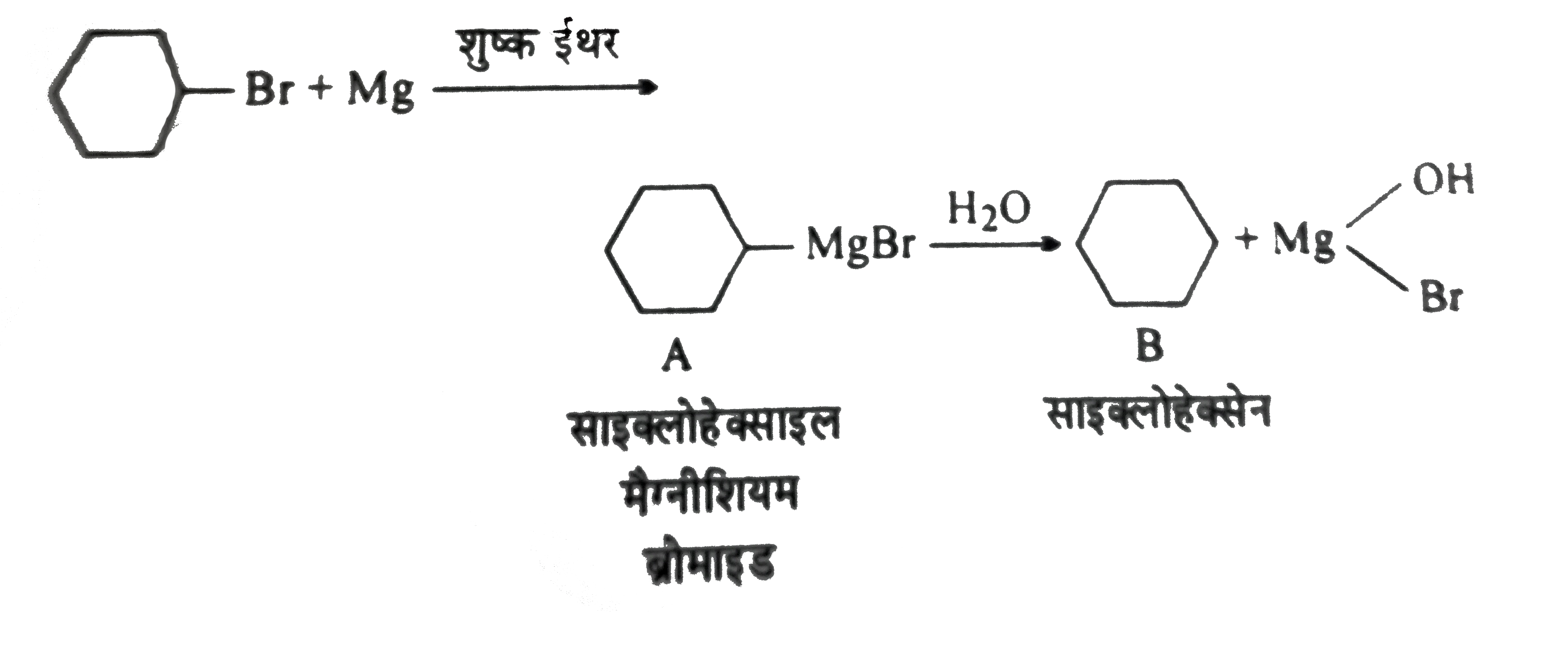
R−Br+Mgशुष्क ईथर −−−−−−→RMgBrCD2O−−→CH3−CH∣D−CH3
चूँकि D उस C परमाणु जिस पर MgBr या Br उपस्थित होगा है, से जुड़ा होता है, अतः
R−Br=CH3−CH∣Br−CH3 या R=CH3−CH−CH3∣MgBrआइसोप्रोपाइल
Topper's Solved these Questions
- हैलोएल्केन्स तथा हैलोएरिन्स BOOK - NAVBODHCHAPTER - हैलोएल्केन्स तथा हैलोएरिन्स EXERCISE - NCERT पाठ्य-पुस्तक प्रश्नोत्तर
89 Videos
- हैलोएल्केन्स तथा हैलोएरिन्स BOOK - NAVBODHCHAPTER - हैलोएल्केन्स तथा हैलोएरिन्स EXERCISE - अन्य महत्वपूर्ण प्रश्नोत्तर (लघु उत्तरीय प्रश्न)
28 Videos
- हैलोएल्केन्स तथा हैलोएरिन्स BOOK - NAVBODHCHAPTER - हैलोएल्केन्स तथा हैलोएरिन्स EXERCISE - एक शब्द/वाक्य में उत्तर दीजिए-
8 Videos
- सतह रसायन BOOK - NAVBODHCHAPTER - सतह रसायन EXERCISE - दीर्घ उत्तरीय प्रश्न
8 Videos
Similar Questions
निम्नलिखित हेलाइडो के नाम आई यु पी ए सी पद्धति से लिखिए तथा उनका वर्गीकरण ऐल्किल ऐलाइलिक बेन्जाइलिक (प्राथमिक, द्वितीयक एवं तृतीयक) वाइनिल अथवा ऐरिल हैलाइड के रूप में कीजिए-
(CH3)2CHCH(Cl)CH3
View Solutionआयोडोफॉर्म अभिक्रिया लिखिए।
View Solutionबेंजीन में मैथिल क्लोराइड के साथ निर्जल AlCl3 की उपस्थित में क्रिया करने पर टालूइन बनता है। इस अभिक्रिया का नाम क्या है ?
View Solutionनिम्नलिखित पर संक्षिप्त टिप्पणियां लिखिए-
(i) ब्राउनी गति, (ii) स्व-उत्प्रेरण।
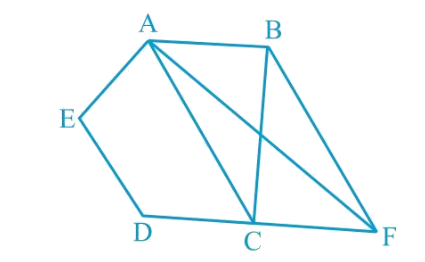
View SolutionIn Figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that ar(ACB)=ar(ACF) (ii) ar(AEDF)=ar(ABCDE)
View SolutionIn Figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that: ar (ACB)=ar (ACF) ar (AEDF)=ar (ABCDE)
View SolutionAny point D is taken in the base BC of a triangle ABC and AD is produced to E, making DE equal to AD. Show that ar (BCE)=ar(ABC)
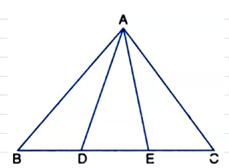
View SolutionIn Figure DandE are two points on BC such that BD=DE=EC Show that ar(ABD)=ar(ADE)=ar(AEC)
View SolutionIdentity A, B, C, D, E, R and R' in the following:
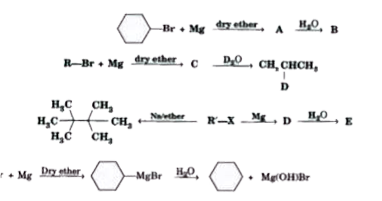
View SolutionIdentity A, B, C, D, E, R and R' in the following:
View SolutionIdentify A, B, C, D, E, R and R1 in the following :

View SolutionIn Figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that: (i) ar(△ACB)=ar(△ACF) (ii) ar(quadrilateralAEDF)=ar(ABCDE)
View SolutionIn triangle ABC , if AD is the bisector of ∠A , prove that: Area(ABD)Area(ACD)=ABAC
View SolutionIn Figure, △ABC and △DBC are on the same base BC . If AD and BC intersect at O , prove that Area (ABC)Area (DBC)=AODO.
View SolutionIn ABC , AD and BE are altitudes. Prove that ar(DEC)ar(ABC)=DC2AC2
View SolutionIf ABC and DEF are two triangles such that ABDE=BCEF=CAFD=25 , then Area(ABC):Area(DEF)=
View Solution
