TP and TQ are any two tangents to a parabola and the tangent at a third point R cuts them in P' and Q'. Prove that TP'TP+TQ'TQ=1
The correct Answer is:∴TP'TP+TQ'TQ=1.
Step by step video, text & image solution for TP and TQ are any two tangents to a parabola and the tangent at a third point R cuts them in P' and Q'. Prove that (TP')/(TP)+(TQ')/(TQ)=1 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PARABOLABOOK - ARIHANT MATHSCHAPTER - PARABOLAEXERCISE - Exercise For Session 1
20 Videos
- PARABOLABOOK - ARIHANT MATHSCHAPTER - PARABOLAEXERCISE - Exercise For Session 2
25 Videos
- PAIR OF STRAIGHT LINES BOOK - ARIHANT MATHSCHAPTER - PAIR OF STRAIGHT LINES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
28 Videos
Similar Questions
Knowledge Check
TP and TQ are the tangents to a circle, with centre O. Find x.
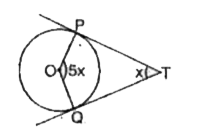 A15∘B60∘C30∘D45∘
A15∘B60∘C30∘D45∘If TP and TQ are two tangents to a circle with centre O, so that ∠POQ=120∘, then ∠PTQ is equal to
A120∘B30∘C60∘D90∘TP and TQ are tangents from T to the circle with centre O. Then is it possible to draw a circle through the points P, O, Q and T ?
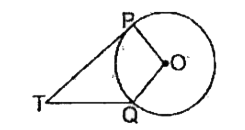 ANoBYesCCannot sayDData insufficient
ANoBYesCCannot sayDData insufficient
Point of intersection of tangent at any Two points on the Parabola
View SolutionIf TP and TQ are the two tangents to a circle with centre O so that ∠POQ=110∘, then ∠PTQ is equal to
View SolutionTangents are drawn from the point(-1, 2) to the parabola y2=4x. These tangents meet the line x=2 at P and Q, then length of PQ is
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T . Prove that ∠PTQ=2∠OPQ .
View SolutionTP&TQ are tangents to the parabola, y2=4ax at P and Q. If the chord passes through the point (−a,b) then the locus of T is
View Solution
