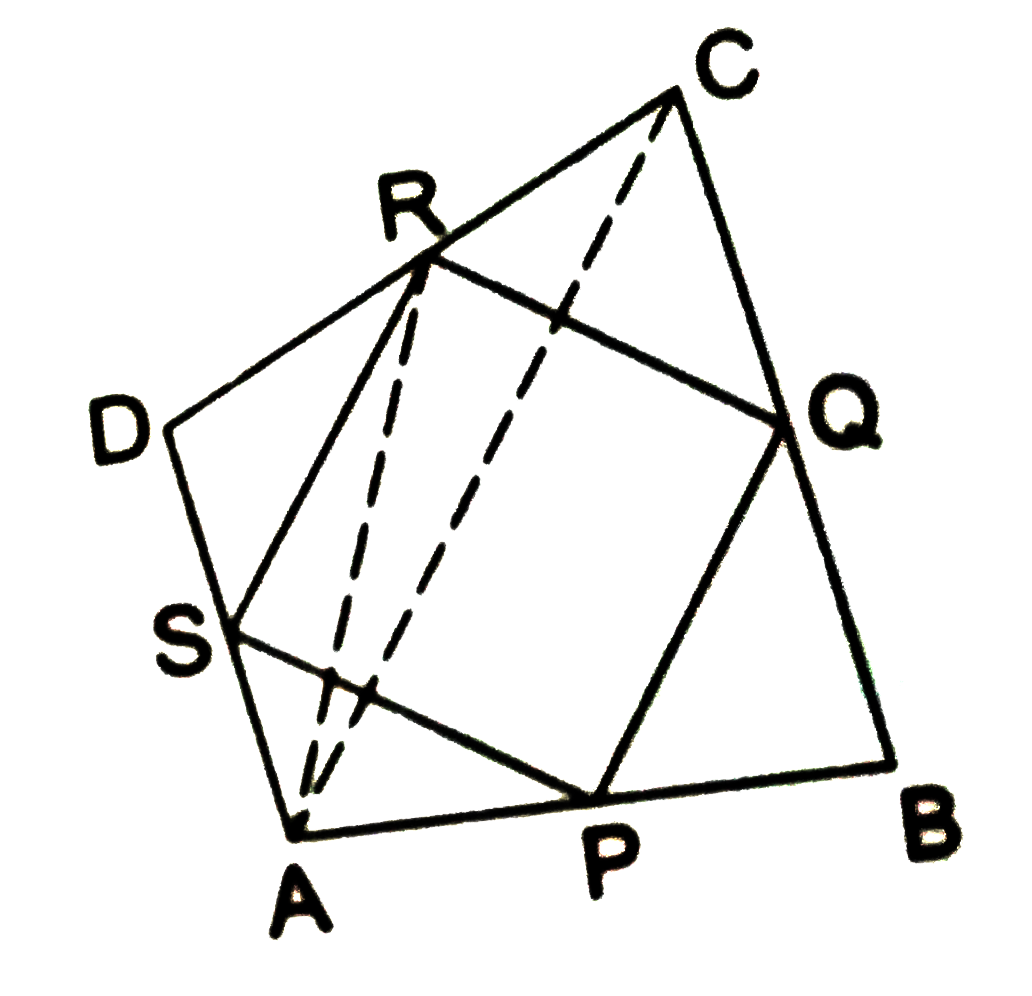
If P, Q, R, S are four coplanar points on the sides AB, BC, CD, DA of a skew quadrilateral, then ABPB⋅BQQC⋅CRRD⋅DSSA equals
1
−1
3
−3
The correct Answer is:(a)
To solve the problem, we need to find the value of the expression:
ABPB⋅BQQC⋅CRRD⋅DSSA
where P,Q,R,S are coplanar points on the sides AB,BC,CD,DA of a skew quadrilateral ABCD.
1. Understanding the Ratios:
- Let P divide AB in the ratio α:1.
- Let Q divide BC in the ratio β:1.
- Let R divide CD in the ratio γ:1.
- Let S divide DA in the ratio δ:1.
2. Expressing the Coordinates:
- The coordinates of point P can be expressed as:
P=(αx2+x1α+1,αy2+y1α+1,αz2+z1α+1)
- Similarly, we can express the coordinates for points Q,R,S using their respective ratios.
3. Setting up the Plane Equation:
- Since P,Q,R,S are coplanar, they must satisfy the plane equation. The general form of the plane equation is:
ax+by+cz+d=0
- Substitute the coordinates of points P,Q,R,S into this equation to derive relationships between α,β,γ,δ.
4. Finding the Values of Ratios:
- After substituting, we will find:
α=−p1p2,β=−p2p3,γ=−p3p4,δ=−p4p1
- Here, p1,p2,p3,p4 are the determinants formed from the coordinates of points A,B,C,D.
5. Calculating the Product:
- Now, we multiply the ratios:
α⋅β⋅γ⋅δ=(−p1p2)⋅(−p2p3)⋅(−p3p4)⋅(−p4p1)
- This simplifies to:
p1p2p3p4p2p3p4p1=1
6. Conclusion:
- Therefore, the value of the expression is:
ABPB⋅BQQC⋅CRRD⋅DSSA=1
- Let P divide AB in the ratio α:1.
- Let Q divide BC in the ratio β:1.
- Let R divide CD in the ratio γ:1.
- Let S divide DA in the ratio δ:1.
- The coordinates of point P can be expressed as:
P=(αx2+x1α+1,αy2+y1α+1,αz2+z1α+1)
- Similarly, we can express the coordinates for points Q,R,S using their respective ratios.
- Since P,Q,R,S are coplanar, they must satisfy the plane equation. The general form of the plane equation is:
ax+by+cz+d=0
- Substitute the coordinates of points P,Q,R,S into this equation to derive relationships between α,β,γ,δ.
- After substituting, we will find:
α=−p1p2,β=−p2p3,γ=−p3p4,δ=−p4p1
- Here, p1,p2,p3,p4 are the determinants formed from the coordinates of points A,B,C,D.
- Now, we multiply the ratios:
α⋅β⋅γ⋅δ=(−p1p2)⋅(−p2p3)⋅(−p3p4)⋅(−p4p1)
- This simplifies to:
p1p2p3p4p2p3p4p1=1
- Therefore, the value of the expression is:
ABPB⋅BQQC⋅CRRD⋅DSSA=1
Topper's Solved these Questions
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Exercise (More Than One Correct Option Type Questions)
28 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Exercise (Statement I And Ii Type Questions)
14 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Exercise For Session 4
7 Videos
- THEORY OF EQUATIONSBOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
35 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
Similar Questions
The quadrilateral formed by joining the mid-points of the sides AB, BC, CD, DA of a quadrilateral ABCD is
View SolutionP, Q , R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.
View SolutionP, Q, R and S are respectively the mid-points of sides AB, BC, CD and DA of quadrilateral ABCD in which AC=BD and AC⊥BD. Prove that PQRS is a square.
View SolutionLet ABCD be a rectangle. Let P, Q, R, S be the mid-points of sides AB, BC, CD, DA respectively. Then the quadrilateral PQRS is a
View SolutionLet P, Q, R, S be respectively the midpoints of the sides AB, BC, CD and DA of quad. ABCD Show that PQRS is a parallelogram such that
ar(||gm PQRS)=12ar(quad. ABCD).
View SolutionABCD is a rhombus and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
View SolutionA circle touches all the four sides of a quadrilateral ABCD . Prove that: AB+CD=BC+DA .
View SolutionA circle touches all the four sides of a quadrilateral ABCD. Prove that AB + CD = BC + DA
View SolutionIf a,b∈N, PQRS is a square and P, Q, R, S are mid points of AB, AD, CD, BC respectively then a+b is
View SolutionABCD is a square. P, Q, R, S are points on the sides AB. BC, CD, DA respectively such that AP = BQ = CR = DS. What is ∠SPQ equal to ?
View Solution
