A large open tank has two holes in the wall. One is a square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then, R is equal to
L
The correct Answer is:B
Velocity of efflux when the hole is a t depth ,
Rate of flow of water from square hole
Rate of flow of water from circular hole
According to problem,
.
Topper's Solved these Questions
Similar Questions
A large open tank has two holes in the wall. One is a square hole of side L at a depth h from the top and the other is a circular hole of radius r at a depth 4h from the top. Whwn the tank is completely filled with water, the quantity of water flowing out per second from both the holes are the same. What is the value of r?
View SolutionA vessel completely filled with water has holes 'A' and 'B' at depths 'h' and '3h' from the top respectively. Hole 'A' is a square of side 'L' and 'B' is circle of radius 'r'. The water flowing out per second from both the holes is same. Then 'L' is equal to
View Solution
Knowledge Check
A large open tank has two holes in the wall. One is a square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then, R is equal to
AL√2πB2πLCL√2πDL2πA large open tank has two holes in its wall. Ine is a square hole of side a at a depath of x from the top and the other is a cirular hle of radius r at a depth 4x from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then r is equal to
A2πaBaCa2πDa√2πA large open tank has two holes in the wall. The top hole is a square hole of side L at a depth y from the surface of water. The bottom hole is a circular hole of radius R at a depth 4y from surface of water . If R=L/2√π, find the correct graph. V1 and V2 are the velocities in top and bottom holes. Area of the square & curcular holes are a1 and a2
A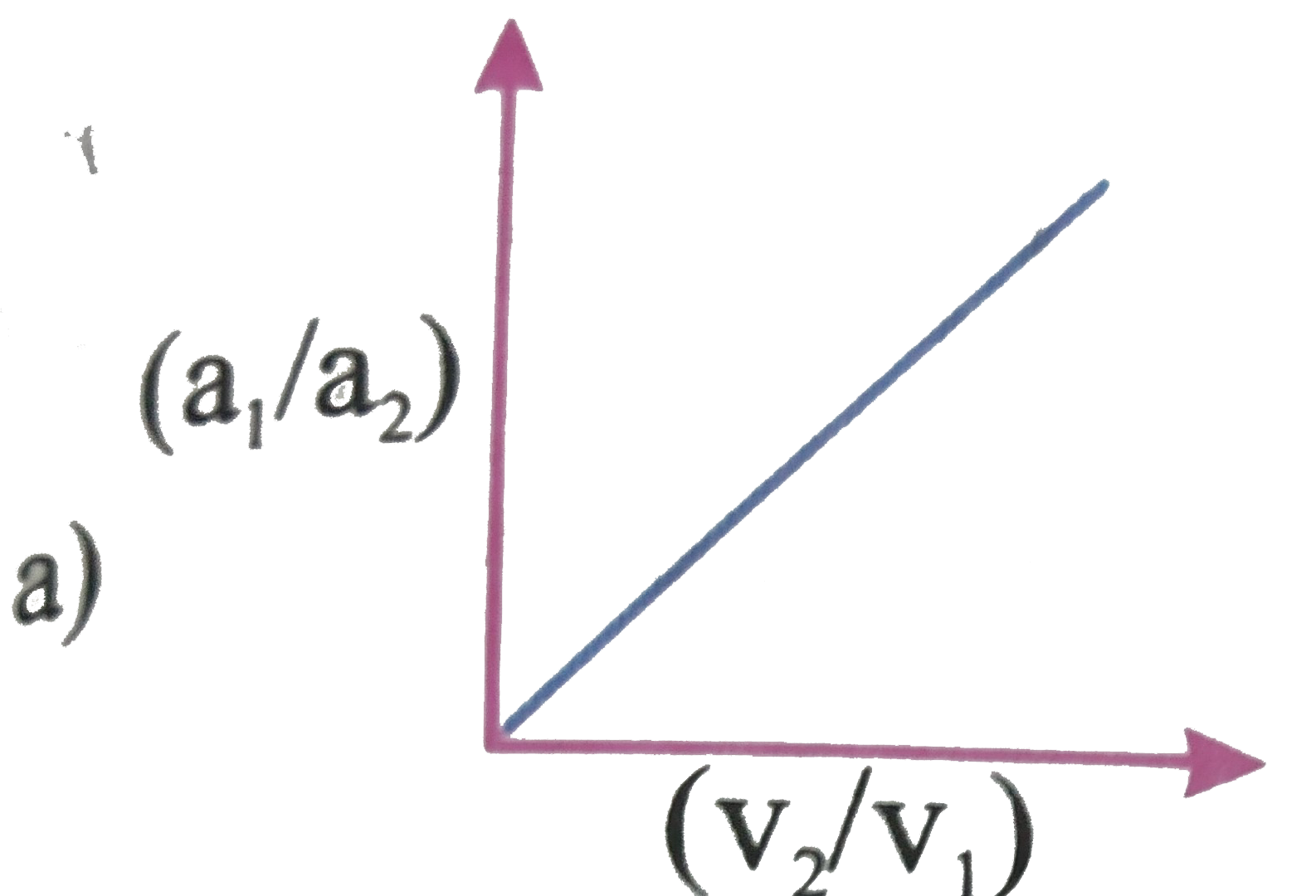 B
B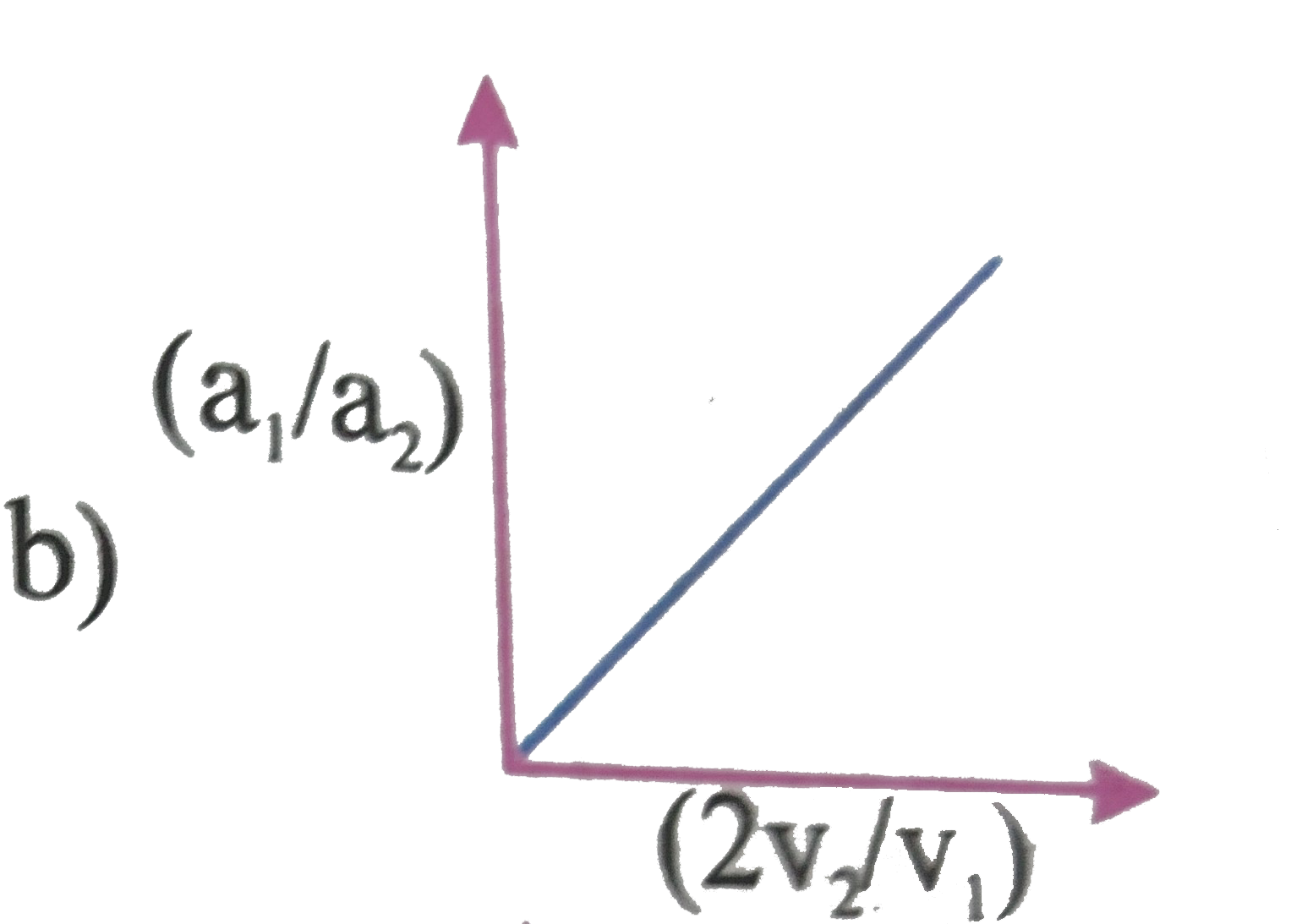 C
C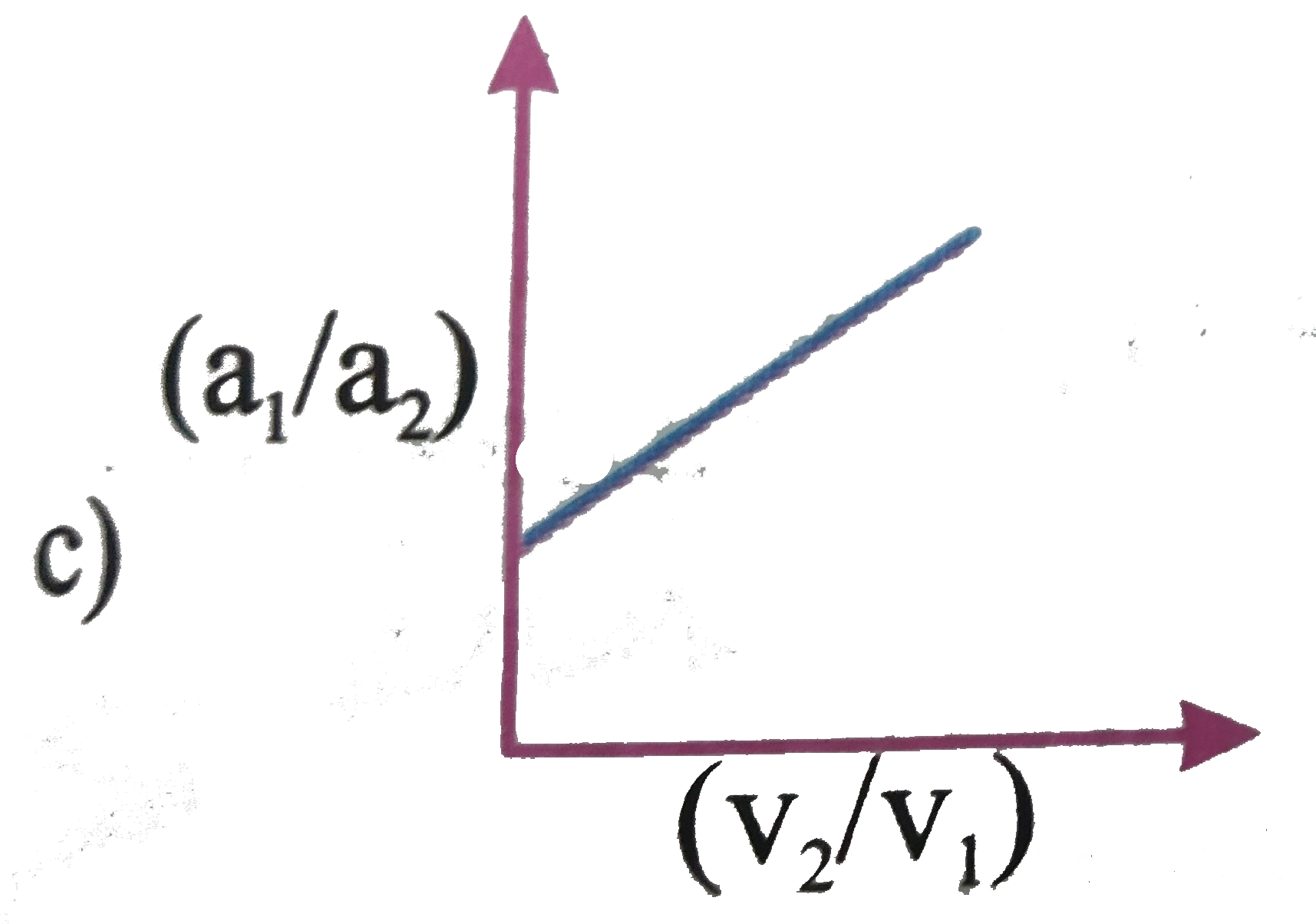 D
D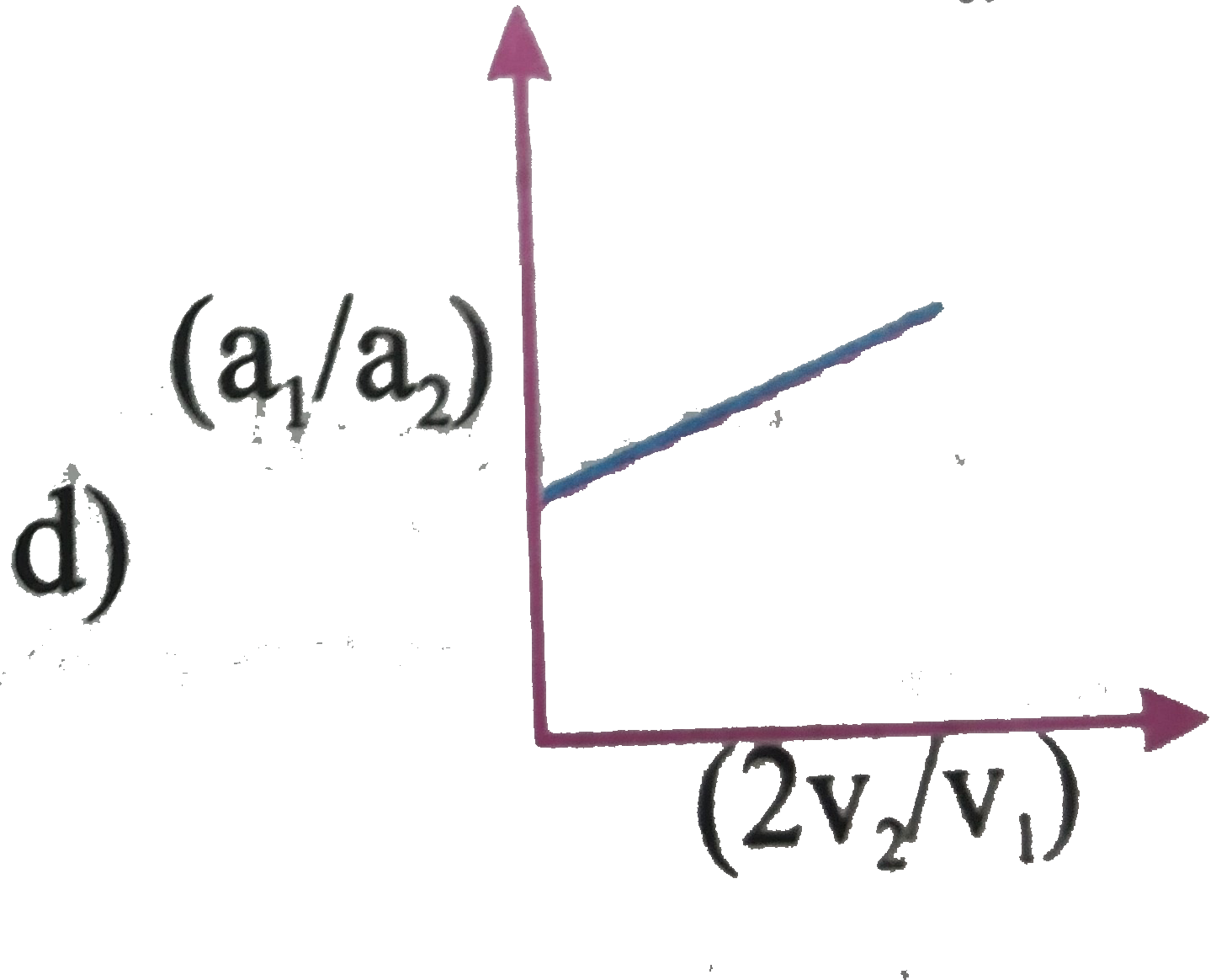
A vessel completely filled with water has holes 'A' and 'B' at depths 'h' and '2h' from the top, respectivel. Hole 'A' is a square of side 'L' and 'B' is circle of radish 'r' The water flowing out per second from both the holes is same Then, 'L'is equal to
View SolutionA tank filled with fresh water has a hole in its bottom and water is flowing out of it. If the size of the hole is increased, then
View SolutionThere are two holes P and Q at depths h and 4h from the top of a large vessel, completely filled with water. P is a square hole of side L and Q is a circular hole of radius r. If the same quantity of water flows out per second from both the holes, then the relation between L and r is
View SolutionA tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View SolutionThere is a hole h metre from its top of a tank filled with water. Calculate the distance x where the stream of water will touch the ground from the bottom (in m).
View Solution
