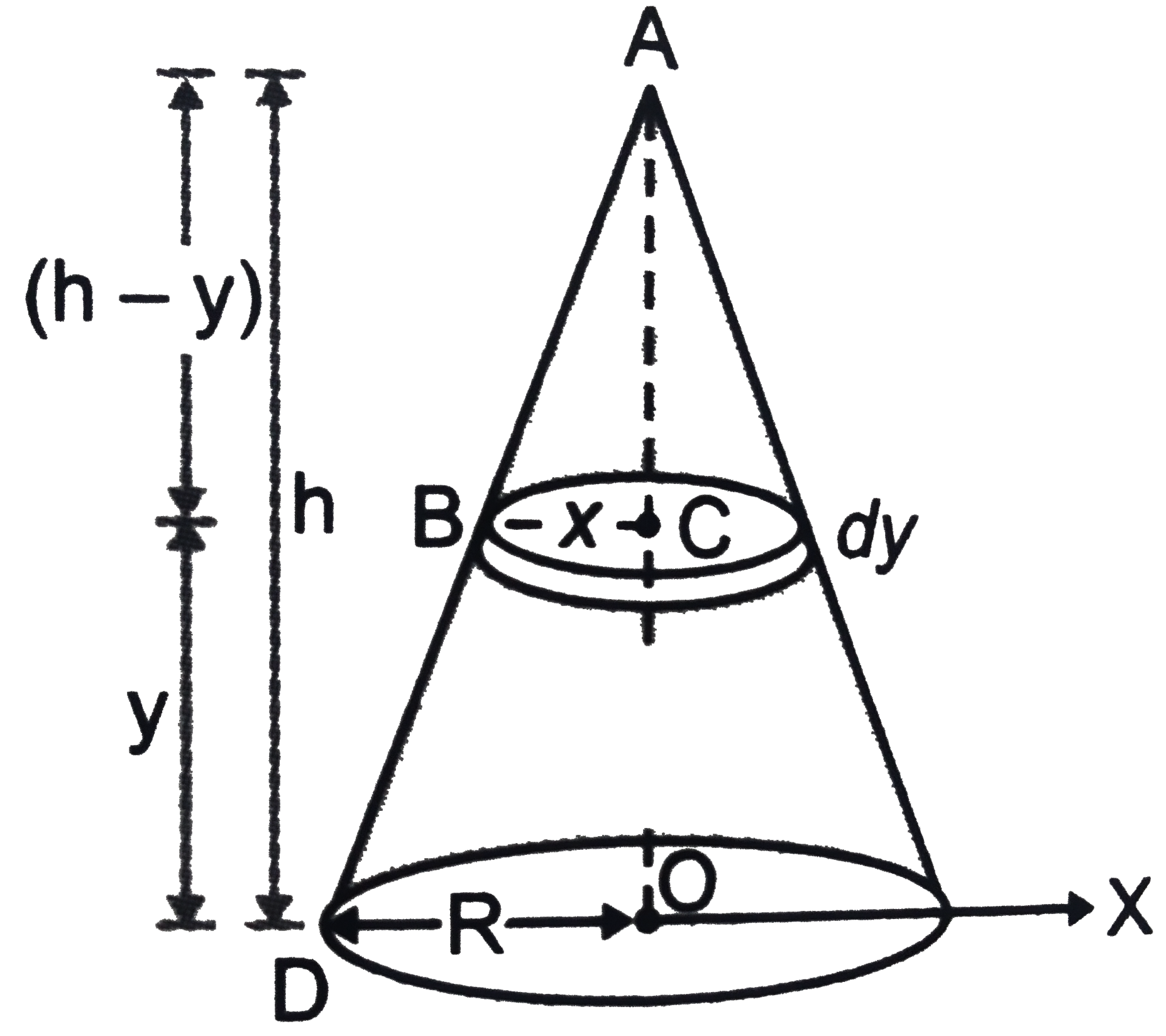
Determine the coordinates of the centre of mass of a right circular soild cone of base radius $R$ and height $h$ .

Video Solution

Text Solution

Verified by Experts

Fig. shown a unifrom soild cone of base radius $R$ and height $h$ . It is supposed to be made up of a large number of circular discs of different radii and masses.
Consider one such disc of radius $B C = x$ and mass $d m$ at a height $y$ from the base.
$Δ^{s} A B C$ and $A D O$ are similar,
$∴ \frac{A O}{A C} = \frac{D O}{B C} or \frac{h}{h - y} = \frac{R}{x}$
or $x = \frac{R (h - y)}{h}$ ..(i)
Mass of elementary disc, $d m =$ Volume xx density
$d m = π x^{2} . d y \times ρ = π ρ \frac{R^{2} {(h - y)}^{2}}{h^{2}} d y$ ..(ii)
$∴$ Co-ordinates of centre of mass of one are
$y_{c m} = \frac{1}{M} \int y d m = \frac{1}{M} \int y . \frac{π ρ R^{2}}{h^{2}} {(h - y)}^{2} d y$
$= \frac{π ρ R^{2}}{M h^{2}} h \int 0 (h^{2} y + y^{3} - 2 h y^{2}) d y$
$y_{c m} = \frac{π ρ R^{2}}{\frac{1}{3} π R^{2} h ρ \times h^{2}} \times \frac{h^{4}}{12} = \frac{h}{4}$
$(∵ M = \frac{1}{3} π r^{2} h ρ)$
Similarly $x_{c m} = 0, z_{c m} = 0$
Hence co-ordinates of the centre of mass of soild
cone are $(0, \frac{h}{4}, 0)$ .

Updated on:21/07/2023

The volume of a right circular cone of height $12 c m$ and base radius $6 c m,$ is
A $(12 π) c m^{3}$
B $(36 π) c m^{3}$
C $(72 π) c m^{3}$
D $(144 π) c m^{3}$
A metallic sphere of diameter 20 cm is recast into a right circular cone of base radius 10 cm. What is the bigger height of the cone ?
A4 cm
B40 cm
C60 cm
D120 cm