Question

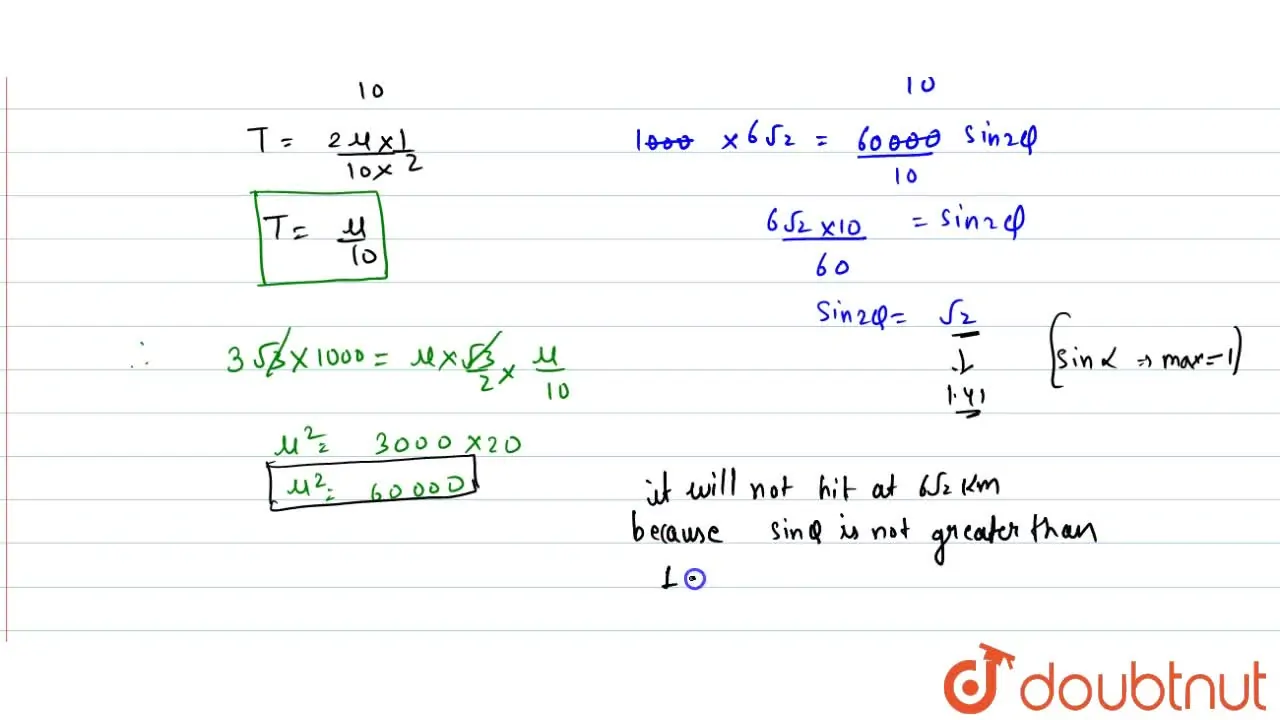
A bullet fired at an angle of ${\displaystyle {30}^{\circ }}$ with the horizontal hits the ground ${\displaystyle 3\sqrt{3}km}$ away. Can wr hit a target at a distance of ${\displaystyle 6\sqrt{2}km}$ by adjustion its angle of projection?

Explanation

Here, `theta =30^(@)`,
`R=3 sqrt 3 km =300 sqrt3 m`
As, `R=(u^(2) sin 2 theta)/g`
:. 3000 sqrt 3 =(u^(2) sin 2 xx 30^(@))/g`
or `u^(2)/g =(3-00 sqrt 3)/(sin 50^(@)) =(3000sqrt 3)/(sqer3//2)`
`=6000 m =6 km `
The maximum horizontal rage `=u^(2)/g =6 km`,
which is less than `6 sqrt2 km`. Thus the bullet will never hit the target at a distance `6 sqrt 2 km ` by adjusting anlge of projection.