The velocity of a body moving in a straight line is increased by applying a constant force F, for some distance in the direction of the motion. Prove that the increase in the kinetic energy of the body is equal to the work done by the force on the body.

Video Solution

Text Solution

Verified by Experts

Les s be the distance covered by the body moving in the direction of constant force F. Work done by this force on the body,
W = F s ........(i)
If a is the acceleration produced in the body of mass m due to this constant force F and if its velocity changes from u to $υ$ then
F = ma.....(ii)
and $υ^{2} - u^{2} = 2 a s or s = \frac{υ^{2} - u^{2}}{2 a} ... . . (i i i)$
From eqns. (i), (ii) and (iii), $W = (m a) (\frac{υ^{2} - u^{2}}{2 a}) = \frac{1}{2} μ ψ l o n^{2} - \frac{1}{2} μ^{2}$
Since $\frac{1}{2} μ ψ l o n^{2} - \frac{1}{2} μ^{2}$ = final kinetic energy - initial kinetic energy
= increases in kinetic energy,
Work done, W = increases in kinetic energy.

Updated on:21/07/2023

If $K_{i}$ and $K_{f}$ are the initial and final values of kinetic energy of a body respectively, then the work done by the net force on the body is equal to
A $\frac{K_{f} K_{i}}{K_{f} - K_{i}}$
B $K_{f} + K_{i}$
C $\frac{K_{f} + K_{i}}{2}$
D $K_{f} - K_{i}$
If kinetic energy of a body is increasing then.
Awork done by conservative forces must be positive.
Bwork done by conservative forces may be positive.
Cwork done by conservative forces may be zero
Dwork done by non-conservative forces may be zero
The kinetic energy of a body moving along a straight line varies with time as shown in figure. The force acting on the body:

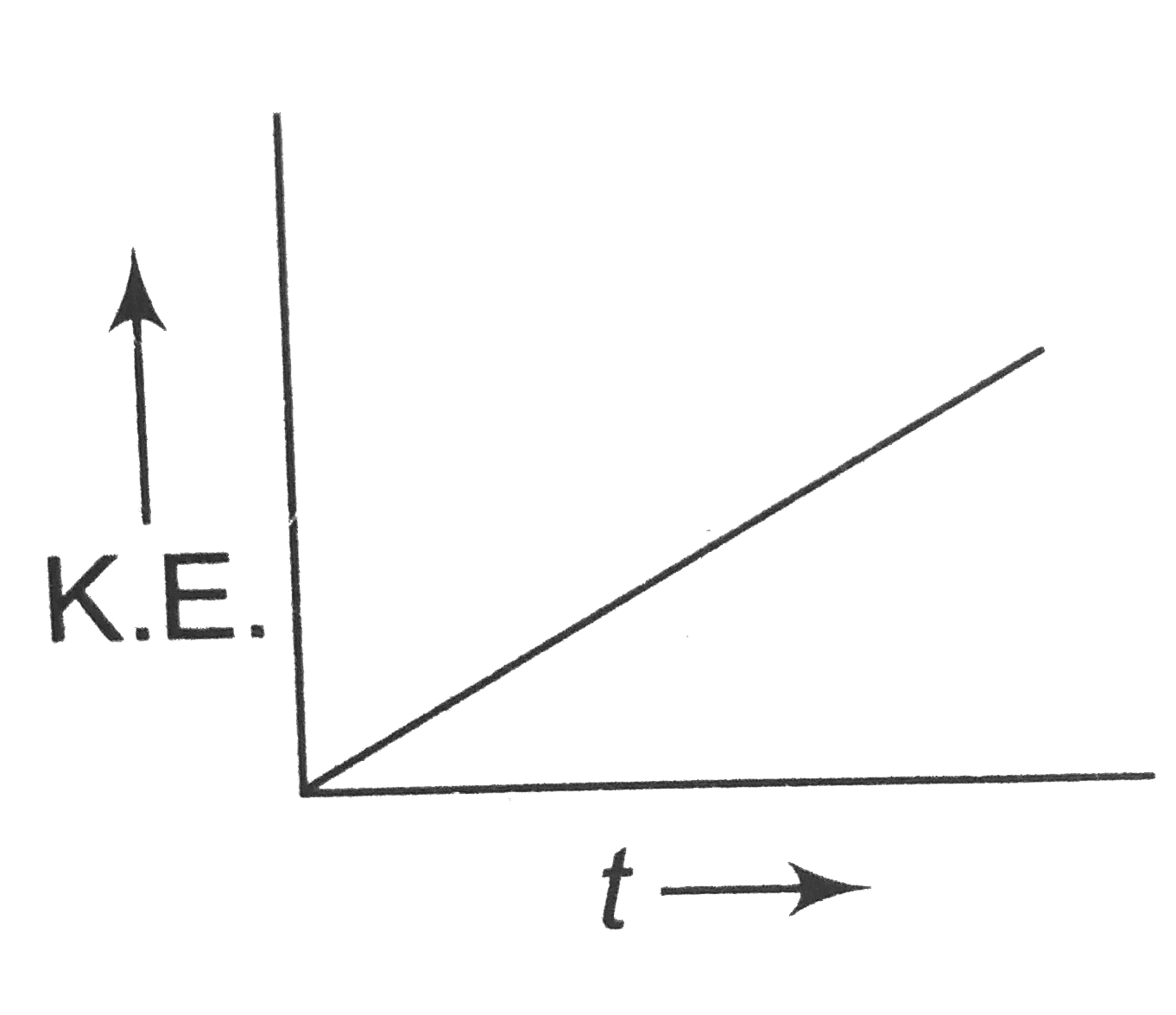Azero
Bconstant
Cdirectly proportional to velocity
Dinversely proportional to velocity