Find the thrust acting on the human body due to atmospheric pressure. Take the surface area of a man of middle size to be 1.5m2 and atmospheric pressure (1 atm)=1.013×105 Pa.
Here, surface area of the human body, A=1.5m2
pressure acting on the body, P=1.013×105Pa=1.013×105Nm2
Thus, thrust acting on the human body, F=P×A=(1.013×105Nm2)×(1.5m2)
=1.52×105N
=1.52×104kgwt=15.2→nwht
Topper's Solved these Questions
Similar Questions
Knowledge Check
Find the mean free path and collision frequency of a hydrogen molecule in a cylinder containing hydrogen at 3atm and temperature 27∘C. Take the radius of a hydrogten molecule to be 10A. Given, one atmospheric pressure =1.013×105Nm−2 and molecular mass of hydrogen=2.
A7.66×10−8m,2.52×105s−1B7.66×10−5m,2.52×105s−1C7.66×10−8m,2.52×1010D7.66×10−10m,2.52×108s−1The pressure inside a small air bubble of radius 0.1mm situated just below the surface of water will be equal to [Take surface tension of water 70×10−3Nm−1 and atmospheric pressure=1.013×105Nm−2]
A2.954×103PaB1.027×103PaC1.027×105PaD2.054×105Pa
Calculate the pressure at a depth of 10 m in an Ocean. The density of sea water is 1030kg/m3. The atmospheric pressure is 1.01×105 pa.
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
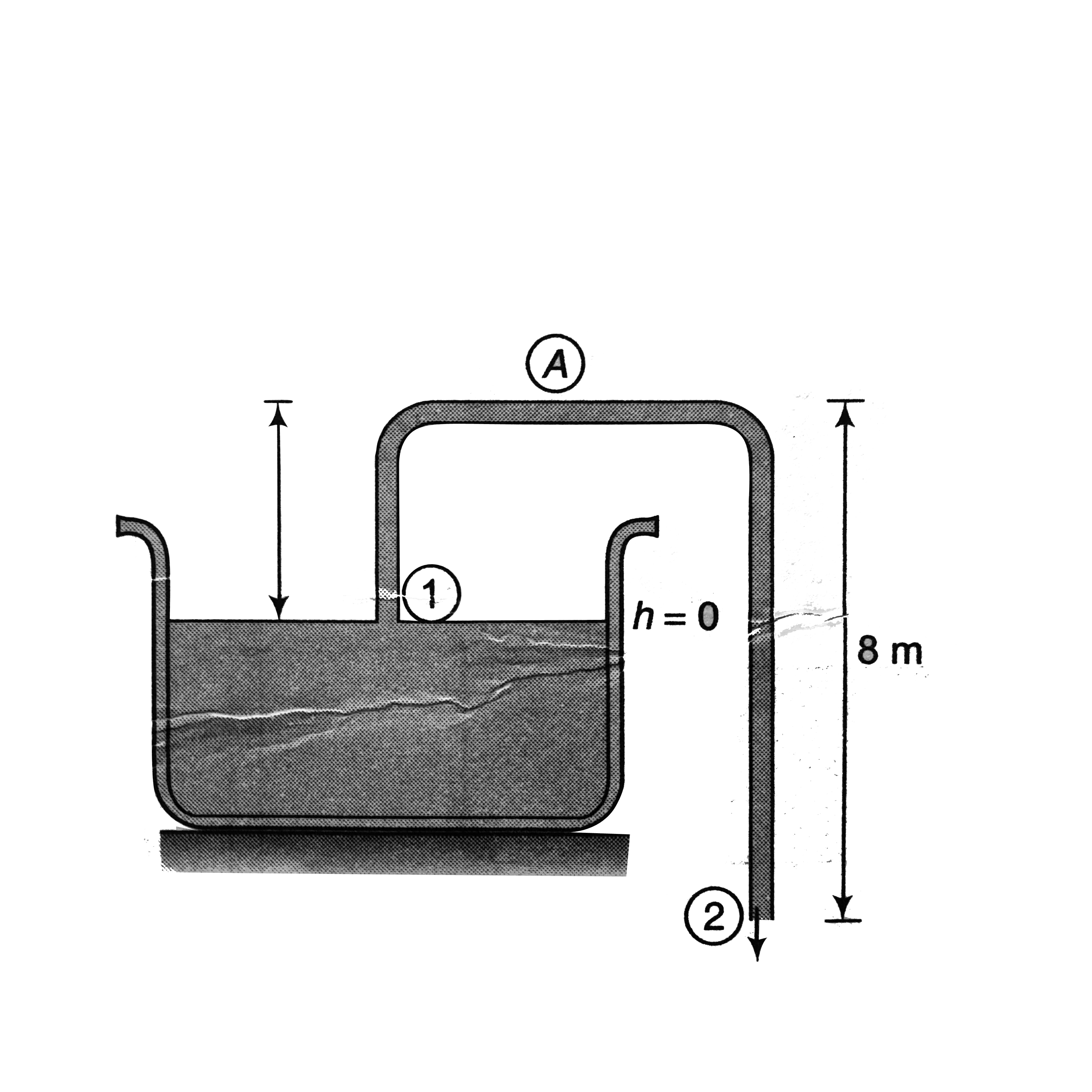 .
.
View SolutionAt a depth of 1000 m in an ocean (a) what is the absolute pressure? (b) what is the auge pressure? (c ) Find the force acting on the windown of area 20cm×20cm of a submarine at this depth, the interior of which is maintained at sea-level atmospheric pressure. The density of sea water is 1.03×103kgm−3, g=10ms−2. Atmospheric pressure = 1.01×105Pa.
View SolutionA raindrop of diamter 4mm is about to fall on the ground. Calculate the pressure inside the raindrop. [Surface tension of water T=0.072N/m, atmospheric pressure =1.013×105N/m2]
View SolutionWhat is the absolute pressure on a swimmer 10m below the surface of a lake? Take atmospheric pressure 1×105N/m2
View SolutionWhat should be the pressure inside a small air bubble of 0.1mm radius situated just below the surface of water? Surface tension of water =72×10−3N/m and atmospheric pressure =1.013×105N/m2
View Solution
