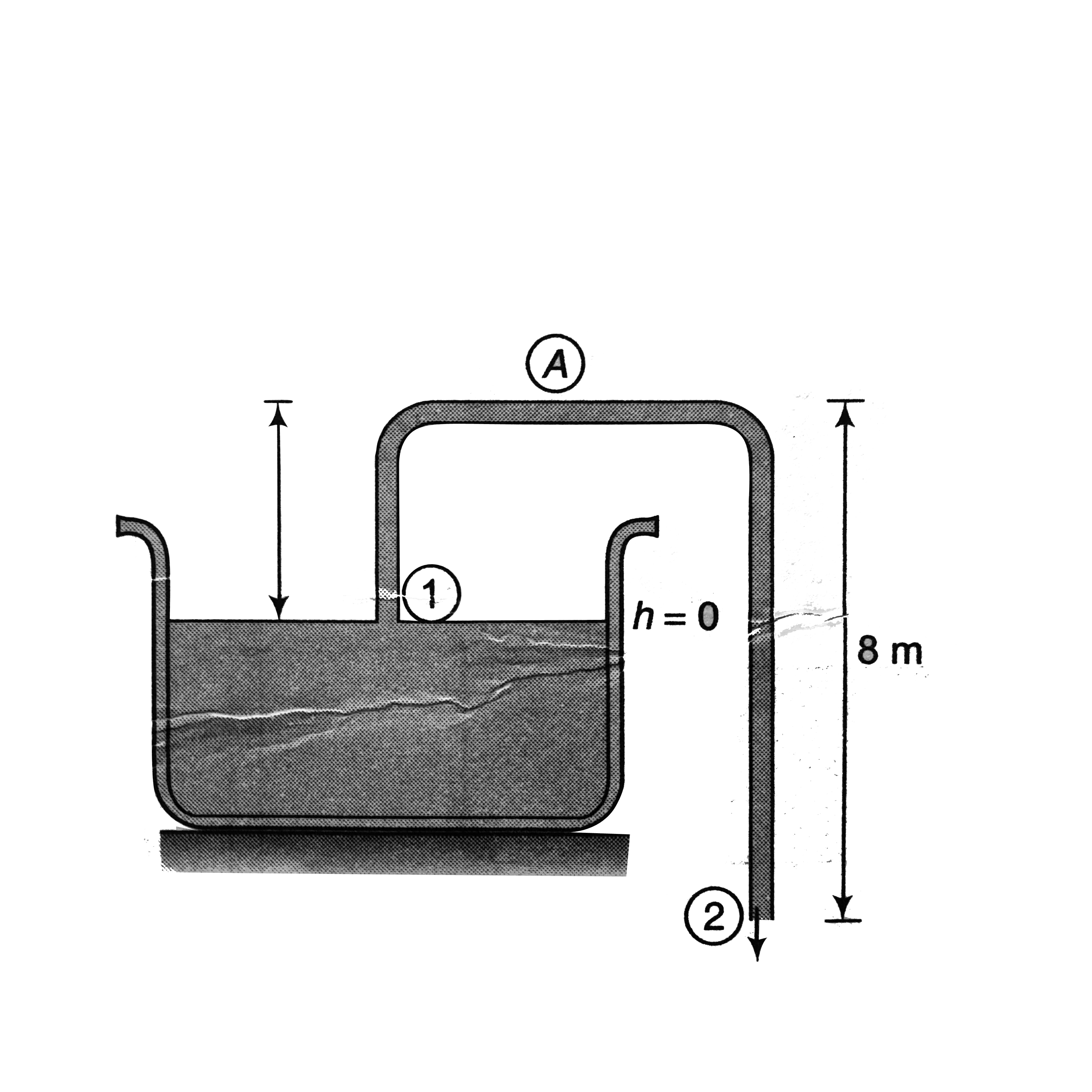
The U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure $= 1.01 \times 10^{5} N / m^{2}, g = 9.8 m / s^{2}$ and density of water $= 10^{3} k g / m^{3}$
.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, D

(a) Applying Bernoulli's equation between points (1) and (2)
$P_{1} + \frac{1}{2} ρ v_{1}^{2} + ρ g h_{1} = p_{2} + \frac{1}{2} ρ v_{2}^{2} + ρ g h_{2}$
Since, area of reservoirgtgt area of pipe
$v_{1} \approx 0$ .
also, $p_{1} = p_{2} =$ atmospheric pressure
so, $v_{2} = \sqrt{2 g (h_{1} - h_{2})}$
$= \sqrt{2 \times 9.8 \times 7}$
$= 11.7 m / s$
(b) The minimum pressure in the bend will be at A. Therefore, applying Bernoulli's equation between (1) and (A)
$p_{1} + \frac{1}{2} ρ v_{1}^{2} + ρ g h_{1} = P_{A} + \frac{1}{2} ρ v_{A}^{2} + ρ g h_{A}$
Again, $v_{1} \approx 0$ and from continuity equation $v_{A} - v_{2}$
or, $p_{A} = p_{1} + ρ g (h_{1} - h_{A}) - \frac{1}{2} ρ v_{2}^{2}$
Therefore, substituting the value , we have
$p_{A} = (1.01 \times 10^{5}) + (1000) (9.8) (- 1) - \frac{1}{2} \times (1000) {(11.7)}^{2}$
$= 2.27 \times 10^{4} N / m^{2}$ .

Updated on:21/07/2023

|

Class 11 PHYSICS FLUID MECHANICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

What is the pressure at the bottom of the ocean at a place where it is 3 km deep ?
[atmospheric pressure $= 1.01 \times 10^{5} P a,$ Density of sea water $= 1030 k g m^{- 30}]$
A $1.01 \times 10^{5} P a$
B $3 \times 10^{5} P a$
C $1.01 \times 10^{7} P a$
D $3 \times 10^{7} P a$

DC PANDEY-FLUID MECHANICS-Solved Examples

Home
Ask Doubt
Profile