A girl of mass jumps with a horizontal velocity of onto a stationary cart with frictionless wheels. The mass of the cart is . What is her velocity as the cart starts moving? Assume that there is no external unbalanced force working in the horizontal direction.
100/43 m/s
200 m/s
200/43 m/s
NONE
The correct Answer is:C
To solve this problem, we will use the principle of conservation of momentum. The total momentum before the girl jumps onto the cart must equal the total momentum after she jumps onto it, as there are no external unbalanced forces acting on the system.
1. Identify the masses and initial velocities:
- Mass of the girl, mg=40kg
- Initial velocity of the girl, vg=5m/s
- Mass of the cart, mc=3kg
- Initial velocity of the cart, vc=0m/s
2. Calculate the initial momentum (Pi):
The initial momentum of the system is the sum of the momentum of the girl and the cart.
Pi=mg⋅vg+mc⋅vc
Since the cart is stationary, vc=0:
Pi=(40kg⋅5m/s)+(3kg⋅0m/s)=200kg m/s
3. Calculate the final momentum (Pf):
After the girl jumps onto the cart, the total mass of the system is mg+mc and they move together with a common velocity V.
Pf=(mg+mc)⋅V
Pf=(40kg+3kg)⋅V=43kg⋅V
4. Apply the conservation of momentum:
According to the law of conservation of momentum:
Pi=Pf
200kg m/s=43kg⋅V
5. Solve for the final velocity (V):
Rearranging the equation to find V:
V=200kg m/s43kg≈4.65m/s
Final Answer:
The velocity of the girl and the cart as they start moving together is approximately 4.65m/s.
---
- Mass of the girl, mg=40kg
- Initial velocity of the girl, vg=5m/s
- Mass of the cart, mc=3kg
- Initial velocity of the cart, vc=0m/s
The initial momentum of the system is the sum of the momentum of the girl and the cart.
Pi=mg⋅vg+mc⋅vc
Since the cart is stationary, vc=0:
Pi=(40kg⋅5m/s)+(3kg⋅0m/s)=200kg m/s
After the girl jumps onto the cart, the total mass of the system is mg+mc and they move together with a common velocity V.
Pf=(mg+mc)⋅V
Pf=(40kg+3kg)⋅V=43kg⋅V
According to the law of conservation of momentum:
Pi=Pf
200kg m/s=43kg⋅V
Rearranging the equation to find V:
V=200kg m/s43kg≈4.65m/s
The velocity of the girl and the cart as they start moving together is approximately 4.65m/s.
Topper's Solved these Questions
- FORCES AND LAWS OF MOTIONBook:PRADEEPChapter:FORCES AND LAWS OF MOTIONExercise:ORAL TESTING
Explore 20 Videos
- FORCES AND LAWS OF MOTIONBook:PRADEEPChapter:FORCES AND LAWS OF MOTIONExercise:QUIZ TESTING
Explore 12 Videos
- FORCES AND LAWS OF MOTIONBook:PRADEEPChapter:FORCES AND LAWS OF MOTIONExercise:VALUE BASED QUESTIONS
Explore 4 Videos
- FLOTATIONBook:PRADEEPChapter:FLOTATIONExercise:Problem For Practice
Explore 6 Videos
- GRAVITATIONBook:PRADEEPChapter:GRAVITATIONExercise:Model Test paper (1)
Explore 36 Videos
Similar Questions
(i) How much momentum will a dumb-bell of mass 10kg transfer to the floor if it falls a height of 80cm? Take its downward acceleration to be 10m/s2.
(b) A girl of mass 40kg running at a speed of 5m/s horizontally jumps onto a stationary cart with frictionless wheels. The mass of the cart is 3kg. What is her velocity as the cart starts moving?
View SolutionA girl of mass 50 kg jumps out of a rowing boat of mass 300 kg on to the bank, with a horizontal velocity of 3 m/s. With what velocity does the boat begin to move backwards ?
View Solution
Knowledge Check
A boy of mass 50 kg jumps with horizontal velocity of 1 m/s on to a stationary cart. If mass of the cart will be [Assume friction between the wheels the cart and road is zero ]
A1011 m/sB1110 m/sC0⋅65 m/sD0⋅8 m/sAn object of mass 2kg is sliding with a constant velocity of 4ms−1 on a frictionless horizontal table. The force required to keep the object moving with the same velocity is
A32NB0 NC2ND8NA bullet, of mass 40g is horizontally fired with a velocity 150ms−1 from a pistol of mass 3kg. What is the recoil velocity of the pistol?
A−1.25ms−1B−3.0ms−1C−1.5ms−1D−2.0ms−1
A boy of mass 60kg running at 3/s jumps on to a trolley of mass 140kg moving with a velocity of 1.5m/s in the same direction . What is their common velocity?
View SolutionA boat of mass 1000 kg is moving with a velocity of 5 m/s. A person of mass 60 kg jumps into the boat. The velocity of the boat with the person will be -
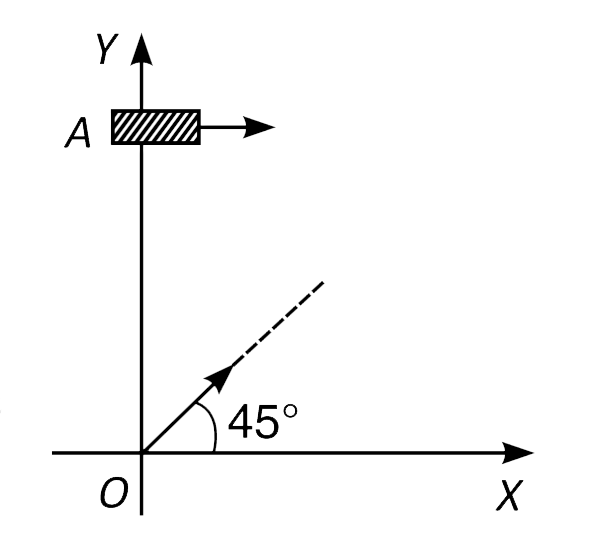
View SolutionA small cart A starts moving on a horizontal surface, assumed to be x-y plane along a straight line parallel to x-axis (see figure) with a constant acceleration of 4m/s2. Initially it is located on the positive y-axis at a distance 9 m from origin. At the instant the cart starts moving, a ball is rolled along the surface from the origin in a direction making an angle 45° with the x-axis. The ball moves without friction at a constant velocity and hits the cart.
(a) Describe the path of the ball in a reference frame attached to the cart.
(b) Find the speed of the ball.
View SolutionA body of mass 1kg is moving along a straight line with a velocity of 1m/s. The external force acting in the body is
View SolutionA man and a cart move towards each other. The man weight 64kg and the cart weighs 32kg. The velocity of the man is 5.4km/hr and that of the cart he jumps on to it The velocity of the cart carrying the man will be .
View Solution