A wave travels on a light string. The equation of the wave is Y=Asin(kx−ωt+θ)). It is reflected from a heavy string tied to an end of the light string at x=0. If 64% of the incident energy is reflected the equation of the reflected wave is
y=0.5Asin(kx+ωt+θ)
y'=−0.5Asin(kx+ωt+θ)
y'=−0.5Asin(ωt−kx−θ)
y'=−0.5Asin(kx+ωt−θ)
The correct Answer is:D
As wave has benn reflected from a rares medium therefore there is no change in phase. Hence equation for the oppsite direction can be written as
y=0.1Asin(−kz−ωt+ϕ)
=−0.5Asin(kx+ωt−θ)
Topper's Solved these Questions
- WAVES AND ACOUSTICSBOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Beats
20 Videos
- WAVES AND ACOUSTICSBOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Stationary Waves
20 Videos
- WAVES AND ACOUSTICSBOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Equation And Energy Of Progressive Wave
16 Videos
- VECTORSBOOK - A2ZCHAPTER - VECTORSEXERCISE - Chapter Test
29 Videos
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Chapter Test
29 Videos
Similar Questions
Knowledge Check
A wave travels on a light string. The equation of the waves is y=Asin(kx−ωt+30∘). It is reflected from a heavy string tied to an end of the light string at x = 0. If 64% of the incident energy is reflected, then the equation of the reflected wave is
Ay=0.8Asin(kx−ωt+30∘+180∘)By=0.8Asin(kx+ωt+30∘+180∘)Cy=0.8Asin(kx−ωt−30∘)Dy=0.8Asin(kx−ωt+30∘)A wave travels on a light string. The equation of the wave is Y=Asin(kx−ωt+30∘+180∘). It is relected from a heavy string tied to an end of the light string at x=0. If 64% of the incident energy is reflected the equation of the reflected wave is
AY=0.8Asin(kx−ωt+30∘+180∘)BY=0.8Asin(kx+ωt+30∘+180∘)CY=0.8Asin(kx+ωt−30∘)DY=0.8Asin(kx+ωt+30∘)A travelling wave y=Asin(kx−ωt+θ) passes from a heavier string to a lighter string . The juction of the strings is at x=0. The equation of the reflected wave is
Ay'=0.5Asin(kx+ωt+θ)By'=−0.5Asin(kx+ωt+θ)Cy'=−0.5Asin(kx−ωt−θ)Dy'=−0.5Asin(kx+ωt−θ)
A transverse wave is passing through a light string shown in fig.The equation of wave is y=Asin(wt−kx) the area of cross-section of string A and density is ρ the hanging mass is :
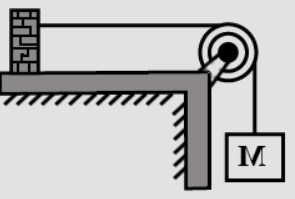
View SolutionA transverse wave is passing through a light string shown in fig.The equation of wave is y=Asin(wt−kx) the area of cross-section of string A and density is ρ the hanging mass is

View SolutionA travelling wave y=Asin(kx−ωt+θ) passes from a heavier string to a lighter string. The reflected wave has amplitude 0.5A . The junction of the strings is at x=0. The equation fo the refelcted wave is :
View SolutionThe equation of a wave travelling on a string is d
View SolutionThe equation of a wave is y=Asin(2πnt)When it is reflected at a free end its amplitude becomes 90% The equation of the reflected wave is
View Solution
