If rod is thrown upward with initial angular velocity and velocity of centre of mass then its momentum changes but angular velocity remains same.
Torque on rod about cente of mass due to gravitational force is zero.
If both assertion and reason are true and reason is the correct explanation of assertion.
If both assertion and reason are reason are true but reason is not the correct explanation of assertion.
If assertion is true but reason is false
If both assertion and reason are false.
The correct Answer is:A
(a) Since net torque is zero angular velocity remains constant.
Topper's Solved these Questions
- ROTATIONAL DYNAMICSBook:A2ZChapter:ROTATIONAL DYNAMICSExercise:NEET Questions
Explore 59 Videos
- ROTATIONAL DYNAMICSBook:A2ZChapter:ROTATIONAL DYNAMICSExercise:AIIMS Questions
Explore 40 Videos
- ROTATIONAL DYNAMICSBook:A2ZChapter:ROTATIONAL DYNAMICSExercise:Problems Based On Mixed Concepts
Explore 31 Videos
- PROPERTIES OF MATTERBook:A2ZChapter:PROPERTIES OF MATTERExercise:Chapter Test
Explore 29 Videos
- THERMAL PROPERTIES OF MATTERBook:A2ZChapter:THERMAL PROPERTIES OF MATTERExercise:Chapter Test
Explore 30 Videos
Similar Questions
If no external torque acts on a body, will its angular velocity remain conserved ?
View SolutionA uniform rod AB of length 'l' is thrown upwards such that initially AB is horizontal velocity of centre is 'u' upwards and angular velocity ' omega ' is such that velocity of ' B ' at this moment is zero. The values of omega 'and 'u' are also such that the rod becomes vertical first time at the moment when the centre of rod reaches the highest point of its motion Q: The value of omega ' in terms of u ' and
View Solution
Knowledge Check
If 'A' is areal velocity of a planet of mass M, its angular momentum is
AM/AB2MACA2MDAM2A rod is rotating with angular velocity ω about axis AB. Find cosθ.
 Ag2lω2Bglω2C2g(lω)2D3g2lω2
Ag2lω2Bglω2C2g(lω)2D3g2lω2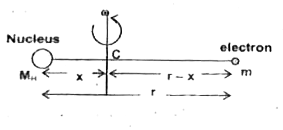
The angular momentum of the atom about the centre of mass will beAmr2ωBm(r−X)2ωC(MH+M)r2ωMHm2DMHmMH+mr2ω
A uniform rod of length l is spinning with an angular velocity ω=2vl while its centre of mass moves with a velocity v. Find the velocity of the end of the rod.
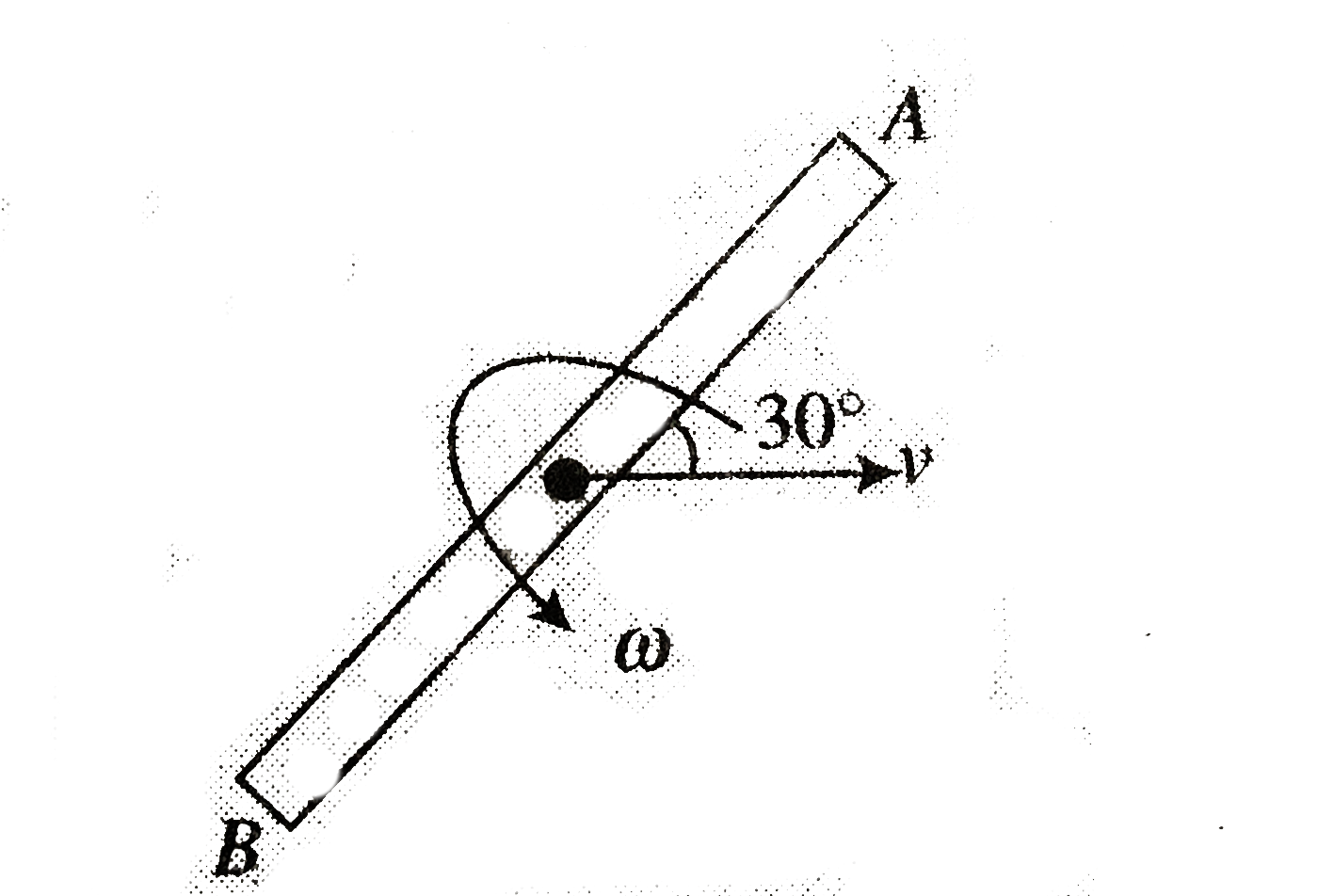
View SolutionA mass is whirled in a circular path with constant angular velocity and its angular momentum is L. If the string is now halved keeping the angular velocity the same, the angular momentum is
View SolutionA body of mass m and radius of gyration K has an angular momentum L. Then its angular velocity is
View SolutionA mass is whirled in a circular path with a constant angular velocity and its angular momentum is L. If the string is now halved keeping the angular velocity same, the angular momentum is
View SolutionA particle moves in a circle with constant angular velocity ω about a point P on its circumference. The angular velocity of the particle about the centre C of the circle is
View Solution