One end of string of length l is connected to a particle on mass m and the other end is connected to a small peg on a smooth horizontal table. If the particle moves in circle with speed v the net force on the particle (directed toward centre) will be (T reprents the tension in the string):
T+mv21
T−mv21
zero
T
The correct Answer is:D
To solve the problem, we need to analyze the forces acting on the particle moving in a circular path. The particle of mass m is connected to a string of length l that is fixed at one end to a peg on a smooth horizontal table. The particle is moving in a circle with a constant speed v.
1. Identify the Forces Acting on the Particle:
- The only forces acting on the particle are the tension T in the string and the gravitational force mg acting downward. However, since the table is horizontal and smooth, we only need to consider the horizontal forces for circular motion.
2. Understand Circular Motion:
- For an object moving in a circular path, there must be a net inward force (centripetal force) acting towards the center of the circle. This net force is provided by the tension in the string.
3. Centripetal Force Requirement:
- The required centripetal force Fc for an object of mass m moving with speed v in a circular path of radius r is given by the formula:
Fc=mv2r
- In this case, the radius r is equal to the length of the string l.
4. Set Up the Equation:
- Since the tension T in the string provides the necessary centripetal force, we can equate the tension to the centripetal force:
T=mv2l
5. Conclusion:
- The net force acting on the particle directed towards the center of the circular path is simply the tension in the string. Therefore, the net force on the particle is:
T=mv2l
Final Answer:
The net force on the particle directed toward the center is given by:
T=mv2l
- The only forces acting on the particle are the tension T in the string and the gravitational force mg acting downward. However, since the table is horizontal and smooth, we only need to consider the horizontal forces for circular motion.
- For an object moving in a circular path, there must be a net inward force (centripetal force) acting towards the center of the circle. This net force is provided by the tension in the string.
- The required centripetal force Fc for an object of mass m moving with speed v in a circular path of radius r is given by the formula:
Fc=mv2r
- In this case, the radius r is equal to the length of the string l.
- Since the tension T in the string provides the necessary centripetal force, we can equate the tension to the centripetal force:
T=mv2l
- The net force acting on the particle directed towards the center of the circular path is simply the tension in the string. Therefore, the net force on the particle is:
T=mv2l
The net force on the particle directed toward the center is given by:
T=mv2l
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - AIIMS Questions
26 Videos
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Chapter Test
30 Videos
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Assertion Reasoning
14 Videos
- MOTION IN TWO DIMENSIONBOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test
29 Videos
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Chapter Test
29 Videos
Similar Questions
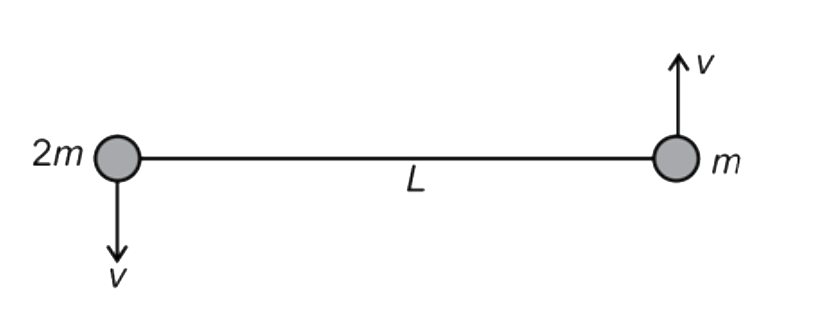
Two particles of mass m and 2m are connected by a string of length L and placed at rest over a smooth horizontal surface. The particles are then given velocities as indicated in the figure shown. The tension developed in the string will be
View SolutionA block of mass 1kg is tied to a string of length 1m the other end of which is fixed The block is moved on a smooth horizontal table with constant speed 10ms−1. Find the tension in the string
View Solution
Knowledge Check
One end of string of length / is connected to a particle of mass 'm' and the other end is connected to a small peg on a smooth horizontal table. If the particle moves in circle with speed 'v', the net force on the particle (directed towards centre) will be (T represents the tension in the string)
AT+mv2lBT−mv2lCzeroDTOne end of string of length l is connected to a particle on mass m and the other end is connected to a small peg on a smooth horizontal table. If the particle moves in circle with speed v the net force on the particle (directed toward centre) will be (T reprents the tension in the string):
AT+mv2IBT−mv2lCZeroD1A particle of mass m is suspended from the ceiling through a string of length L. The particle moves in a horizontal circle of radius r. The speed of the particle is
Arg√L2−r2Br√g(L2−r2)14Cr√g(L2−r2)12DmgL(L2−r2)12
A paricle of mass m is tied to a light string of length L and moving in a horizontal circle of radius r with speed v as shown. The forces acting on the particle are

View SolutionTwo particles of masses m and M(M>m) are connected by a cord that passes over a massless, frictionless pulley. The tension T in the string and the acceleration a of the particles is
View SolutionA particle of mass m is attached to one end of a string of length l while the other end is fixed to a point h above the horizontal table. The particle is made to revolve in a circle on the table so as to make p revolutions per second. The maximum value of p if the particle is to be in contact with the table will be :
View SolutionA particle of mass m1 is fastened to one end of a massless string and another particle of mass m2 is fastened to the middle point of the same string. The other end of the string being fastened to a fixed point on a smooth horizontal table. The particles are then projected, so that the two particles and the string are always in the same straight line and describe horizontal circles. Then, the ratio of rotations in the two parts of the string is :
View SolutionA particle of mass m1 is fastened to one end of a string and one of m2 to the middle point, the other end of the string being fastened to a fixed point on a smooth horizontal table The particles are then projected, so that the two portions of the string are always in the same straight line and describes horizontal circles find the ratio of tensions in the two parts of the string

View Solution
