The distance travelled by a particle starting from rest and moving with an acceleration 43ms−2, in the third second is.
6 m
4 m
103m
193m
The correct Answer is:C
To find the distance travelled by a particle starting from rest and moving with an acceleration of 43m/s2 in the third second, we can use the formula for the distance travelled in the nth second:
sn=u+12a(2n−1)
Where:
- sn is the distance travelled in the nth second,
- u is the initial velocity,
- a is the acceleration,
- n is the second for which we want to find the distance.
1. Identify the given values:
- Initial velocity u=0m/s (since the particle starts from rest).
- Acceleration a=43m/s2.
- We want to find the distance travelled in the third second, so n=3.
2. Substitute the values into the formula:
s3=u+12a(2n−1)
Substituting the known values:
s3=0+12(43)(2×3−1)
3. Calculate 2n−1:
2n−1=2×3−1=6−1=5
4. Substitute this back into the equation:
s3=12(43)×5
5. Calculate 12×43:
12×43=46=23
6. Now multiply by 5:
s3=23×5=103m
7. Final result:
The distance travelled by the particle in the third second is 103m.
Summary:
The distance travelled by the particle in the third second is 103m.
- sn is the distance travelled in the nth second,
- u is the initial velocity,
- a is the acceleration,
- n is the second for which we want to find the distance.
- Initial velocity u=0m/s (since the particle starts from rest).
- Acceleration a=43m/s2.
- We want to find the distance travelled in the third second, so n=3.
s3=u+12a(2n−1)
Substituting the known values:
s3=0+12(43)(2×3−1)
2n−1=2×3−1=6−1=5
s3=12(43)×5
12×43=46=23
s3=23×5=103m
The distance travelled by the particle in the third second is 103m.
The distance travelled by the particle in the third second is 103m.
Topper's Solved these Questions
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - AIIMS Questions
20 Videos
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Chapter Test
30 Videos
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Assertion Reasoning
20 Videos
- FLUID MECHANICSBOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Chapter Test
29 Videos
- GRAVITATIONBOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Chapter Test
29 Videos
Similar Questions
The distance travelled by a particle starting from rest and moving with an acceleration 4/3m/s2 ,in the third-second is
View SolutionThe ratio of the distances travelled by a body starting from rest and moving with uniform acceleration in the first, third and fifth seconds of its journey is
View Solution
Knowledge Check
A particle starts from rest and moves with constant acceleration. Then velocity displacement curve is:
A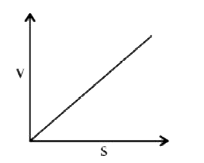 B
B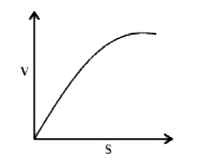 C
C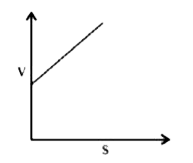 D
D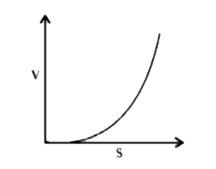
A particle starts moving with acceleration 2m/s2. Distance travelled by it in 5th half second is
A1.25 mB2.25 mC6.25 mD30.25 mThe ratio of distances traveled by two bodies, A and B starting form rest moving along a straight line with equal accelerations is x, where x≥1, then
Atime taken by body A > time taken by body B.Btime taken by body A < time taken by body B.Ctime taken by body A ≥ time taken by body B.Dtime taken by body A ≤ time taken by body B.
A particle starts from rest and moves with an acceleration of a={2+|t−2|}m/s2 The velocity of the particle at t=4 sec is
View SolutionA body starting from rest has an acceleration of 4ms−2 . Calculate distnce travelled by it in 5th second.
View SolutionA particle starting from rest attains a velocity of 50ms−1 in 5 s. The acceleration of the particle is
View SolutionA body starting from rest is moving with a uniform acceleration of 8ms2. Then the distance travelled by it in 5th second will be
View SolutionA body starting from rest and has uniform acceleration
8m/s2 . The distance travelled by it in5th second will be
View Solution
