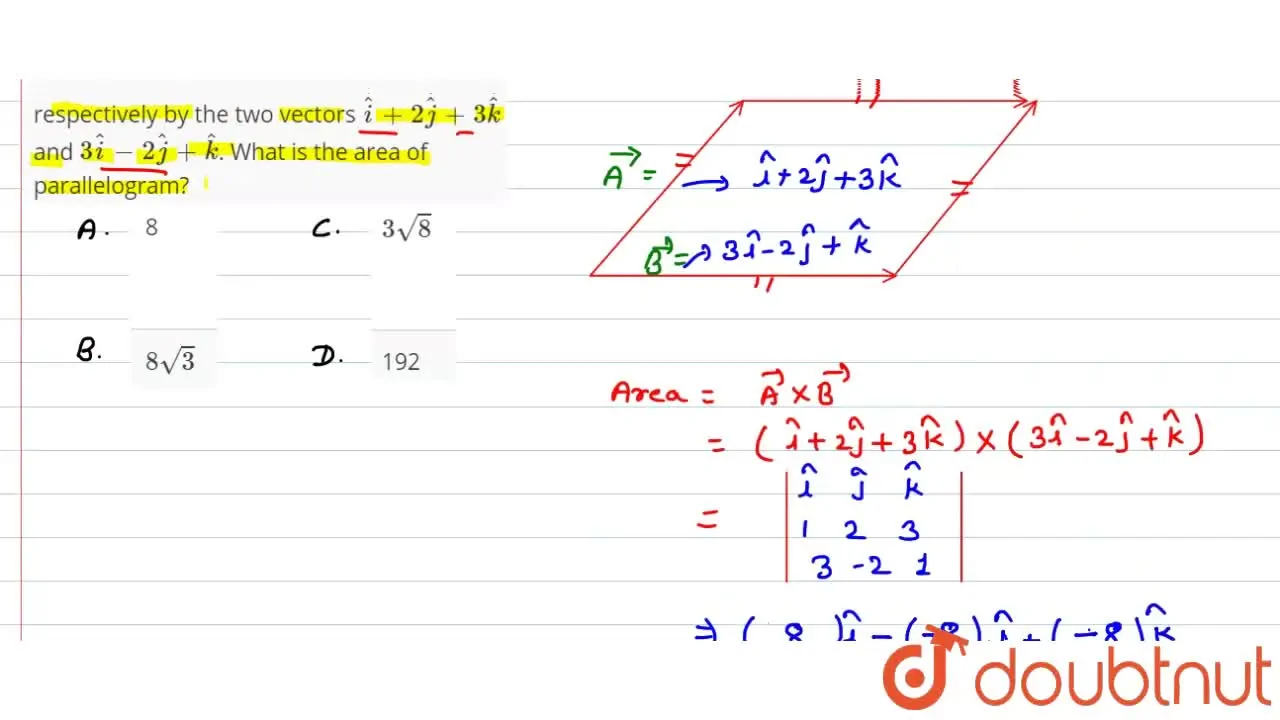
Two adjacent sides of a parallelogram are respectively by the two vectors $ˆ i + 2 ˆ j + 3 ˆ k$ and $3 ˆ i - 2 ˆ j + ˆ k$ . What is the area of parallelogram?

A

8

B

$8 \sqrt{3}$

C

$3 \sqrt{8}$

D

192

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Area of a parallelogram $= \to A \times \to B$
$= (ˆ i + 2 h A T (j) + 3 ˆ k) \times (3 ˆ i - 2 ˆ j + ˆ k)$
$= ∣ ∣ ∣ ∣ ∣ \begin{matrix} ˆ i & ˆ j & ˆ k 1 & 2 & 3 3 & - 2 & 1 \end{matrix} ∣ ∣ ∣ ∣ ∣ = (8) ˆ i + (8) ˆ j - (8) ˆ k$
Magnitude $= \sqrt{64 + 64 + 64} = 8 \sqrt{3}$

|

Updated on:21/07/2023

Class 11 PHYSICS VECTOR

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two adjacent sides of a parallelogram are respectively by the two vectors $ˆ i + 2 ˆ j + 3 ˆ k$ and $3 ˆ i - 2 ˆ j + ˆ k$ . What is the area of parallelogram?
A8
B $8 \sqrt{3}$
C $3 \sqrt{8}$
D192
Question 2 - Select One
The two vectors $A = 2 ˆ i + ˆ j + 3 ˆ k$ and $B = 7 ˆ i - 5 ˆ j - 3 ˆ k$ are :-
Aparallel
Bperpendicular
Canti-parallel
DNone of these
Question 3 - Select One
The sides of a parallelogram represented by vectors $p = 5 ˆ i - 4 ˆ j + 3 ˆ k$ and $q = 3 ˆ i + 2 ˆ j - ˆ k$ . Then the area of the parallelogram is :
A $\sqrt{684}$ unit
B $\sqrt{72}$ unit
C $\sqrt{191}$ unit
D72 unit

A2Z-VECTORS-Cross Product

Home
Ask Doubt
Profile