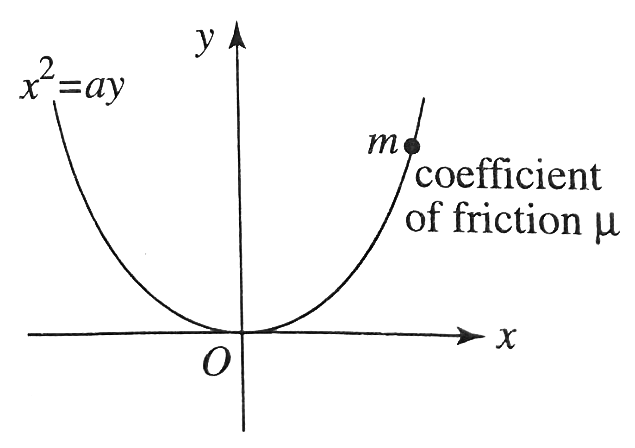
A bead of mass m is located on a parabolic wire with its axis vertical and vertex directed towards downwards as in figure and whose equation is x2=ay. If the coefficient of friction is μ, the highest distance above the x-axis at which the particle will be in equilibrium is
μa
μ2a
μ2a4
2μa
The correct Answer is:C
for the sliding not occur when
tanθ≤μ
tanθ=dydx=2xa=2√2√a=2√ya
2√ya≤μ or y≤aμ24
Similar Questions
Find the equation of the line intersecting the y-axis at a distance 2 units above the origin and making an angle of 300 with the positive direction of x-axis.
View SolutionFind the equation of the line which intersects the y-axis at a distance of 2 units above the origin and makes an angle of 300 with the positive direction of the x-axis.
View Solution
Knowledge Check

A bead of mass m is located on a parabolic wire with its axis vertical and vertex directed towards downwards as in figure and whose equation is x2=ay. If the coefficient of friction is μ, the highest distance above the x-axis at which the particle will be in equilibrium isAμaBμ2aC14μ2aD12μaA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equations is x2=4ay . The wire frame is fixed and the bead can slide on it without friction . The bead is released from the point y = 4a on the wire frame from rest . The tangential acceleration of the bead when it reaches the position given by y = a is :
 Ag2B(√3g)(2)Cg√2Dg
Ag2B(√3g)(2)Cg√2DgA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x2=4ay. The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is
 Ag2B√3g2Cg√2Dg
Ag2B√3g2Cg√2Dg
An inclined plane is bent in such a way that the vertical cross-section is given by y=x24 where y is in vertical and x in horizontal direction. If the upper surface of this curved plane is rough with coefficient of friction μ = 0.5, the maximum height in cm at which a stationary block will not slip downward is______cm.
View SolutionFind the equation of the straight line interesting y-axis at a distance of 2 units above the origin and making an angle of 00 with the positive direction of the x-axis
View SolutionA body of mass (m) is projected with a speed (v) making an angle θ with the vertical. What is the change in momentum of the body along (i) the X-axis (ii) the Y-axis , between the strting point and the highest point of its path.
View SolutionA smooth hollow cone whose vertical angle is 2α with its axis vertical and vertex downwards revolves about its axis η time per seconds. A particle is placed on the inner surface of cone so that it rotates with same speed. The radius of rotation for the particle is :
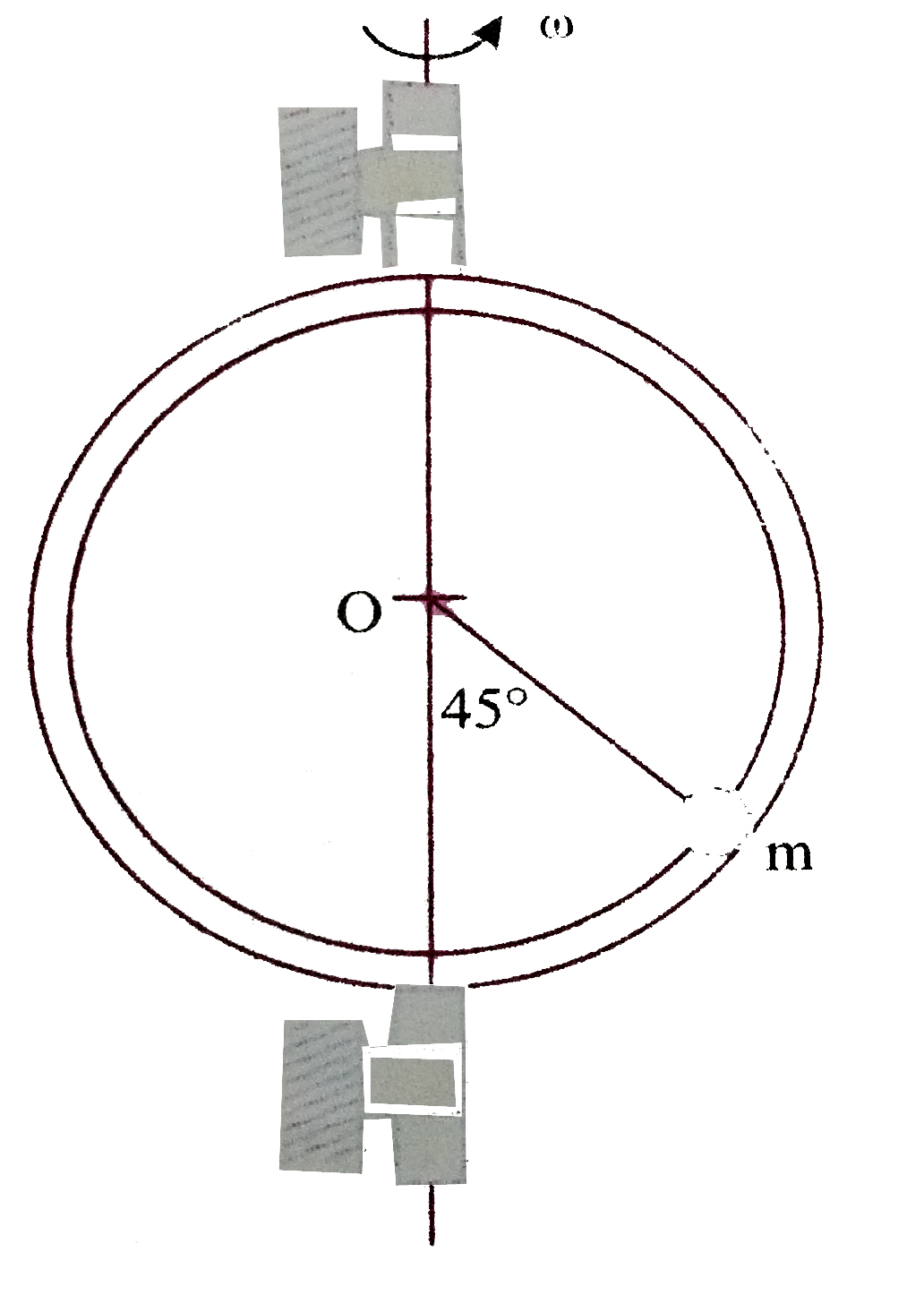
View SolutionA small bead of mass m is carried by a circular hoop having center at O and radius √2m which rotates about a fixed vertical axis. The coefficient of friction between beed and hoop is μ=0.5. The maximum angular speed of the hoop for which the bead does not have relative motion with respect to hoop. (g=10m/s2)
View Solution
