Question

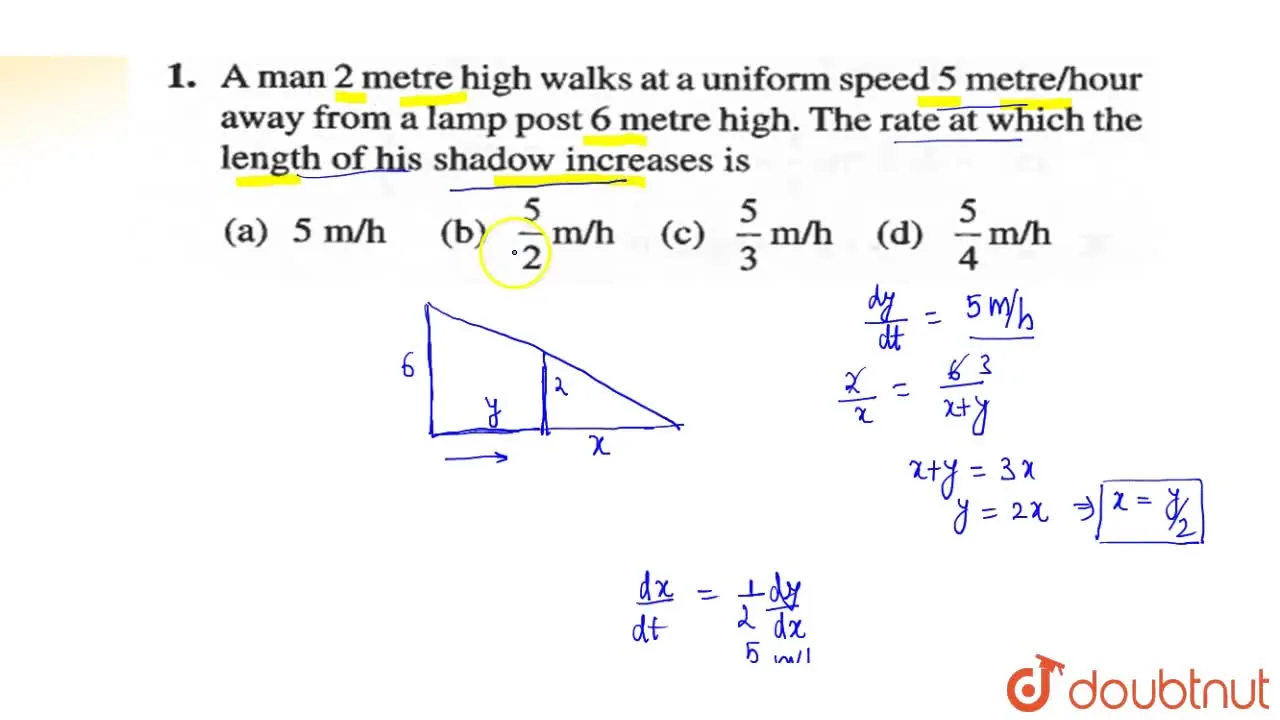
A man 2 metre high walks at a uniform speed 5 metre/hour away from a lamp post 6 metre high. The rate at which the length of his shadow increases is

Options

A. 5m/h
B. `(5)/(2)(m)/(h)`
C. `(5)/(3)(m)/(h)`
D. `(5)/(4)(m)/(s)`

Answer

B

Explanation

To solve the problem, we will use the concept of similar triangles and related rates.

1. Understand the Setup:
- We have a lamp post that is 6 meters high and a man who is 2 meters tall.
- The man walks away from the lamp post at a speed of 5 meters/hour.

2. Define Variables:
- Let \( y \) be the distance of the man from the lamp post.
- Let \( x \) be the length of the shadow of the man.
- The total distance from the lamp post to the tip of the shadow is \( y + x \).

3. Establish Similar Triangles:
- The triangle formed by the lamp post and the tip of the shadow is similar to the triangle formed by the man and the tip of his shadow.
- Using the properties of similar triangles, we can write the proportion:
\( \frac{2}{6} = \frac{x}{y + x} \)
- Simplifying this gives:
\( \frac{1}{3} = \frac{x}{y + x} \)
- Cross-multiplying yields:
\( x = \frac{1}{3}(y + x) \)
- Rearranging gives:
\( 3x = y + x \implies 2x = y \implies y = 2x \)

4. Differentiate with Respect to Time:
- We know that the man walks away from the lamp post at a rate of \( \frac{dy}{dt} = 5 \) meters/hour.
- Now, differentiate the relationship \( y = 2x \) with respect to time \( t \):
\( \frac{dy}{dt} = 2 \frac{dx}{dt} \)

5. Substitute Known Values:
- We substitute \( \frac{dy}{dt} = 5 \) into the differentiated equation:
\( 5 = 2 \frac{dx}{dt} \)
- Solving for \( \frac{dx}{dt} \):
\( \frac{dx}{dt} = \frac{5}{2} \text{ meters/hour} \)

6. Conclusion:
- The rate at which the length of the shadow increases is \( \frac{5}{2} \) meters/hour.

Final Answer:
The rate at which the length of his shadow increases is \( \frac{5}{2} \) meters/hour.

---