A child playing with a long rope ties one end holds the other. The rope is stretched taut along the horizontal. The child shakes the end he is holding, up and down, in a sinusoidal manner with amplitude 10cm and frequency 3 Hz.
Speed of the wave is 15m/s and, at t=0, displacement at the child's end is maximum positive. Assuming that there is no wave reflected from the fixed end, so that the waves in the rope are plane progressive waves, answer the following quetions.
(Also assume that the wave propagates along the positive x-direction.)
Phase difference between the child,s end and a point 2.5m from the child's end will be
π/2
3π/2
π/4
π
The correct Answer is:d
ω=2πf=6πrad/s
and k=ωv=6π15=1.26
y=0.1cos(1.26x−18.8t)
At point 2.5m from child and equation of displacement time to reach 2.5m=T//2<br>Deltaphi=(2piDeltat)/(T)=pi
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Integer
9 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Assertion-Reasoning
6 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICSCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
What type of waves are illustrated by the movement of a rope whose one end is fixed to a pole and the other end is moved up and down?
View SolutionA horse is tied to a rope of length 5 m and the other end of the rope is tied to a pole. Find the displacement and the distance travelled by the horse in the following cases.
When it makes 34 th of the revolution.
View Solution
Knowledge Check
A child playing with a long rope ties one end holds the other. The rope is stretched taut along the horizontal. The child shakes the end he is holding, up and down, in a sinusoidal manner with amplitude 10cm and frequency 3 Hz.
Speed of the wave is 15m/s and, at t=0, displacement at the child's end is maximum positive. Assuming that there is no wave reflected from the fixed end, so that the waves in the rope are plane progressive waves, answer the following quetions.
(Also assume that the wave propagates along the positive x−direction.)
A wave function that describe the wave in the given sutuation isAy=(0.1m)cos[(2rad/s)x−(12.5rad/s)t]By=(0.1m)cos[(1.26rad/s)x−(18.8rad/s)t]Cy=(0.1m)cos[(1.5rad/s)x−(10rad/s)t]Dy=(0.1m)cos[(1.5rad/s)x−(4rad/s)t]A heavy rope is suspended from a rigid support A wave pulse is set up at the lower end, then
AThe pulse will travel with uniform speedBThe pulse will travel with increasing speedCThe pulse will travel with decreasing speedDThe pulse cannot travel through the ropeA stone is tied to one end of a string. On holding the other end, the string is whirled in a horizontal plane with progressively increasing speed. It breaks at some speed because
Athe grvitational forces of the Earth is greater than the tension in string.Bthe required centripetal force is greater than the tension sustained by the string.Cthe required centripetal force is less than the tension in the string.Dthe centripetal force is greatr than the weight of the stone.
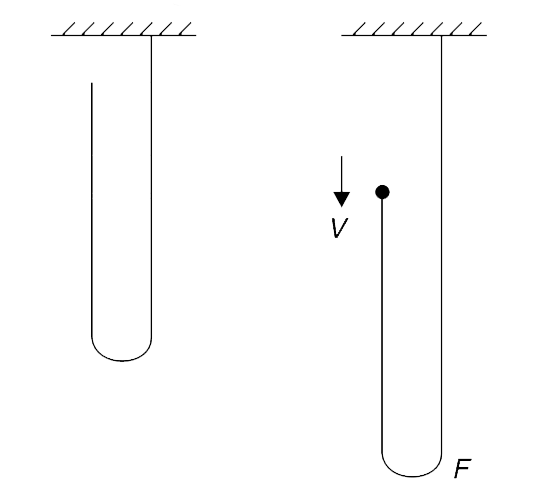
One end of a rope is fixed at a point on the ceiling the other end is held close to the first end so that the rope is folded. The second end is released from this position. Find the speed at which the fold at F is descending at the instant the free end of the rope is going down at speed V.
View SolutionA horse is tied to a rope of length 5 m and the other end of the rope is tied to a pole. Find the displacement and the distance travelled by the horse in the following cases.
When it makes one full revolution.
View SolutionA horse is tied to a rope of length 5 m and the other end of the rope is tied to a pole. Find the displacement and the distance travelled by the horse in the following cases: When it makes one full revolution.
View SolutionA horse is tied to a rope of length 5 m and the other end of the rope is tied to a pole. Find the displacement and the distance travelled by the horse in the following cases:
When it makes 34th of the revolution.
View SolutionThe equation for the fundamental standing sound wave in a tube that is closed at both ends if the tube is 80cm long and speed of the wave is 330m/s is (assume that amplitude of wave at antinode to be s0)
View Solution
