A sinusoidal wave is propagating in negative x-direction in a string stretched along x-axis. A particle of string at x=2 cm is found at its mean position and it is moving in positive y-direction at t=1 s. the amplitude of the wave, the wavelength and the angular frequency of the wave are 0.1m,π/4m and 4πrad/s, respectively.
The instantaneous power transfer through x=2m and t=1.125s is
10J/s
4π/3J/s
2π/3J/s
0
The correct Answer is:d
Time period of oscillation T=2πω=2π4π=12s
Hence at t=1.125s, that is, at T/4 seconds after t=1s, the particle is at extreme position. Hence, instantaneous power at x=2 at t=1.125s is zero.
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Integer
9 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Assertion-Reasoning
6 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICSCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A sinusoidal harmonic wave is propagating along a string stretched along x – axis. A particle on the string at x = 1 m is found to be at its mean position travelling in positive y direction at t=1s. The amplitude, wavelength and frequency of the wave are 0.01m,π2m and 20Hz respectively. Write the equation of the wave if-
(a) it is travelling along negative X direction
(b) it is travelling along positive X direction.
View SolutionA sinusoidal wave having wavelength of 6m propagates along positive x direction on a string. The displacement (y) of a particle at x=2m varies with time (t) as shown in the graph
(a) Write the equation of the wave
(b) Draw y versus x graph for the wave at t=0

View Solution
Knowledge Check
A sinusoidal wave is propagating in negative x-direction in a string stretched along x-axis. A particle of string at x=2 cm is found at its mean position and it is moving in positive y-direction at t=1 s. the amplitude of the wave, the wavelength and the angular frequency of the wave are 0.1m,π/4m and 4πrad/s, respectively.
The equation of the wave isAy=0.1sin(4π(t−1)+8(x−2))By=0.1sin((t−1)−(x−2))Cy=0.1sin(4π(t−1)−8(x−2))Dnone of theseA sinusoidal wave is propagating in negative x-direction in a string stretched along x-axis. A particle of string at x=2 cm is found at its mean position and it is moving in positive y-direction at t=1 s. the amplitude of the wave, the wavelength and the angular frequency of the wave are 0.1m,π/4m and 4πrad/s, respectively.
The speed of particle at x=2m and t=1s isA0.2πm/sB0.6πm/sC0.4πm/sD0A wave travelling in the negative x-direction having displacement along y-direction 2m, wavelength 2π m and frequency of 1/π Hz is represented by
Ay=2sin(x+2t)By=2sin(2πx+2πt)Cy=2sin(10πx+20πt)Dy=2sin(2πx+2πt)
Figure shows a plot of the transverse displacement of the particle of a string at t=0 through which a travelling wave is passing in the positive in the positive x-direction. The wave speed is 20cm/s. Find (a) the amplitude (b) the wavelength (c) the wave number and (d) the frequency of the wave.
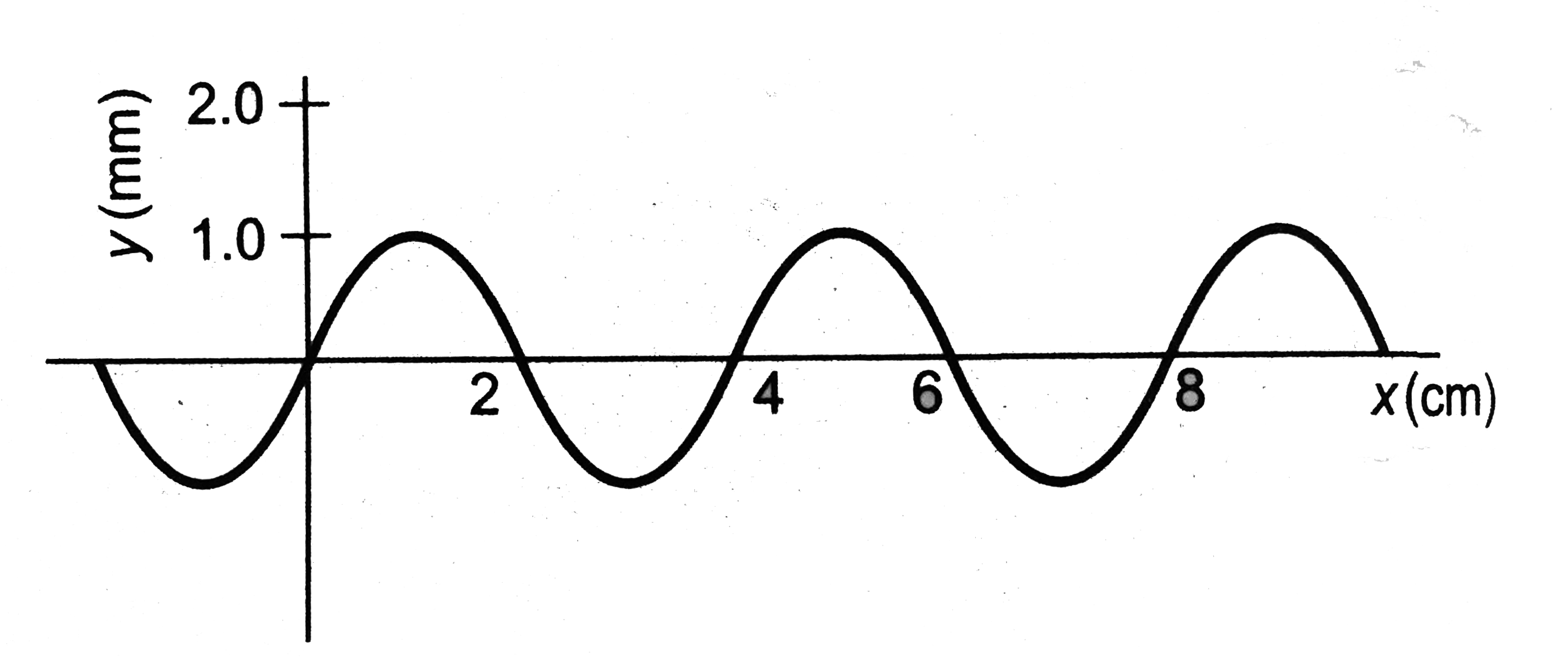
View SolutionThe position of a particle moving along x-axis varies eith time t as x=4t−t2+1. Find the time interval(s) during which the particle is moving along positive x-direction.
View SolutionA sinusoidal wave trsvelling in the positive direction on a stretched string has amplitude 2.0cm, wavelength 1.0cm and velocity 5.0m/s. At x=0 and t=0 it is given that y=0 and ∂y∂t<0. Find the wave function y(x,t).
View SolutionA wave travelling in the +ve x-direction having displacement along y-direction as 1m, wavelength 2π m and frequency of 1/π Hz is represented by
View SolutionA wave travelling in positive x-direction with a=0.2 m/s. Velocity = 360 m/s. and λ = 60 m, then correct expression for the wave is
View Solution
