A block of mass m is suspended from the ceiling of a stationary standig elevator through a spring of spring constant k. Suddenly, the cable breaks and the elevator starts falling freely. Show that the bklock now executes a simple harmonic motion of amplitude in the elevator
the block executes simple harmonic motion with time period
the block excutes simple harmonic motion with amplitude
the block executes simple harmonic motion about its mean position and the mean position is the position When the spring acquires its natural length.
all of the above.
The correct Answer is:D
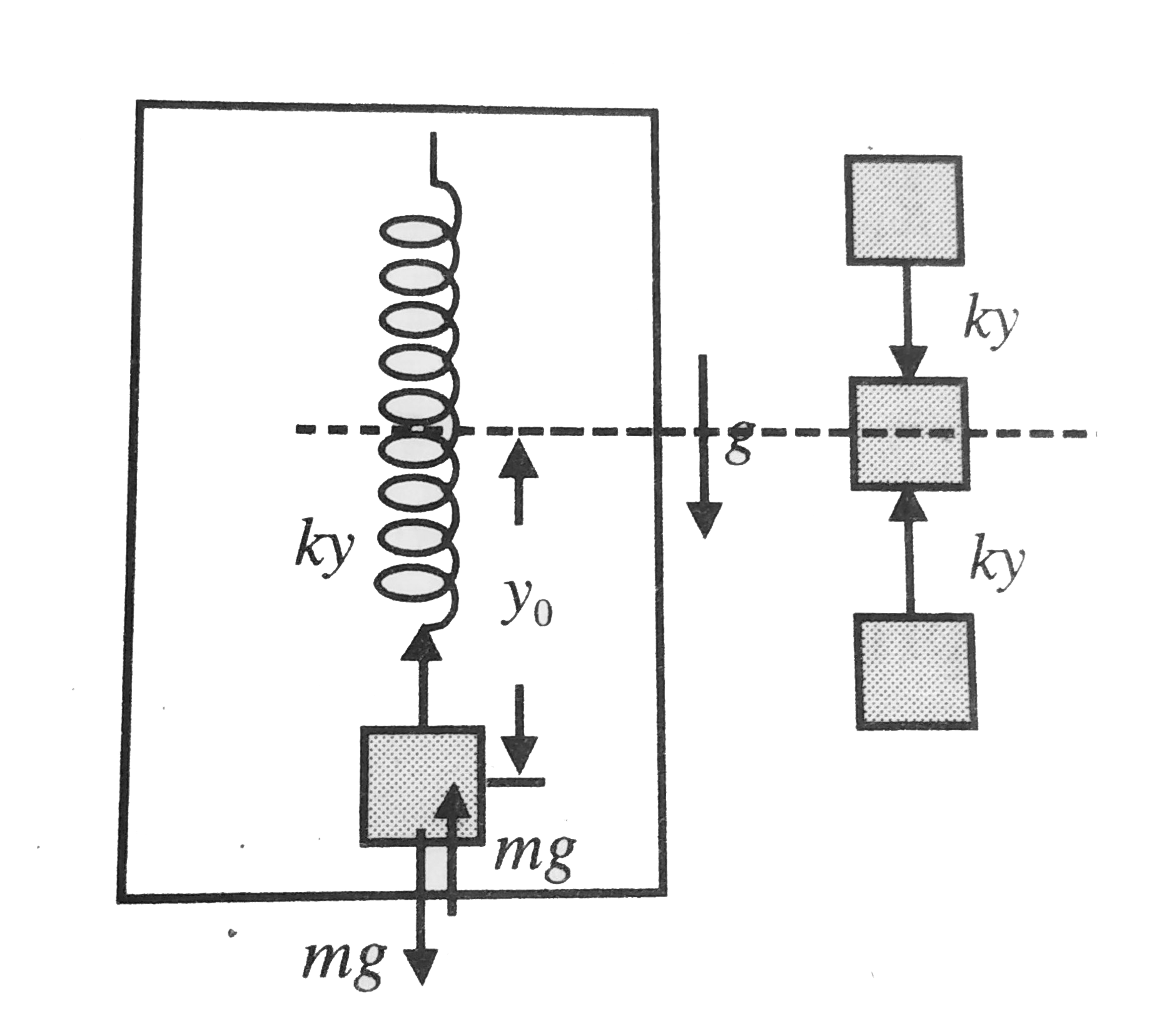
When the elevator is at rest, the elongation in spring is given by or
As the instant the elevator starts falling down with acceleration g, the block is at rest w.r.t elevator and the net force acting on it is in the upward direction w.r.t. lift frame of reference. Due to this force, the block moves up and as a result elongation in the spring decreases, and the force experienced by the block becomes zero when spring and stops momentarily till compression in the spring becomes .
Hence the block will always have net force towards relaxed position of spring and the block will perform simple harmonic notion with time period and with amplitude
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct
35 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Assertion Reasoning
6 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Subjective
21 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
A block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
View SolutionA block of 2 kg is suspended from the ceiling trhough a massless spring of spring constant k=100 N/m. What is the elongation of the spring? If another 1 kg is dded to the block, what would be the further eleongation?
View Solution
Knowledge Check
A solid ball of mass m is made to fail from a height H on a pan suspended through a spring of spring constant K as shown in figure. If the does not rebound and the pan is massless, then amplitude of oscillation is
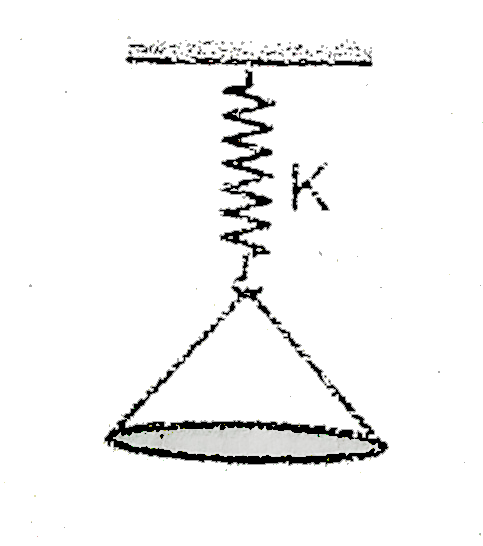 AmgKBmgk(1+2HKmg)1/2CmgK+(2HKmg)1/2DmgK[1+(1+2HKmg)1/2]
AmgKBmgk(1+2HKmg)1/2CmgK+(2HKmg)1/2DmgK[1+(1+2HKmg)1/2]A weight is suspended from the ceiling of a lift by a spring balance. When the lift is stationary the spring balance reads W . If the lift suddenly falls freely under gravity, the reading on the spring balance will be
AWB2WCW/2D0A body of mass m falls from a height h onto the pan of a spring balance. The masses of the pan and spring are negligible. The force constant of the spring is k. The body sticks to the pan and oscillates simple harmonically. The amplitude of oscillation is
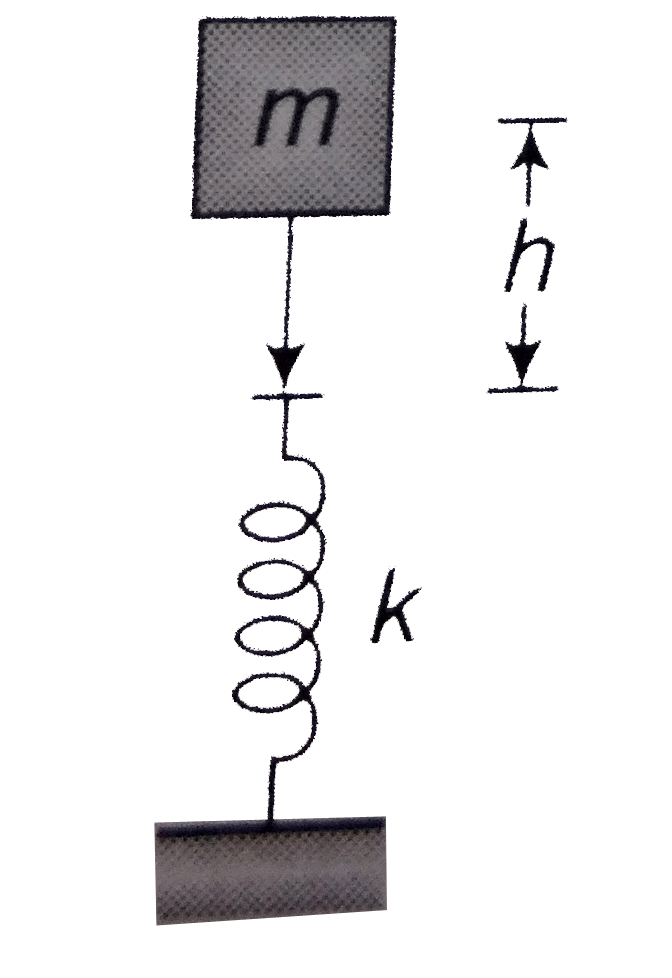 AmgkBmgk√1+2hkmgCmgk+mgk√1+2hkmgDNone of these
AmgkBmgk√1+2hkmgCmgk+mgk√1+2hkmgDNone of these
A block of mass M is lying on a frictionless horizontal surface and it connected to a spring of spring constant k, whose other end is fixed to a wall, system executes simple harmonic motion of amplitude A0, when the block is passing through its equilibrium positions, an object of mass m is put on it and the two move together with a new time period T and amplitude A. Find the value of T and A in terms of given constants.
View SolutionA block of mass m is connected to another .block of mass M by a massless spring of spring constant k. A constant force f starts action as shown in figure, then:

View SolutionA body of mass m is suspended from a spring fixed to the ceiling of an elevator car. The stiffness of the spring is x. At the moment t=0 the car starts going up with an accelerationw. Neglecting the mass of the spring , find the law of motion y(t0 of the body relative to the elevator car if y(0)−0 and y(0)=0. Consider the following two cases :
(a) w=const,
(b) w=αt, where αis a constant.
View SolutionTwo springs of constants k1 and k2 have equal maximum velocities, when executing simple harmonic motion. The ratio of their amplitudes (masses are equal) will be
View SolutionThe angular frequency of a spring block system is ω0. This system is suspended from the ceiling of an elevator moving downwards with a constant speed V0. Ther block is at rest relative to the elevator. Lift is suddenly stopped. Assuming the downwards as a positive direction, choose the wrong statement:
View Solution
