Question

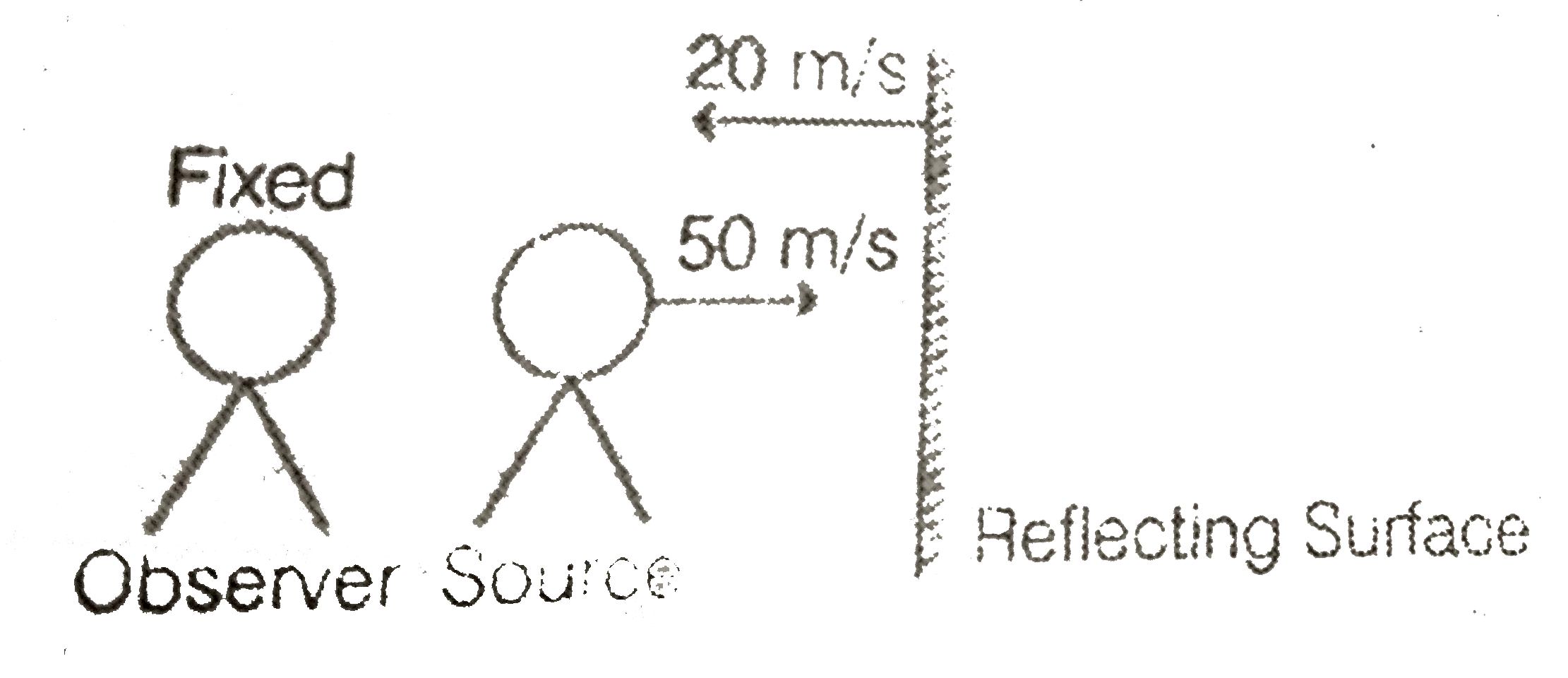
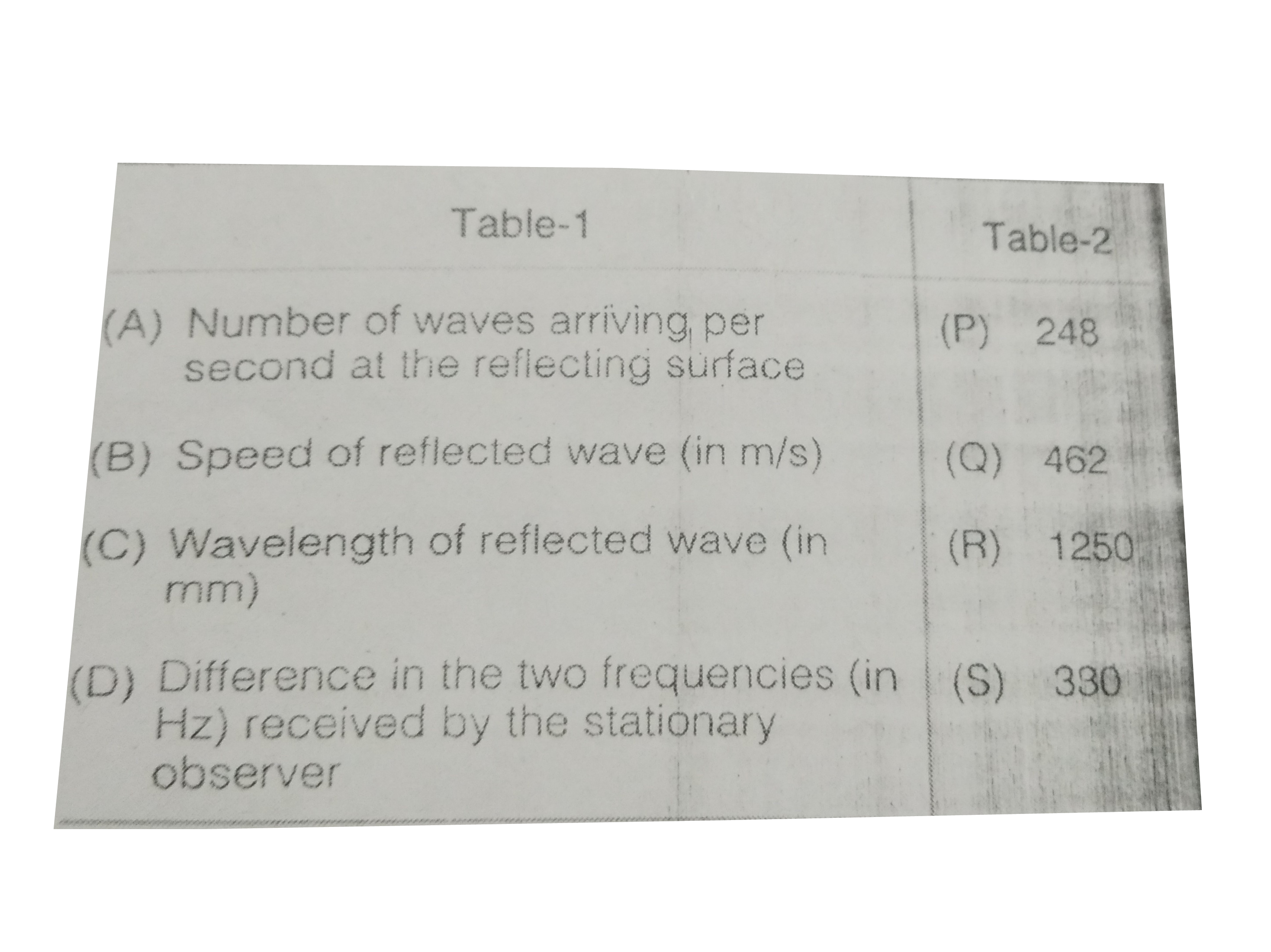
A source of sound of frequency 1000 Hz moves to the right with a speef of ${\displaystyle 32\frac{m}{s}}$ relative to the ground. To its right there is a reflecting surface moving to the left with a speed of ${\displaystyle 64\frac{m}{s}}$ relative to the ground. Take the speed of ${\displaystyle 64\frac{m}{s}}$. Relative to the ground. Take the speed of sound in air to be ${\displaystyle 332\frac{m}{s}}$ and find
(a) The wavelength of the sound emitted in air by the source,
(b) the number of waves per second arriving at the reflecting surface, (c ) The speed of the reflected waves and
(d) The wavelength of the reflected waves.

Explanation

(a) Due to motion of the source the wavelength (and hence the frequency) is actually changed from `lamda` and `lamda'` such that if `n=` actual frequency.
`lamda'=(c-v_S)/(n)=(332-32)/(1000)=0.3m`
(b) The number of waves arriving at the reflecting surface is the same as the number of waves received by an observer moving towards the source. This is given by the apparent frequency.
`n'=(c-v_0)/(c-v_S)xxn`
`=(332-(-64))/(332-32)xx1000=1320Hz`
(c ) Same as that of the incident wave because the speed of wave depends only on the characteristics of the medium. therefore, speed of the reflected wave`=332ms^-1`
(d) Now reflector will act as a source of frequency 1320 Hz. So wavelength emitted by this:
`lamda'=(v-v_(ref))/(1320)=(332-64)/(1320)=0.2m`