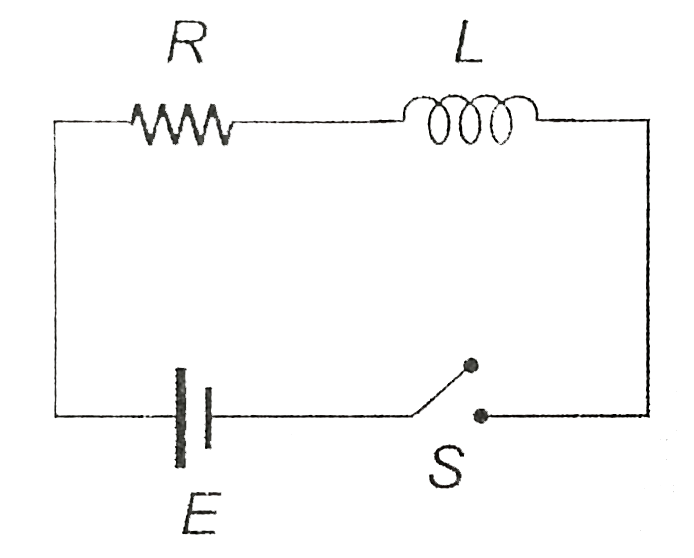Consider the RL circuit in Fig. When the switch is closed in position 1 and opens in position 2, electrical work must be performed on the inductor and on the resistor. The energy stored in the inductor is for the resistor energy appears as heat.
a. What is the ratio of PL/PR of the rate at which energy is stored in the inductor to the rate at which energy is dissipated in the resistor?
b. Express the ratio PL/PR as a function of time.
c. If the time constant of circuit is t, what is the time at which PL=PR?
Video Solution
Text Solution
The anergy stored in the inductor is UL=12LI2
Power PL=dUdt=ddt(12LI2)=LIdIdt(i)
Power of resitor PR=I2R (ii)
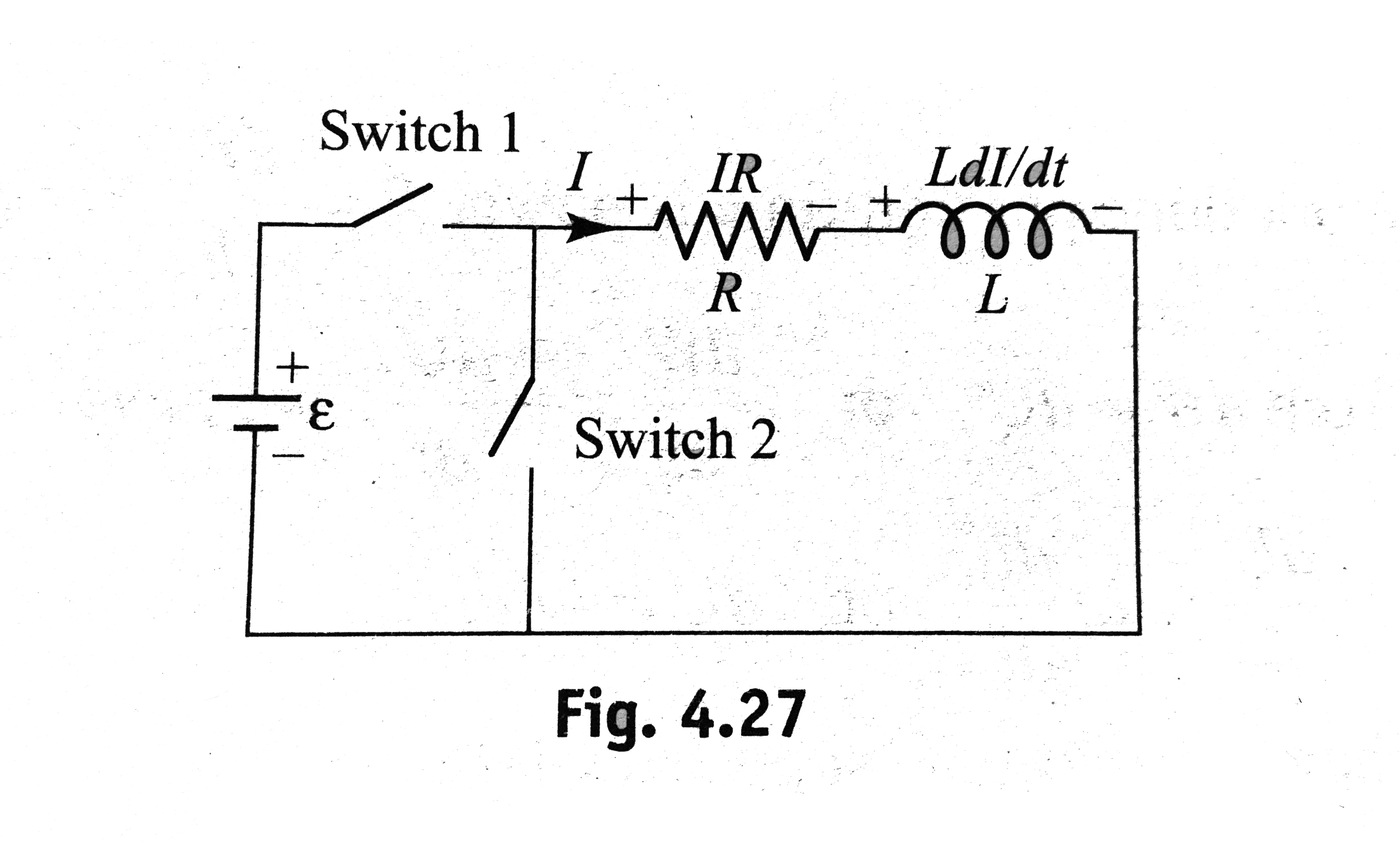
So the ratio PLPR=LIdI/dtI2R=τ(dI/dt)I (iii)
b. As I=ER[1−e−(t/τ)] (iv)
dIdt=ddt[ER(1−e−(t/τ))]=ERτe(−(t/τ) (v)
Inserting the value of dI/dt and I in Eq.(iii), we have
PLPR=τ=ERτe−t/τER(1−e−t/τ)=e−(t/τ)1−e−(t/τ)=1e(t/τ)−1 (vi)
When t=0, this ratio tends to infinity, due to large initial value of dI/dt and small initial value of I. When t tends to infinity, after many time constants, the current tends to zero. As a result, vanishes and the ratio goes to zero.
c. From Eq.(vi),PL=PR when 1e(t/τ)−1=1 which on
simplification yields e(t/τ)=2,tτ=In2⇒t=0.693τ
|
Topper's Solved these Questions
- INDUCTANCEBook:CENGAGE PHYSICSChapter:INDUCTANCEExercise:Solved Examples
Explore 3 Videos
- INDUCTANCEBook:CENGAGE PHYSICSChapter:INDUCTANCEExercise:Exercise 4.1
Explore 24 Videos
- HEATING EFFECT OF CURRENTBook:CENGAGE PHYSICSChapter:HEATING EFFECT OF CURRENTExercise:Thermal Power in Resistance Connected in Circuit
Explore 28 Videos
- KINETIC THEORYBook:CENGAGE PHYSICSChapter:KINETIC THEORYExercise:Question Bank
Explore 31 Videos
Similar Questions
Knowledge Check
An inductor stores the energy in
A1. its electric fieldB2. its coilC3. its magnetic fieldD4. both in electric and magnetic fieldsIn an inductor of inductance L=100mH, a current of I=10A is flowing. The energy stored in the inductor is
A5JB10JC100JD1000JAn inductor may store energy in
Aits electric fieldBits coilsCits magentic fieldDBoth in electric and magnatic field
5A 50 mH inductor is in series with a 10 2 resistor and a battery with an emf oj 25 V. At t = 0 the switch is closed. Find
Q(c) the rate at which energy is stored in the inductor,
View SolutionEnergy Stored in an Indutor || Inductor in a Circuit
View SolutionIn the steady state of the circuit shown in the figure the ratio of energy stored in the inductor to th energy stored in the capacitor is 10000.
View SolutionReferring to the previous illustration find the energy stored in the inductor when the current l is dropped to a value of 5A .
View SolutionAn inductor may store energy in
View Solution