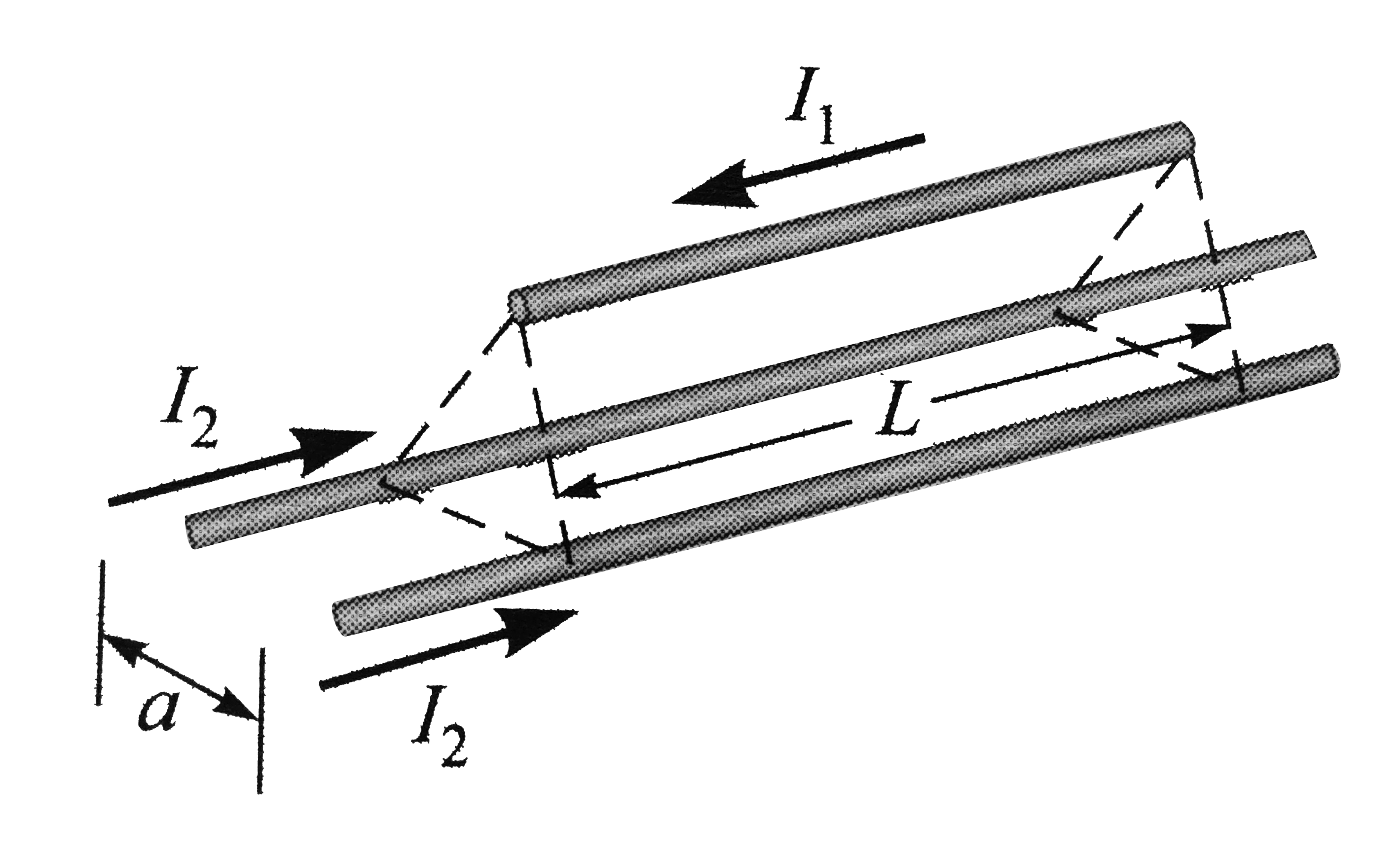
Two infinetely long, parallel wires are lying on the ground at distance a=1cm apart as shown in Fig. A third wire, of length L=10m and mass 400g, carries a current of I1=100A and is levitated above the first two wires, at a horizontal position midway between them. The infinitely long wires carry equal currents I1 in the same directions, but in the direction opposite that in the leviated wire. What current must the infinately long wires carry so that the three wires form an equilateral triangle?
Video Solution
Text Solution
Since the current in the short wire is opposite to those in
the long wires, the short wire is repelled from both of the others.
Imaging the currents in the long wires in Fig are increased.
The repulsive force becomes stronger, and the levitated wire rises to the point at which the wire is once again levitated in
equilibrium at a higher position. Figure shows the desired
situation with the three wires forming an equilateral triangle.
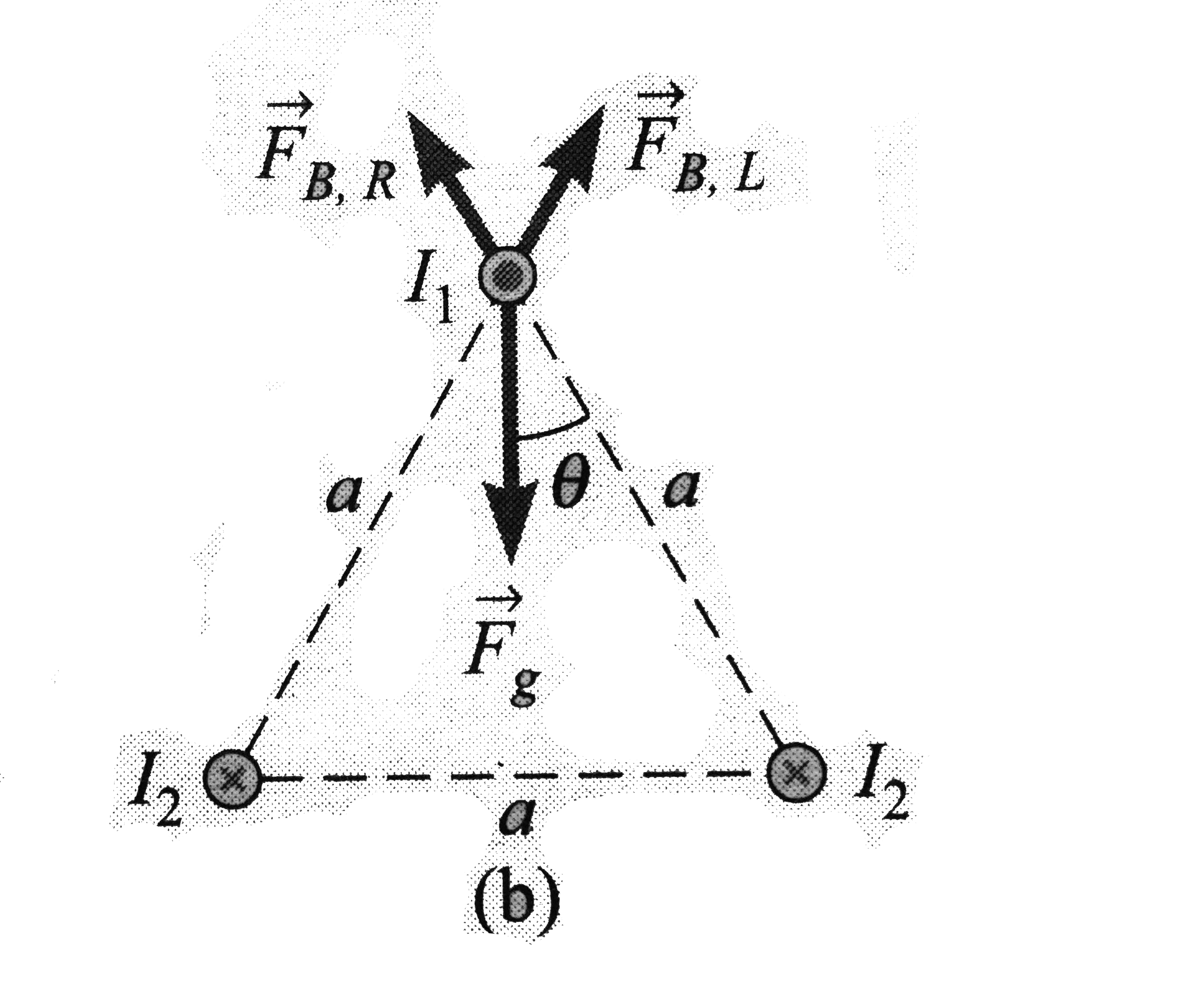
Because the levitated wire is subjected to forces but does not
The horizontal components of the magnetic forces on the levitated
wire cancel. The vertical components are both positive and added
Fig. and in the plane of the page.
The total magnetic force in the upward direction on the levitated
wire:
→FB=2(μ0I1I22πa)cosθˆk=(μ0I1I22πa)lcosθˆk
The gravitational force on the levitaed wire :
→Fg=−mgˆk
Apply the particle in equilibrium model by adding the forces and
setting the net force equal to zero:
Σ→F=→FB+Fg=(μ0I1I2πa)lcosθˆk−mgˆk=0
Solve for the current in the wires on the ground:
I2=mgπaμ0I1lcosθ
Substituting numetical values:
I2=(0.400kg)(10ms−2)π(0.0100m)(4π×10−7T.mA−2)(100A)(10m)cos30∘=113A
|
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Solved Example
20 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Concept Exercise 2.1
35 Videos
- SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - CENGAGE PHYSICSCHAPTER - SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - QUESTION BANK
12 Videos
- THERMAL PROPERTIES OF MATTERBOOK - CENGAGE PHYSICSCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Question Bank
40 Videos
Similar Questions
Knowledge Check
Two infinitely long parallel wires carry equal currents in the same direction .The magnetic field at a mind point in between the two wires is
ASquare of the magnetic field produced due to each of the wiresBhalf of the magnetic field produced due to each of the wiresCtwice the magnetic field produced due to each of the wiresDzeroTwo infinitely long parallel wires carry equal current in same direction. The magnetic field at a mid point in between the two wires is
ATwice the magnetic field produced due to each of the wiresBHalf of the magnetic field produced due to each of the wiresCSquare of the magnetic field produced due to each of the wiresDZeroTwo free parallel wires carrying currents in opposite directions
Ado not affect each otherBattract each otherCrepel each otherDnone of these
Two free parallel wires carrying currents in opposite directions
View SolutionTwo free parallel wires carrying currents in opposite directions
View SolutionTwo infinitely long wires each carrying current I along the same direction are made into the geometry as shown in the figure. The magnetic field at the point P is

View SolutionAn infinitely long straight wire is carrying a current I1. Adjacent to it there is another equilateral triangular wire having current I2. Choose the wrong options
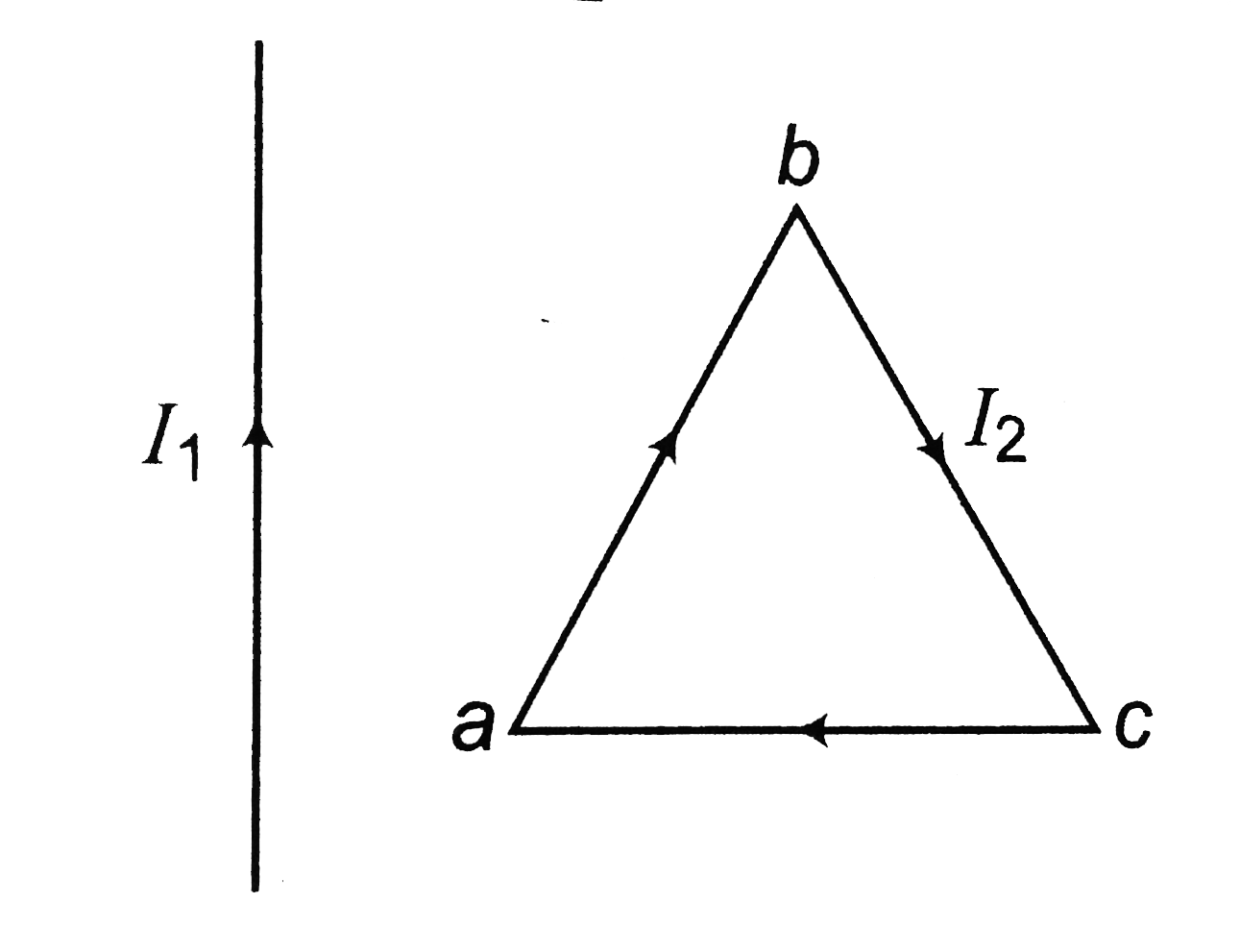
View SolutionThe direction of magnetic field at a point due to an infinitely long wire carrying current is
View Solution
