A gas of hydrogen - like ion is perpendicular in such a way that ions are only in the ground state and the first excite state. A monochromatic light of wavelength 1216Å is absorbed by the ions. The ions are lifted to higher excited state and emit emit radiation of six wavelength , some higher and some lower than the incident wavelength. Find the principal quantum number of the excited state identify the nuclear charge on the ions . Calculate the values of the maximum and minimum wavelengths.
The energy state of hydrogen-like atom is given by
En=−Bn2
where B=Z2Rhc.
The emission of six wavelength implies that the higher excited state corresponds to quantum number n=4 . As some wavelength are lower and some wavelength are higher , it implies that the initial excited state is n=2 . Thus the principal quantum number of excited states are n=,n=3,n=4.
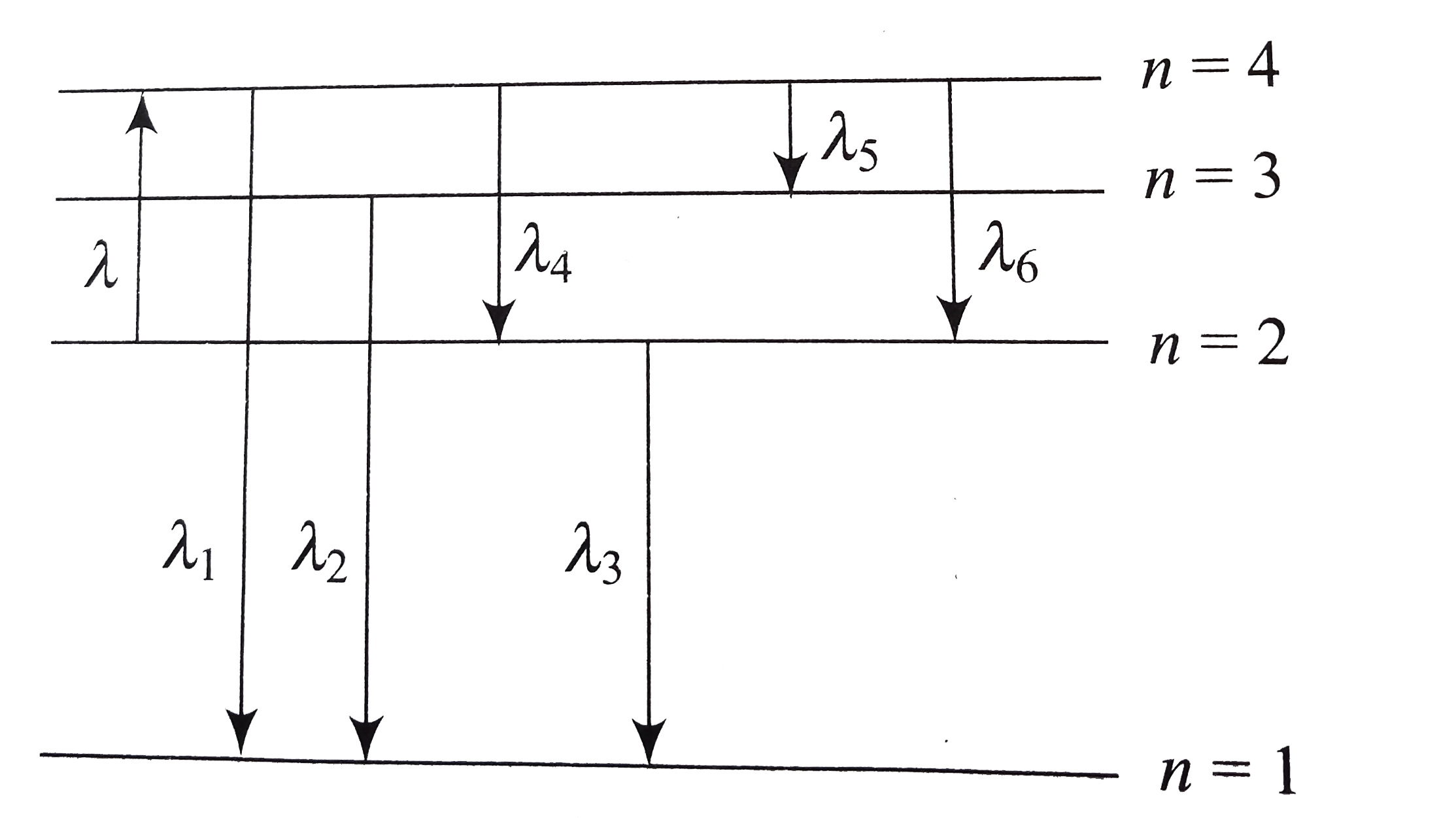
∴hcλ=B(122−142)⇒hcλ=3B16
∴B=1.6×6.62×10−34×3×1083×(1216×10−10)
=8.71×10−18J
If Z is atimic number of necleus, then B=Z2Rhc
∴Z2=BRhc=8.17×10−18J13.6×1.6×10−19=4
∴Z=2
The maximum wavelength of emitted redition corresponds to transition n=4→n=3 and is given by
hcλmax=B(132−142)
⇒λmax=144hc7B=144×6.62×10−34×3×1087×8.71×10−18
=4.69×10−7m
The minimum wavelength of emitted rediction corresponds to transition n=4→n=1 and is given by
hcλmax=B(112−142)
⇒λmax=16hc15B=16×6.62×10−34×3×10815×8.71×10−18
=0.2×10−7m
Topper's Solved these Questions
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICSCHAPTER - ATOMIC PHYSICSEXERCISE - Exercise 4.1
15 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICSCHAPTER - ATOMIC PHYSICSEXERCISE - Exercise 4.2
12 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICSCHAPTER - ATOMIC PHYSICSEXERCISE - ddp.4.3
15 Videos
- ALTERNATING CURRENTBOOK - CENGAGE PHYSICSCHAPTER - ALTERNATING CURRENTEXERCISE - QUESTION BANK
65 Videos
- ATOMSBOOK - CENGAGE PHYSICSCHAPTER - ATOMSEXERCISE - QUESTION BANK
40 Videos
Similar Questions
An excited state of H atom emits a photon of wavelength λ and returns in the ground state. The principal quantum number of excited state is given by:
View SolutionIn a hydrogen atom,electron moves from second excited state to first excited state and then from first excited state to ground state.The ratio of wavelengths is
View Solution
Knowledge Check
Hydrogen atom from excited state comes to the ground state by emitting a photon of wavelength λ. If R is the Rydberg constant, then the principal quantum number n of the excited state is
A√λRλR−1B√λλR−1C√λR2λR−1D√λRλ−1An excited hydrogen atom emits a photon of wavelength λ in returning to the ground state. If R is the Rydberg constant, then the quantum number n of the excited state is
A√λRB√λR−1C√λ(λR−1)D√λRλR−1An excited hydrogen atom emits a photon of wavelength λ in returning to the ground state. If 'R' is the Rydberg's constant, then the quantum number 'n' of the excited state is:
A√λRB√λR−1C√λRλR−1D√λR(λR−1)
A He+ ion in the excited state, on transition to the ground state, emits two photons in succession with wavelengths 108.5 nm and 30.4 nm. What is the quantum number n corresponding to the excited state of He+ ion ?
View SolutionFind the quantum number n corresponding to nth excited state of He++ ion if on transition to the ground state the ion emits two photons in succession with wavelength 108.5 nm and 30.4 nm. The ionization energy of the hydrogen atom is 13.6 eV.
View SolutionAn excited hydrogen atom returns to the ground state . The wavelength of emittted photon is λ The principal quantium number fo the excited state will be :
View SolutionThe value of wavelength radiation emitted by an H-atom, if atom is excited to state with principal quantum number four is :
View SolutionMonochromatic radiation of specific wavelength is incident on H-atoms in ground state. H-atoms absorb energy and emit subsequently radiations of six different wavelength. Find wavelength of incident radiations:
View Solution