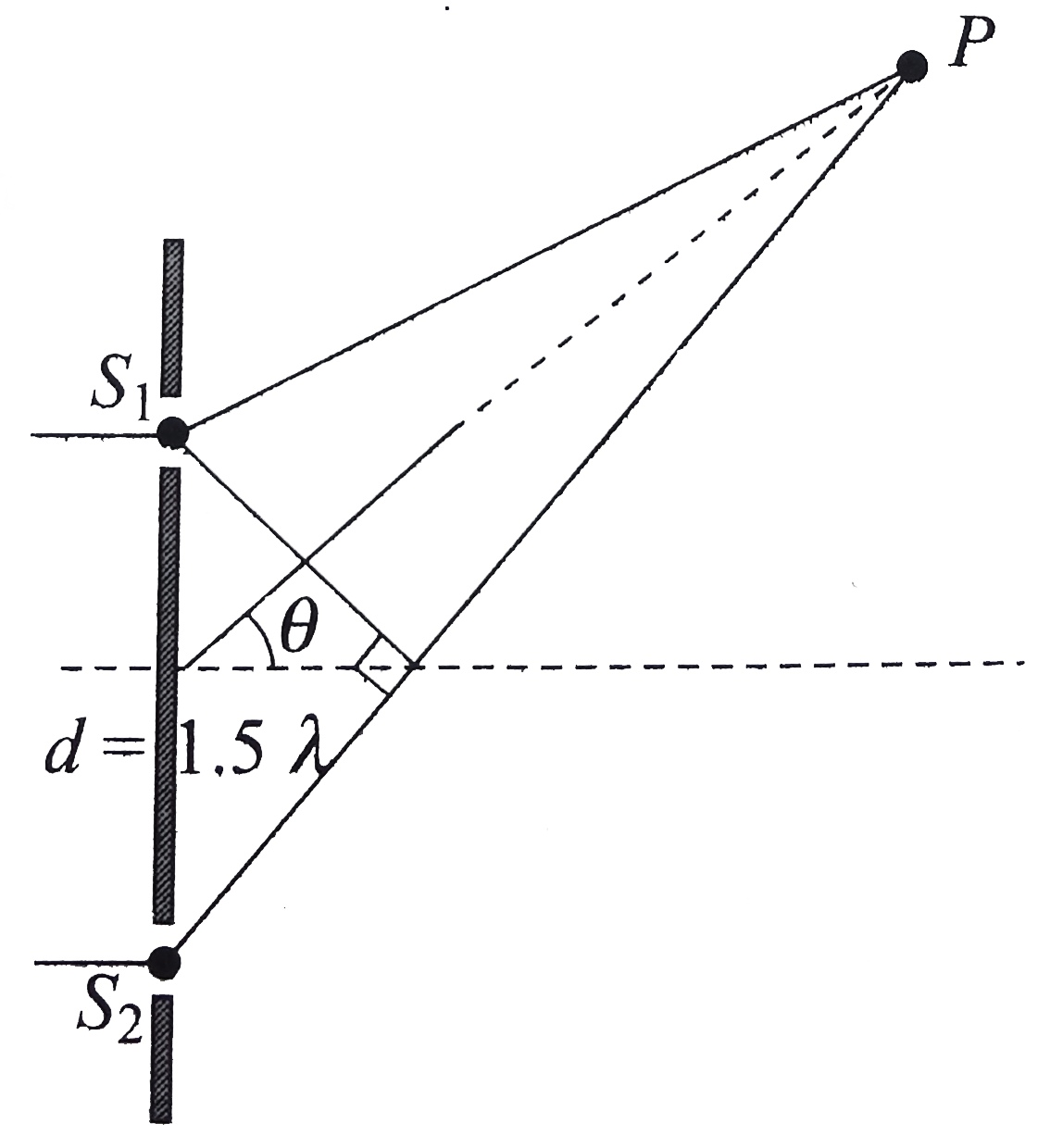
Figure shows two coherent sources S1 and S2 emitting wavelength λ. The separation S1S2=1.5λ and S1 is ahead in phase by π/2 relative to S2 Then the maxima occur in direction θ given by sin−1 of
(i) 0 (ii) 1/2 (iii) −1/6 (iv) −5/6
Correct option are
(ii), (iii), and (iv)
(i), (ii), and (iii)
(i), (iii), and (iv)
All the above
The correct Answer is:a
δ= phase differene between the waves from S1 and S2 at P=
π2−2π2(dsinθ)
For maximum intensity at P, δ=nx where n=0,±1,±2......
∴2π2(1.5λsinθ)π2=nπ
⇒n−12=3sinθ
⇒sinθ=⎛⎝n−123⎞⎠
For n=0, sinθ=−16
For n=±1 sinθ=16,−12
For n=±2, sinθ=12,−56
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Multiple Correct
8 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Assertion- Reasoning
13 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Subjective
11 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - QUESTION BANK
34 Videos
- WAVESBOOK - CENGAGE PHYSICSCHAPTER - WAVESEXERCISE - QUESTION BANK
38 Videos
Similar Questions
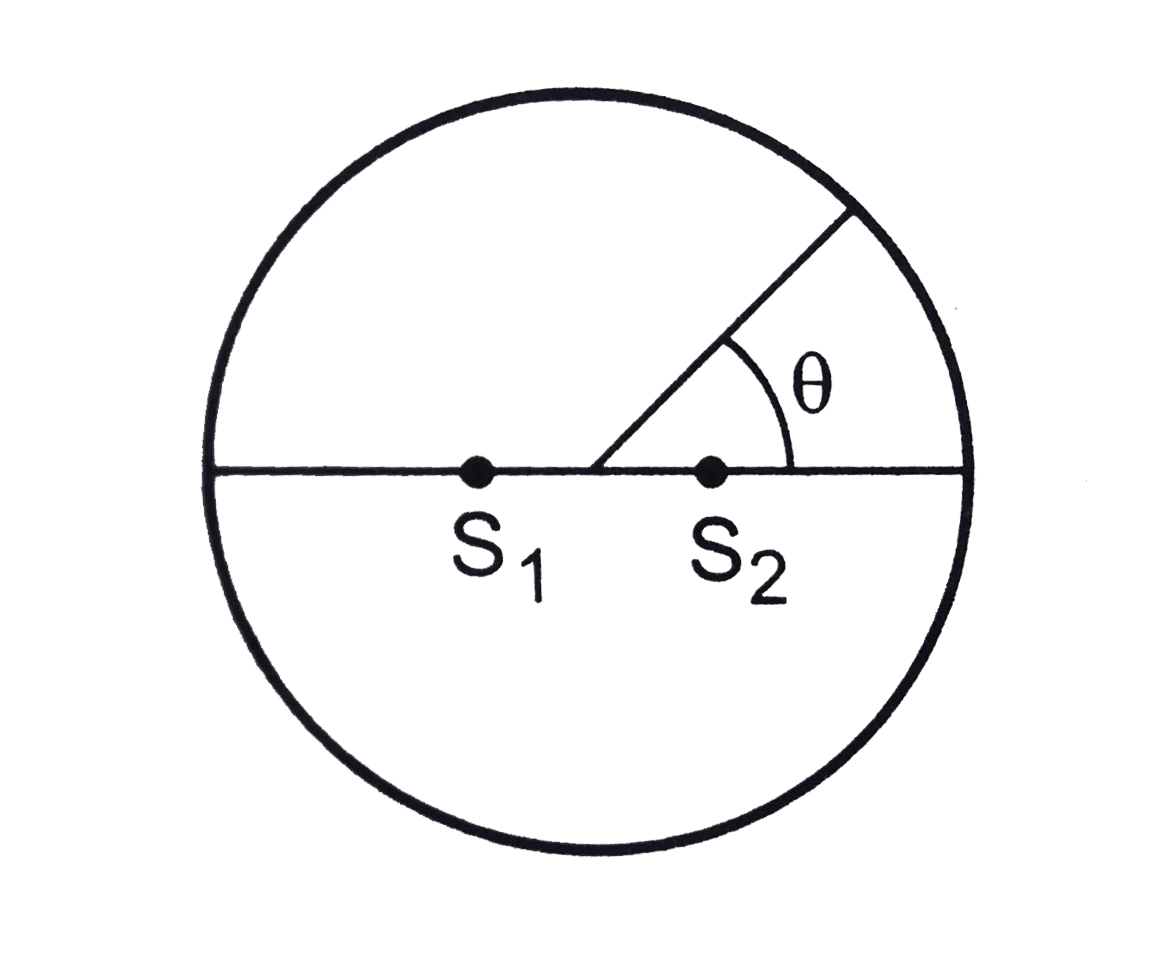
Figure shows two coherent sources S1 and S2 which emit sound of wavelength λ in phase. The separation between the sources is 3λ. A circular wire of large radius is placed in such a way that S1S2 lies in its plane and the middle point of S1S2 is at the centre of the wire. Find the angular positions θ, on the wire for which constructive interference takes place.

View SolutionTwo coherent point sources S1 and S2 vibrating in phase emit light of wavelength λ. The separation between the sources is 2λ. Consider a line passing through S1 and perpendicular to line S1S2. Find the position of farthest and nearest minima.
 .
.
View Solution
Knowledge Check
Two identical light sources S1 and S2 emit light of same wavelength λ. These light rays will exhibit interference if
A(a) Their phase differences remain constantB(b) Their phases are distributed randomlyC(c) Their light intensities remain constantD(d) Their light intensities change randomlyTwo coherent point sources S1 and S2 vibrating in phase emit light of wavelength λ. The separation between the sources is 2λ. Consider a line passing through S2 and perpendicular to line S1S2. Find the position of farthest and nearest minima.
 .A7λ12B15λ4Cλ2D3λ4
.A7λ12B15λ4Cλ2D3λ4Two monochromatic coherent point sources S1 and S2 are separated by a distance L. Each sources emits light of wavelength λ, where L>>λ. The line S1S2 when extended meets a screen perpendicular to it at point A. Then
Athe interference fringe on the screen are straight lines shapeBthe interference fringes on the screen are strainght lines perpendicular to the line S1S2ACpoint A is an intensity maxima if L=nλDpoint A is always an intensity maxima for any separation L
Figure shows two coherent sources S1andS2which emit sound of wavelength λ in phase. The separation between the sources is 3λ. A circular wire at large radius is placed in such a way that S1S2 lies in its plane and the middle point of S1S2, is at the centre of the wire. Find the angular positions 0 on the wire for which constructive interference takes place.
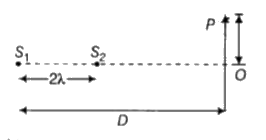
View SolutionTwo coherent point source S1 and S2 vibrating in phase emit light of wavelength λ. The separation between them is 2λ as shown in figure. The first bright fringe is formed at 'P' due to interference on a screen placed at distance 'D' from S1(D>>λ), then OP is

1) √3D,2)1.5D,3)√2D,4)2D
View SolutionChoose the most appropriate option.
Two coherent point sources S1andS2 vibrating in phase emit light of wavelength λ. The separation between them is 2λ. The light is collected ona screen placed at a distance D>>λ from the slit S1 as shown. The minimum distance, so that intensity at P is equal to intensity at O.
View SolutionTwo waves of equal amplitude a from two coherent sources (S1&S2) interfere at a point R such that S2R−S1R=1.5λThe intensity at point R Would be
View SolutionIn the figure , the intensity of waves arriving at D from two coherent soucrces s1ands2isI0. The wavelength of the wave is λ=4m . Resultant intensity at D will be

View Solution
