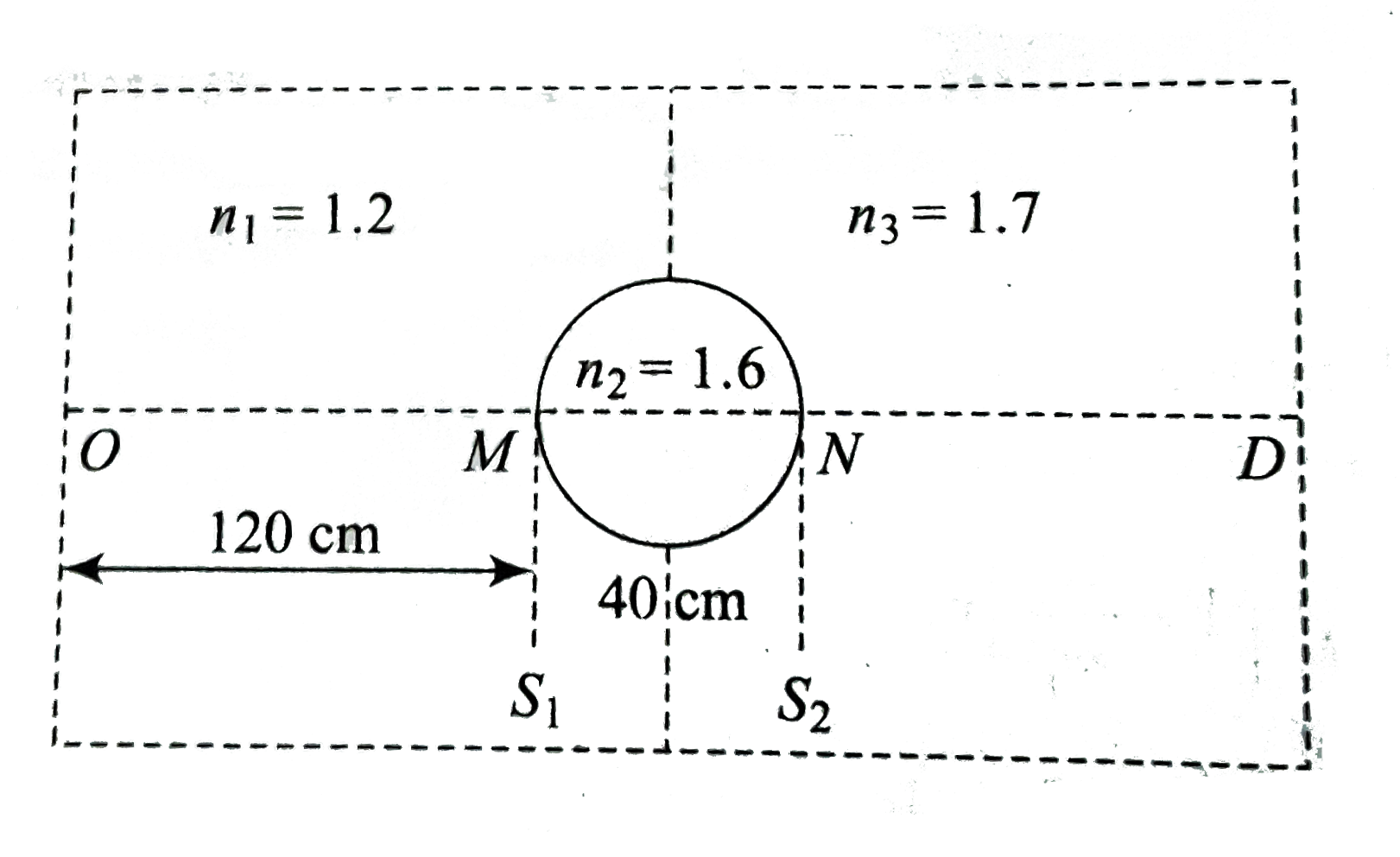
A tranparent sphere of radius 20cm and refractive index 1.6 is fixed in a hole of the partition separating the two media: A(refractive index $n_{1} = 1.2)$ and B ( refractive index $n_{3} = 1.7)$ . A luminous point object is placed 120cm from the surface of the sphere iin medium A. it is viewed from D in medium B in a direction normal to the sphere. Find the position of the image formed by the rays, from point N.
$A tranparent sphere of radius 20cm and refractive index 1.6 is fixed in a hole of the partition separating the two media: A(refractive index n(1)=1.2) and B ( refractive index n(3)=1.7). A luminous point object is placed 120cm from the surface of the sphere iin medium A. it is viewed from D in medium B in a direction normal to the sphere. Find the position of the image formed by the rays, from point N.$

A

304cm, left side of N

B

175 cm, right side of N

C

204 cm, right side of N

D

220cm, left side of N

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

We will have to consider two refractions: first at surface $S_{1}$ and the second at surface $S_{1}$ .
For refraction at surface $S_{1}$ :
$\frac{1.6}{v_{1}} - \frac{1.2}{(- 120)} = \frac{(1.6 - 1.2)}{20}, υ_{1} = + 160 c m$
The positive sign implies that first image is formed to the right fo $S_{1}$ .
For refraction at surface $S_{2}$ :
The first image is object for refraction at second surface,
$υ_{2} = + (160 - 40) c m$
$\frac{1.7}{υ} - \frac{1.6}{+ (160 - 40)} = \frac{1.6 - 1.7}{(- 20)}, υ_{2} = + 204 c m$
The final image is formed at 204cm from vertex and on the right side.

|

Updated on:21/07/2023

Class 12 PHYSICS RAY OPTICS AND OPTICAL INSTRUMENTS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
When a lens of refractive index $n_{1}$ is placed in a liquid of refractive index $n_{2}$ , the lens looks to be disappeared only if
A $n_{1} = \frac{n_{2}}{2}$
B $n_{1} = \frac{3}{4} n_{2}$
C $n_{1} = n_{2}$
D $n_{1} = \frac{5}{4} n_{2}$
Question 2 - Select One
The speed of light in a medium of refractive index 1.5 is ________.
A $1.5 \times 10^{8} m / s$
B $3.0 \times 10^{8} m / s$
C $2.0 \times 10^{8} m / s$
D $1.2 \times 10^{8} m / s$
Question 3 - Select One
Light travels from medium 1 (refractive index = $n_{1}$ ) to medium 2 (refractive index = $n_{2}$ ). If it is found that deviation d=r-i. We can say that
A $n_{1} = n_{2}$
B $n_{1} < n_{2}$
C $n_{1} > n_{2}$
Dnone of these

CENGAGE PHYSICS-GEOMETRICAL OPTICS-Single Correct

Home
Ask Doubt
Profile