Question

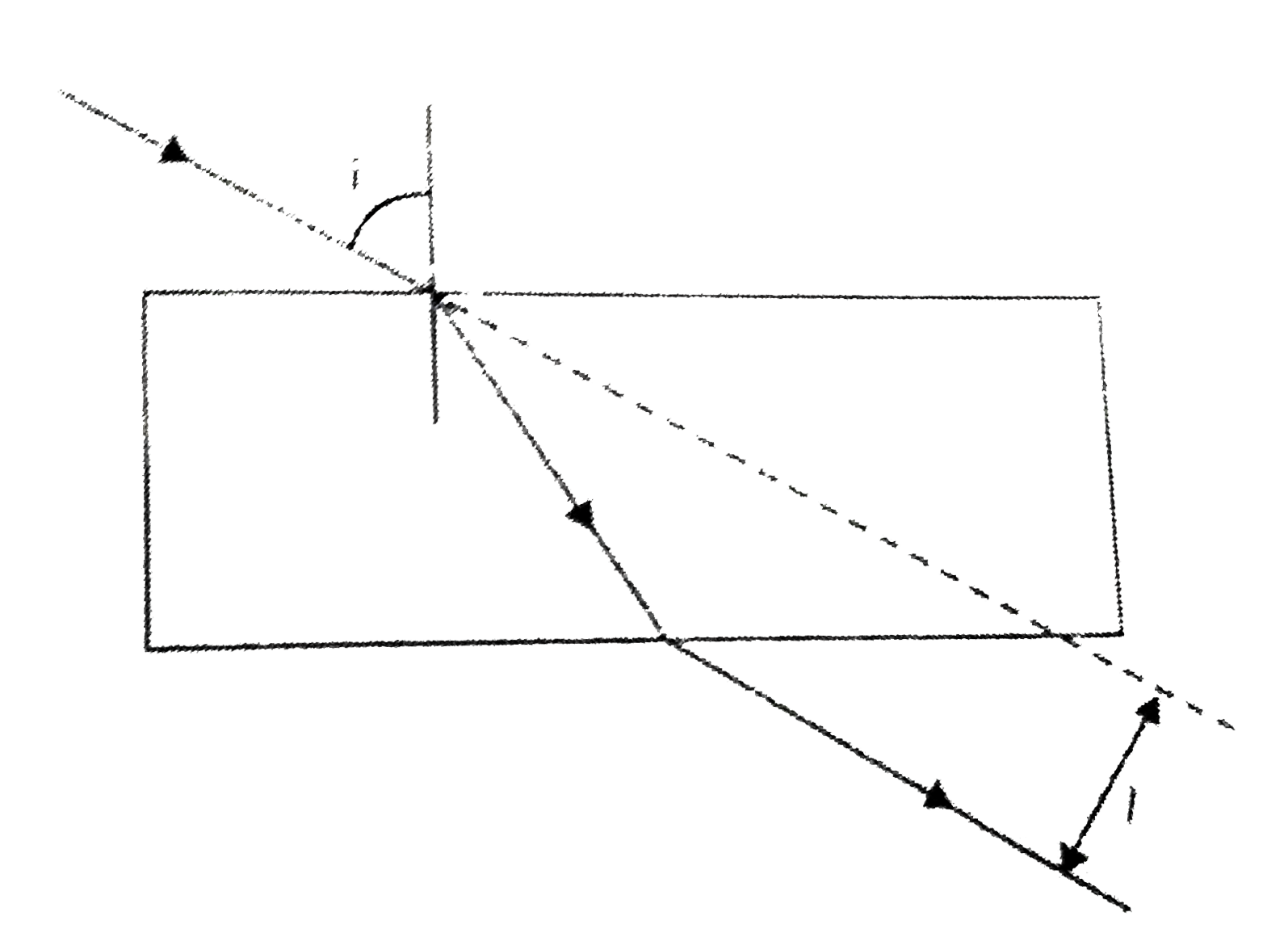
A ray of light enters a rectangular glass slab of refractive index $\sqrt{3}$ at an angle of incidence ${60}^{\circ }$. It travels a distance of 5cm inside the slab and emerges out of the slab. The perpendicular distance between the incident and the emergent rays is

Options

A. `5sqrt(3)cm`
B. `(5)/(2)cm`
C. `5sqrt(3//2)cm`
D. 5cm

Answer

B

Explanation

To solve the problem, we need to find the perpendicular distance between the incident ray and the emergent ray after the light travels through the glass slab. Here’s how we can approach the problem step by step:

Step 1: Identify the Given Parameters
- Refractive index of the glass slab, \( n = \sqrt{3} \)
- Angle of incidence, \( i = 60^\circ \)
- Distance traveled inside the slab, \( d = 5 \, \text{cm} \)

Step 2: Calculate the Angle of Refraction
Using Snell's law:
\( n_1 \sin(i) = n_2 \sin(r) \)
where \( n_1 = 1 \) (air), \( n_2 = \sqrt{3} \), \( i = 60^\circ \), and \( r \) is the angle of refraction.

Substituting the values:
\( 1 \cdot \sin(60^\circ) = \sqrt{3} \cdot \sin(r) \)
\( \sin(60^\circ) = \frac{\sqrt{3}}{2} \)
Thus,
\( \frac{\sqrt{3}}{2} = \sqrt{3} \cdot \sin(r) \)
Dividing both sides by \( \sqrt{3} \):
\( \frac{1}{2} = \sin(r) \)
This gives:
\( r = 30^\circ \)

Step 3: Determine the Perpendicular Distance Inside the Slab
The ray travels a distance of \( d = 5 \, \text{cm} \) inside the slab. We can find the horizontal and vertical components of this distance.

The vertical component (perpendicular distance) can be calculated using:
\( \text{Vertical distance} = d \sin(r) \)
Substituting \( d = 5 \, \text{cm} \) and \( r = 30^\circ \):
\( \text{Vertical distance} = 5 \cdot \sin(30^\circ) = 5 \cdot \frac{1}{2} = 2.5 \, \text{cm} \)

Step 4: Calculate the Total Perpendicular Distance
The total perpendicular distance between the incident and emergent rays is twice the vertical distance (once for entering and once for exiting):
\( \text{Total perpendicular distance} = 2 \cdot \text{Vertical distance} = 2 \cdot 2.5 = 5 \, \text{cm} \)

Final Answer
The perpendicular distance between the incident and the emergent rays is \( 5 \, \text{cm} \).

---