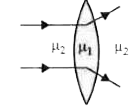
A sphere of radius R made of material of refractive index μ2 is placed in a medium of refractive index μ1. Where would an object be placed so that a real image is formed at equidistant fromk the sphere? ltbgt 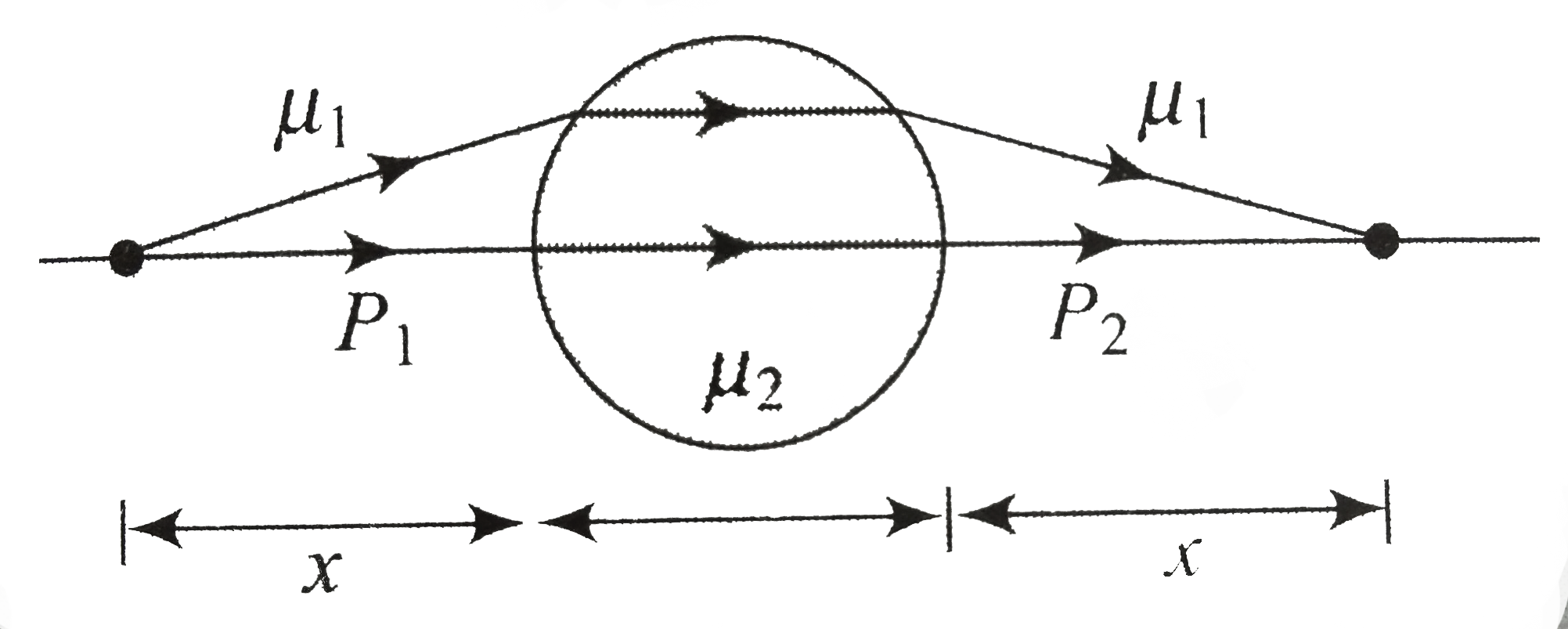
Let the object be placed at a distance x from the pole P1 of the sphere. If a real image is to be formed at equidistant form the sphere, then the rays must pass symmetrical through the sphere, as shown in the figure is question.
Applying the equation at the first surface, we get
μ2+∞−μ1−x=μ2−μ1+R
or x=(μ1μ2−μ1)R
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.6
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.7
21 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.4
12 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK
1 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank
39 Videos
Similar Questions
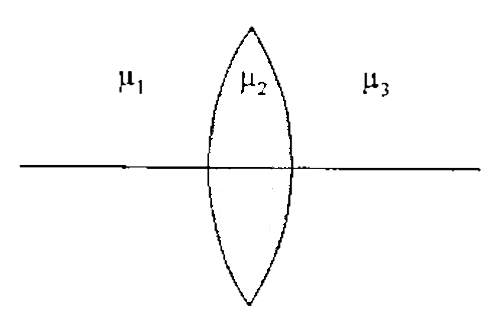
An equiconvex lens of refractive index μ2 is placed such that the refractive :
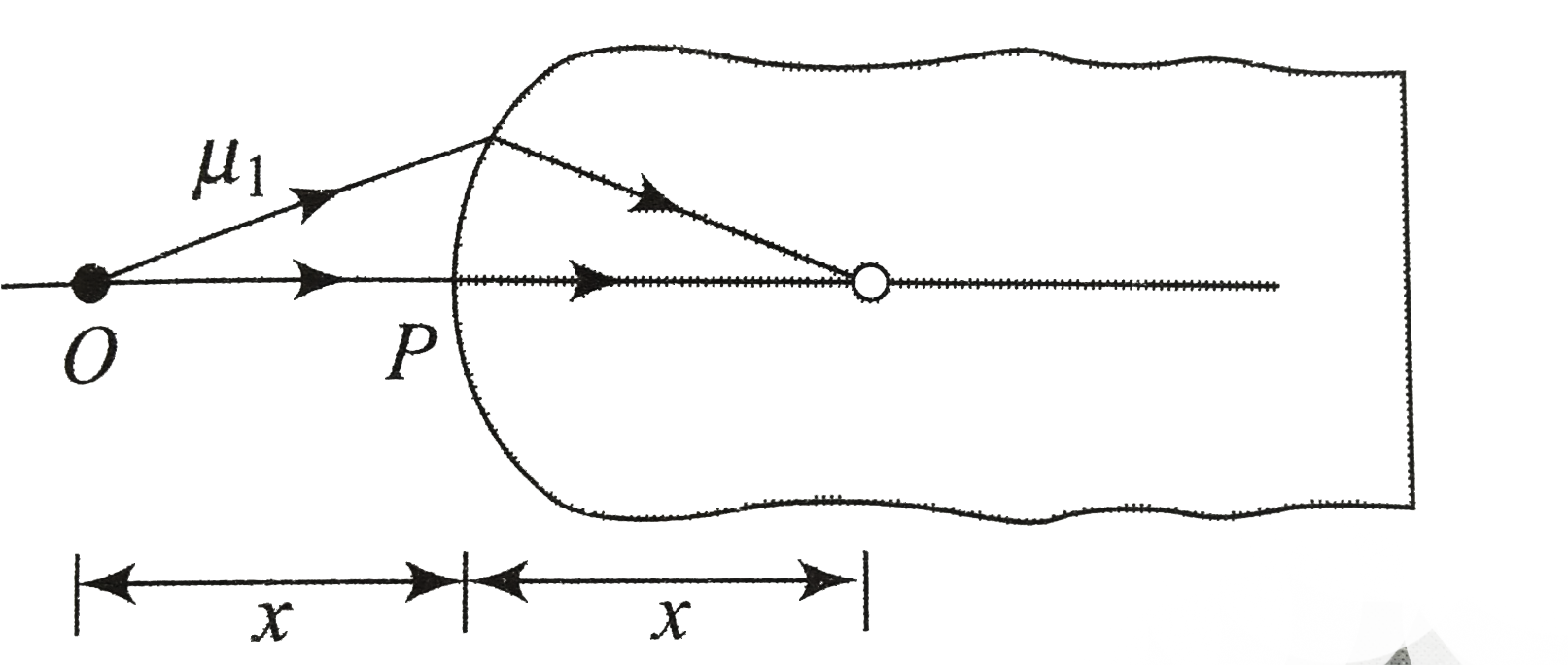
View SolutionA spherical surface of radius R ssparates two media of refractive indices μ1 and μ1 as shown in figure., Where should an object be placed in medium 1 so that a real image is formed in medium 2 at the same distance?
View Solution
Knowledge Check
A lens made of material of Refractive index μ2 is surrounded by a medium of Refractive Index μ1. The focal length f is related as
Af∝(μ2−μ1)Bf∝μ1(μ2−μ1)Cf∝(μ2+μ1)Df∝1(μ2+μ1)There is a sphere of radius R and refractive index μ. At what distance from the surface of sphere should an object be placed so that real image is formed at the same distance from the sphere ?
ARμ−1BRμCR2−μD2Rμ−1If a convex lens of refractive index μl is placed in a medium of refractive index μm Such that μl>μm>μair then
AThe nature of the lens is divergingBThe nature of the lens is convergingCThe nalure of the lens may be converging or divergingDThe nature of the lens can't be determined
A concave mirror of radius R is kept on a horizontal table. Water (refractive index =mu.) is poured into it up to a height h. Where should an object be placed so that its image is formed on itself ?

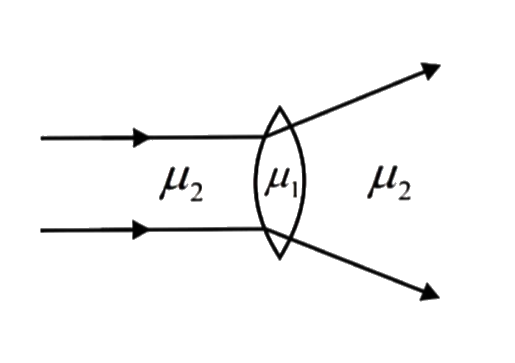
View SolutionA thin bi-convex lens made out of a material of refractive index μ1 is place in a medium of refractive index μ2. A paraxial beam of light, parallel to the principal axis of the lens, is shown in the figure. Based on the ray diagram, we can conclude that
View SolutionWhen a lens of power P (in air) made of material of refractive index μ is immersed in liquid of refractive index μ0 Then the power of lens is:
View SolutionA transparent sphere of radius R and refractie index μ is kept in air. At what distance from the surface of the sphere shold a point object be placed so as to form a rea image at the same distance from the sphere?
View SolutionA convex lens made up of material of refractive index μ1, is immersed in a medium of refractive index μ2 as shown in the figure. The relation between μ1 and μ2 is
View Solution
