A horizontal ray of light passes through a prism whose apex angle is and then strikes a vertical mirror M as shown in Figure. For the ray to become horizontal after reflection, Find the angle by which the mirror must be rotated.
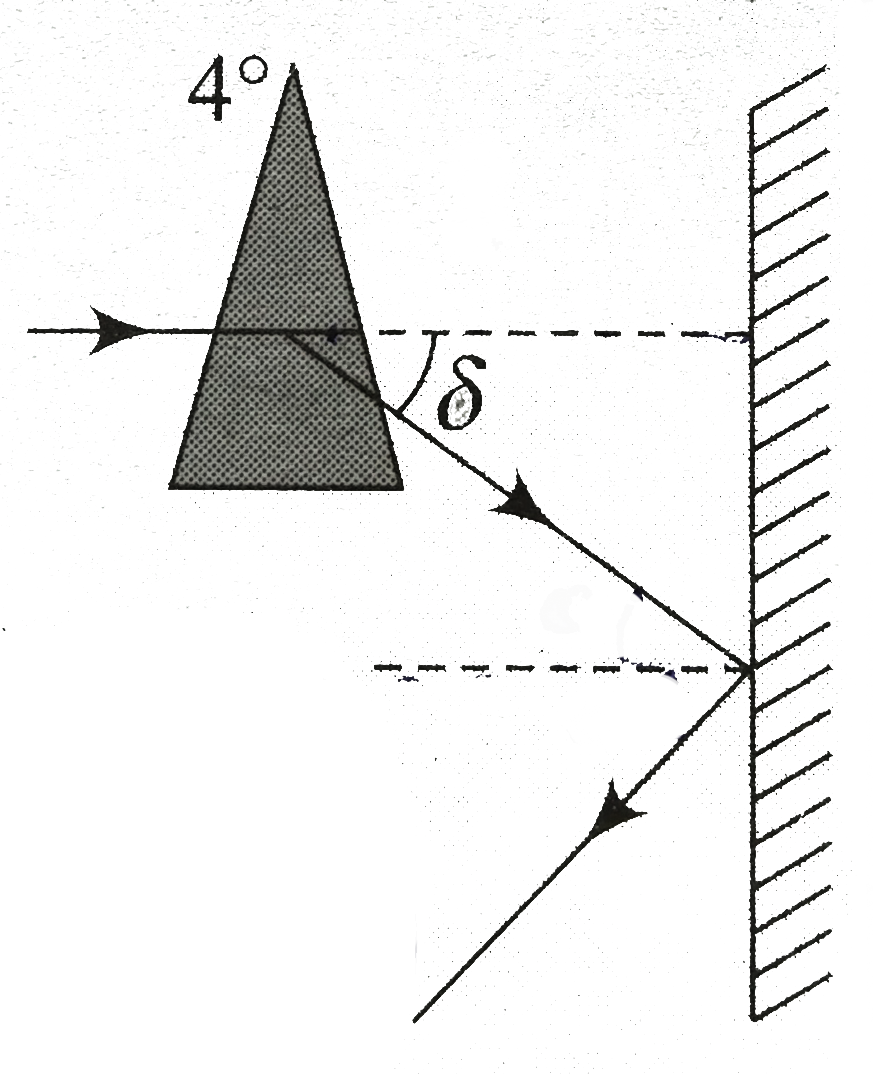
Hence, angle of rotation of mirror

Related Playlists
- BOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.522videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.615videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.325videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK1videos
FRICTION
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank39videos
GRAVITATION
Similar Questions
A horizontal ray of light passes through a prism of index 1.50 and apex angle 4∘ and then strikes a vertical mirror , as shown in the figure. Through what angle must the mirror be rotated if after reflection the ray is to be horizontal?
_E01_041_Q01.png) View Solution
View SolutionA ray of light passes through a prism as shown in the figure.
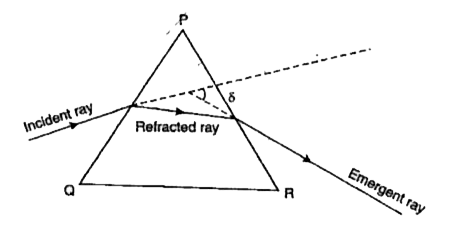
The angle δ is known asView SolutionIf a light ray is reflected from a mirror as shown in the figure, then
 View Solution
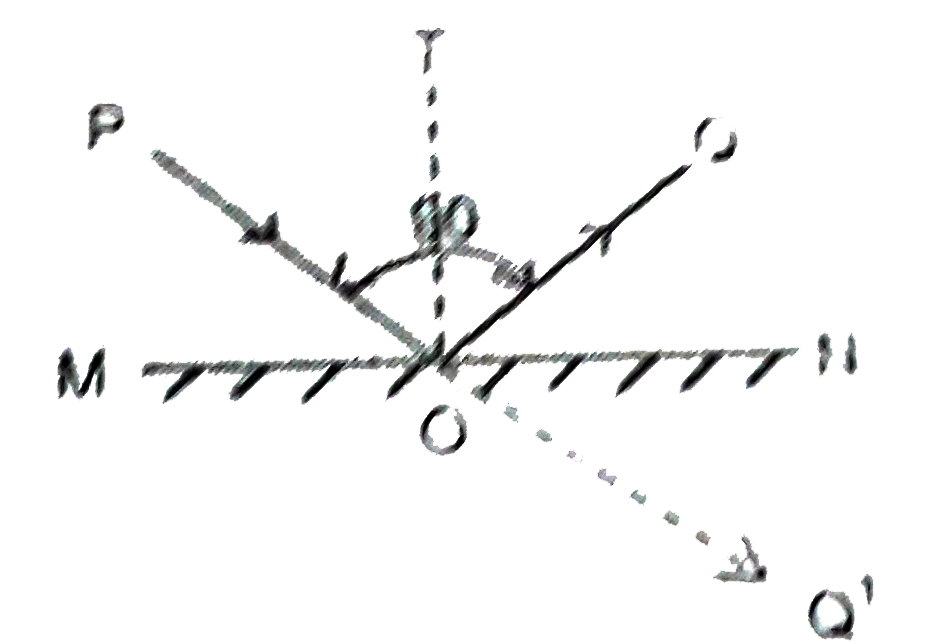
View SolutionIf a light ray is reflected from a mirror as shown in the figure, then
 View Solution
View SolutionA prism having an apex angle 4o and refraction index 1.5 is located in front of a vertical plane mirror as shown in figure. Through what total angle is the ray deviated after reflection from the mirror
 View Solution
View SolutionA prism having an apex angle 4∘ and refractive index 1.5 is located in front of a vertical plane mirror as shown in figure. Through what total angle is the ray is deviated after reflection from the mirror ?
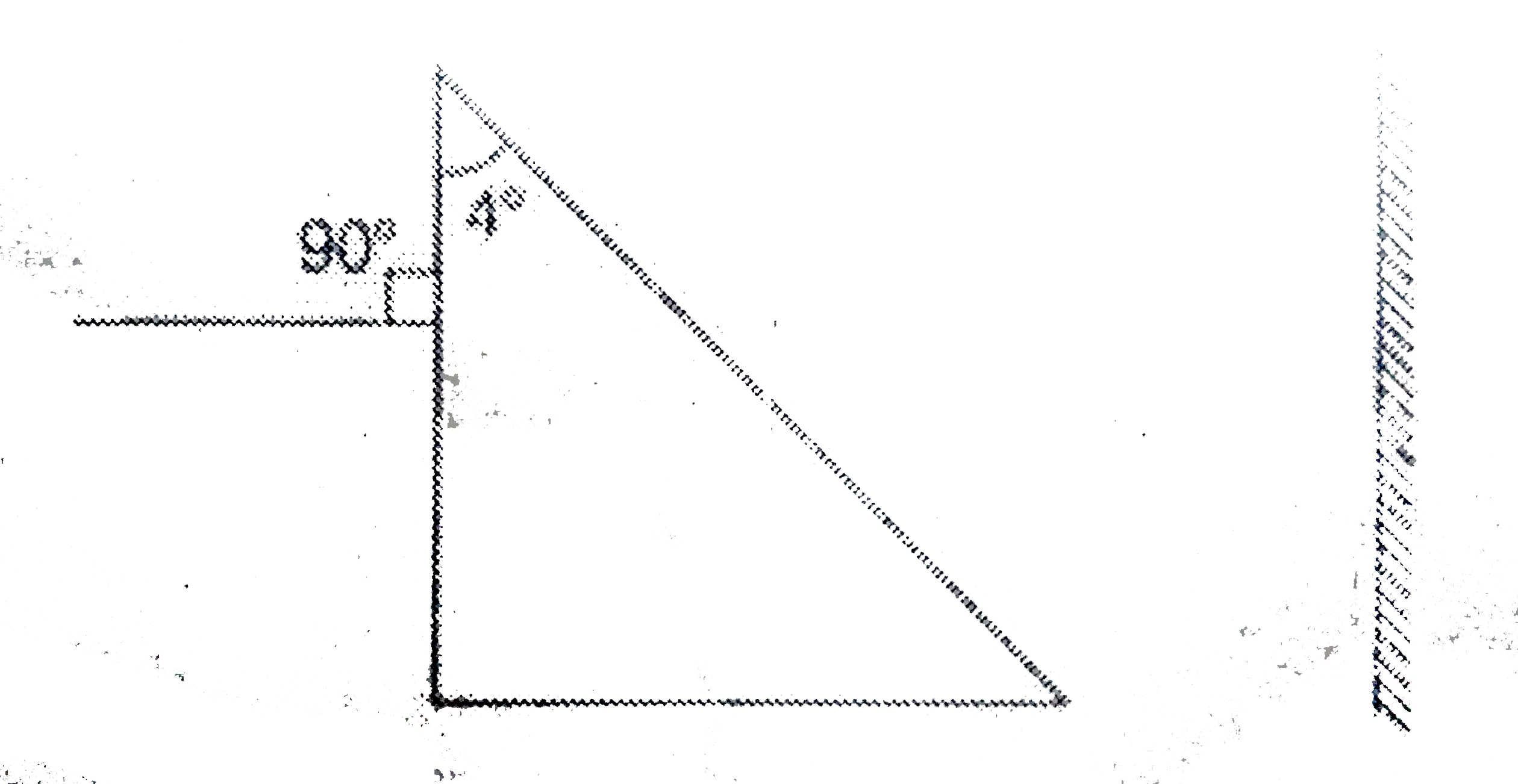 View Solution
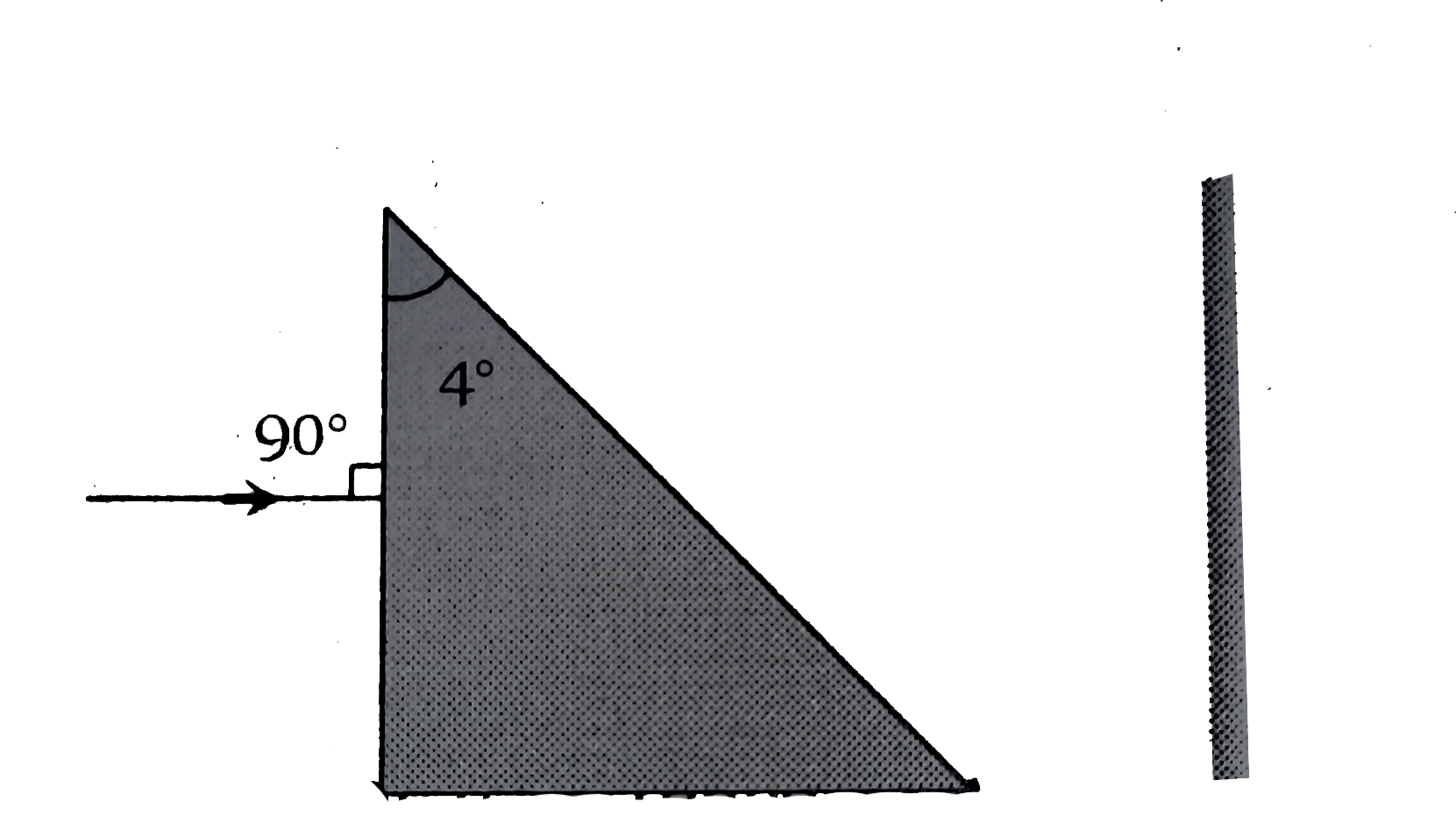
View SolutionA right-angled prism of apex angle 4∘ and refractive index 1.5 is located in front of a vertical plane mirror as shown in figure. A horizontal ray of light is falling on the prism. Find the total deviation produced in the light ray as it emerges 2nd time from the prism.
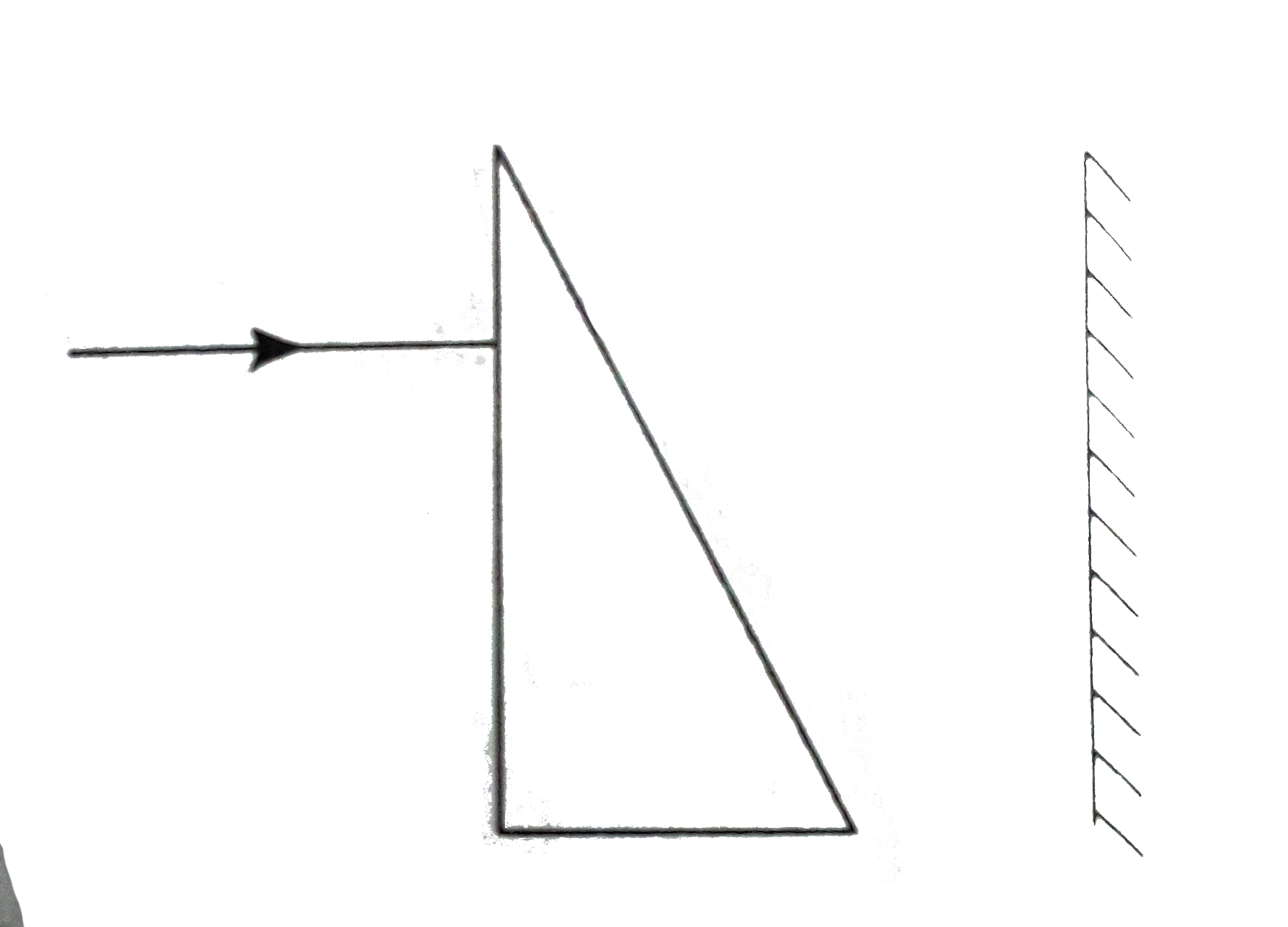 View Solution
View SolutionA ray of light strikes a plane mirror at an angle of incidence 45∘ as shown in Figure . After reflection,l the ray passes through a prism of refractive indes 1.5 whos apex angle is 4∘. Through what angle must the mirror be rotated it total deviation of the ray be 90∘ ?
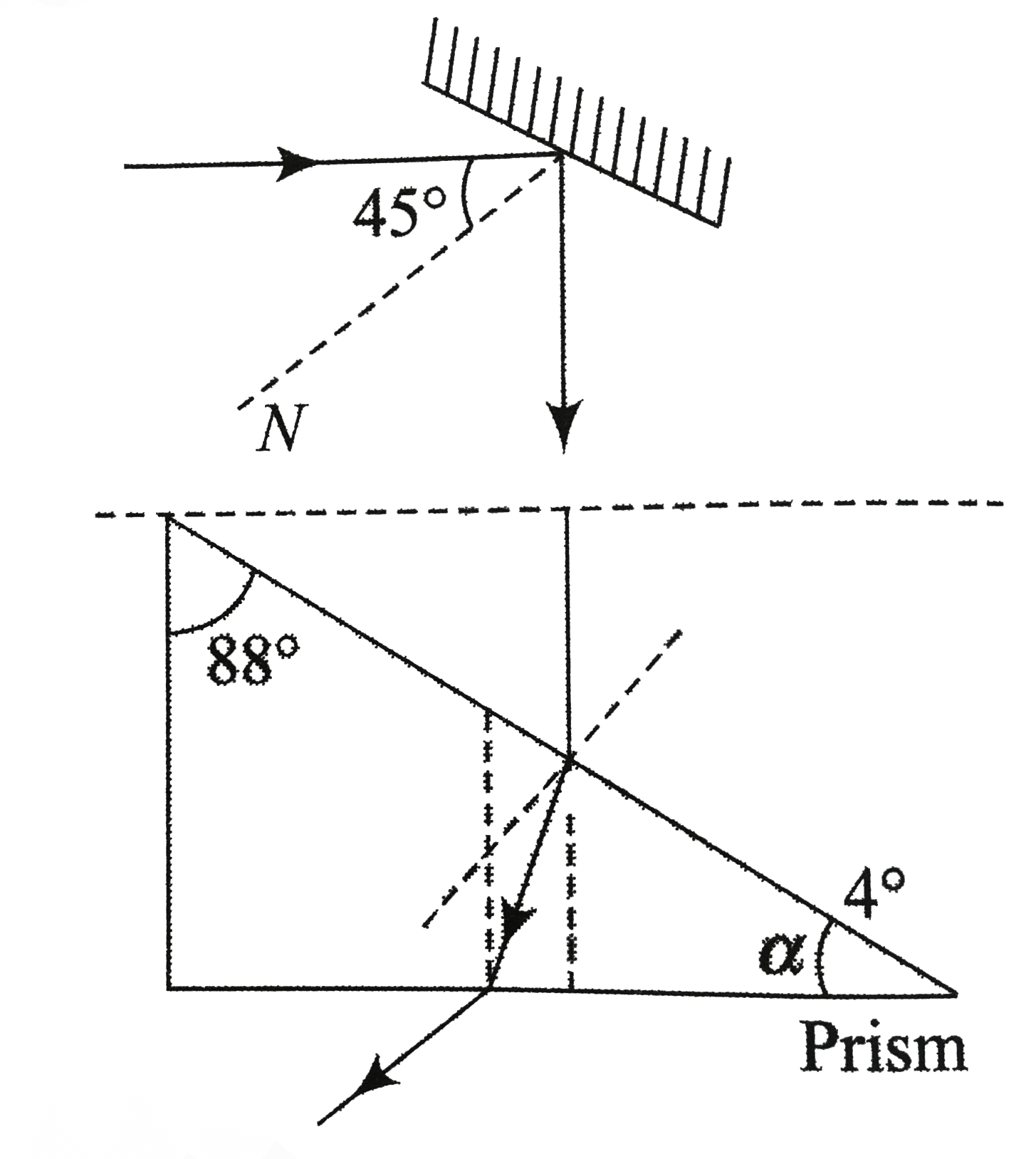 View Solution
View SolutionA small angled prism of apex angle A=4∘ and refractive index μ=1.5 is placed in front of a vertical plane mirror as shown in figure. If the mirror is rotated through an angle θ, then the light ray becomes horizontal either after the mirror or after second time passing from the prism in opposite direction. The value θ is
 View Solution
View Solutionplane mirror makes an angle of 30∘ with horizontal. If a vertical ray strikes the mirror, find the angle between mirror and reflected ray
View Solution


