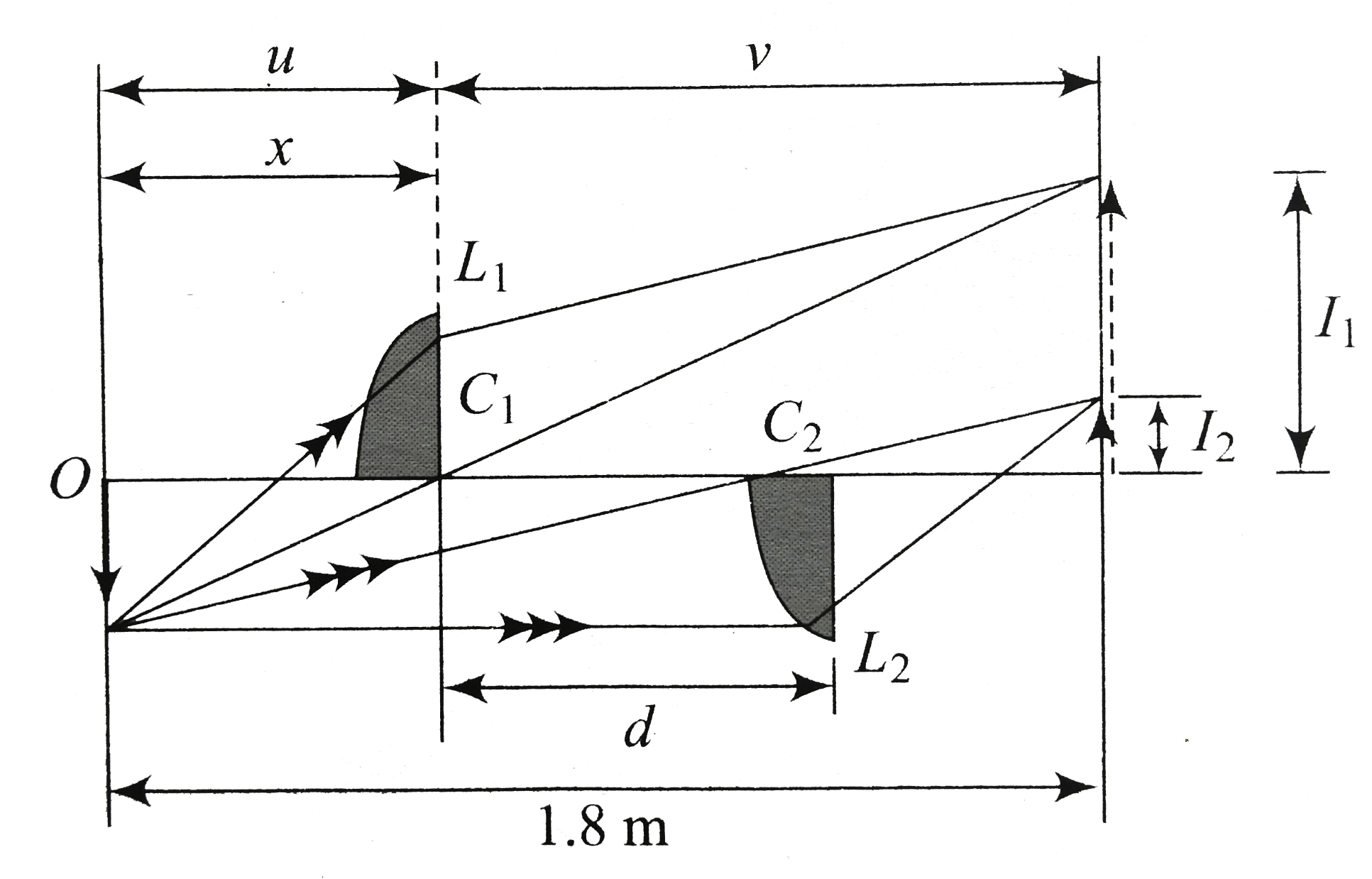
A thin plano-convex lens of focal length f is split into two halves. One of the halves is shifted along the optical axis as shown in figure. The separation between object and image planes is 1.8 m. The magnification of the image, formed by one of the ball lens is 2. Find the focal length of the lens and separation between the two halves. Draw the ray diagram for image formation.

Video Solution

Text Solution

Verified by Experts

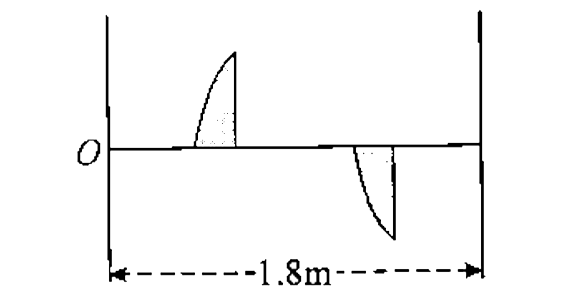
Let magnification caused by the first lens be 2 and distance $O L_{1} = x .$ Distance v of image from first lens $L_{1}$ is given by
$m = \frac{v}{u} = 2 \Rightarrow v = 2 u = 2 x .$
Clearly, $u + v = 1.8 \Rightarrow x + 2 x = 1.8 m$
or $3 x = 1.8 m \Rightarrow x = \frac{1.8}{3} = 0.6 m$
By sign convention,
$u = - x = - 0.6 m, υ = 2 x = 1.2 m$
Lens formula $\frac{1}{f} = \frac{1}{v} - \frac{1}{u}$ gives
$\frac{1}{f} = \frac{1}{1.2} + \frac{1}{0.6} = \frac{1 + 2}{1.2}$
$∴$ Focal length $f = \frac{1.2}{3} = 0.4 m$
For real image, lens formula takes the form
$\frac{1}{f} = \frac{1}{v} + \frac{1}{u}$
Clearly, u and v are interchangeable. Therefore, for lens $L_{2}$
$u^{'} = v = 1.2 m$ and $v^{'} = 0.6 m$
$O L_{1} = L_{2} I_{2} = x$
If d is the separation between the lenses, then
$x + d + x = 1.8 m$
$∴ d = 1.8 - 2 x = 1.8 - 2 \times 0.6 = 0.6 m$
Method-2 Since the magnification for $L_{1}$ is 2
$\Rightarrow \frac{v}{u} = - 2 \Rightarrow \frac{\frac{D + d}{2}}{- \frac{D - d}{2}} = - 2$
$\frac{D + d}{D - d} = 2 \Rightarrow D = 1.8 m, d = 0.6 m .$
$f = \frac{D^{2} - d^{2}}{4 D} = \frac{(1.8 + 0.6) (1.8 - 0.6)}{4 \times 1.8} = 0.4 m$

Updated on:21/07/2023

If a equiconvex lens of focal length f is cut into two halves by a plane perpendicular to the principal axis, then
AThe focal length of each half becomes $\frac{f}{2}$
BThe focal length of each half becomes 2f
CThe focal length of each half remains f
DThe focal length of each half becomes f4
The distance between an object and its real image formed by a lens is D. If the magnification is $m$ , the focal length of the lens is
A $\frac{(m - 1) D}{m}$
B $\frac{m D}{(m + 1)}$
C $\frac{(m - 1) D}{m^{2}}$
D $\frac{m D}{{(m + 1)}^{2}}$
Shown an object and its image formed by a thin lens. Then the nature and focal length of lens is

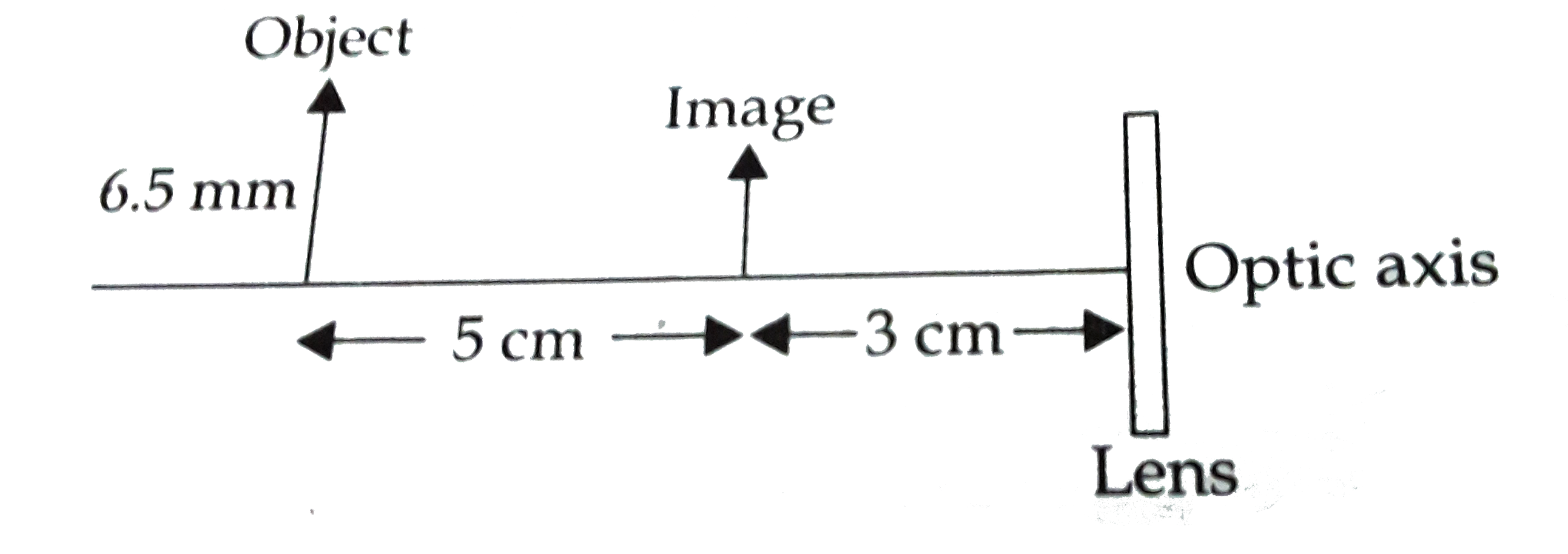A $f = 4.8 c m$ converging lens
B $f = - 4.8 c m$ diverging lens
C $f = 2.18 c m$ converging lens
D $f = - 2.18 c m$ diverging lens