The cross section of a glass prism has the form of an isosceles triangle. One of th erefracting faces is silvered. A ray of light falling normally on the other refracting face, being reflected twice, emerges through the base of the prism perpendicular to it. Find the angles of the prism.
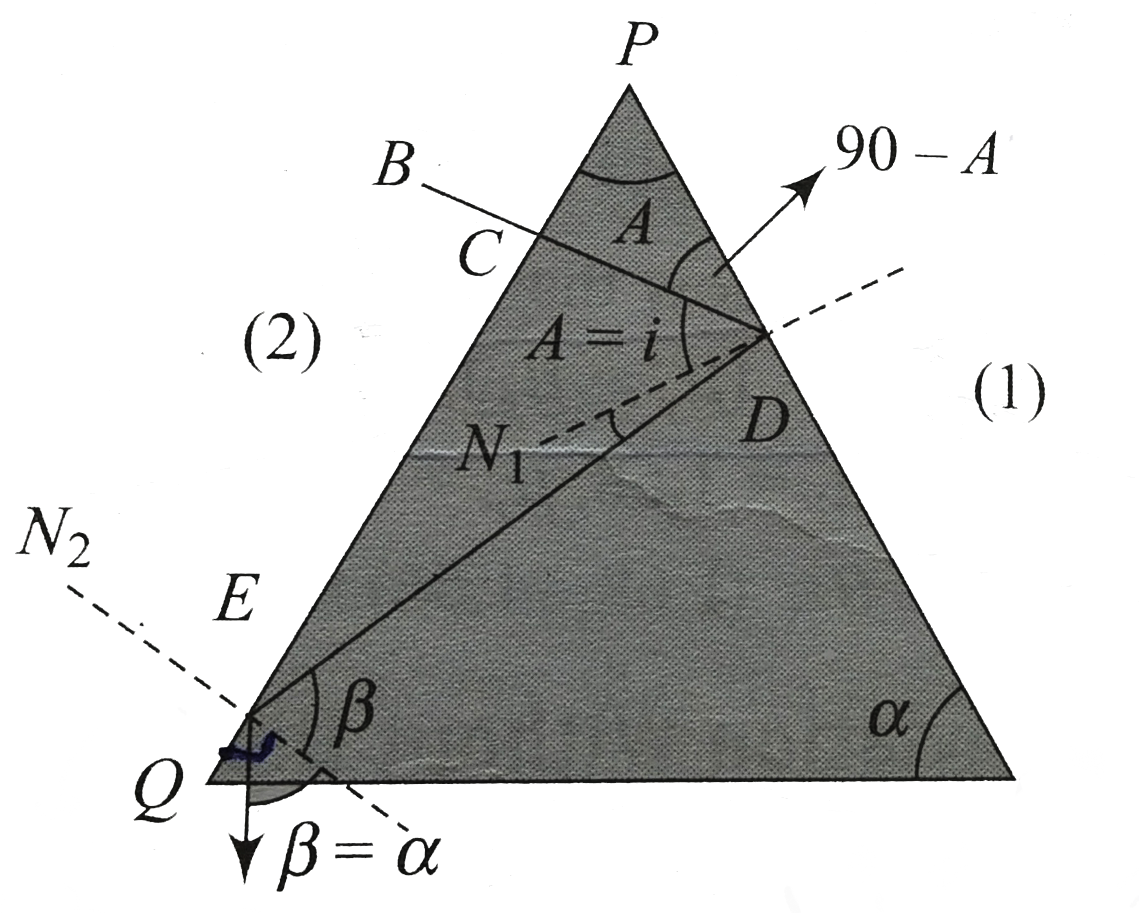
The incident ray BC at normal incidence is reflected at silvered face, along DE and at E it again sufferes reflection along EF. Since the ray emerges normally from the base, therefore the ray EF must fall normally on the base and emerges along EG.
We find i=A.
Also, β=d
Since EN2∣∣CD,β=2i (alternate angles)
∴α=2A(β=α,i=A) (i)
Also, 2α+A=180∘
(∵ Sum of angles of a triangle = 180∘) (ii)
Solving Eqs. (i) and (ii), we get A =36∘,α=72∘ .
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Solved Examples
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.1
14 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK
1 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank
39 Videos
Similar Questions
An isosceles prism has one of the refracting surfaces silvered. A ray of light is incident normally on the refracting face AB. After two reflections, the ray emerges from the base of the prism perpendicular to it. Find the angle of the prism.
View SolutionAn isosceles glass prism has one of its faces silvered. A light ray is incident normally on the other face which is identical in size to the silvered face. The light ray is reflected twice on the same sized faces and emerges through the base of the prism perpendicularly. Find the minimum value of refractive index of the material of the prism.
View Solution
Knowledge Check
A ray of light is incident normally on one of the refracting surfaces of a prism of refracting angle A,. The emergent ray grazes the other refracting surface. Find the refractive index of the material ofprism.
A1.155B1.75C1.33D1.66If a light ray incidents normally on one of the faces of the prism of refractive index 2 and the emergent ray just grazes the second face of the prism, then the angle of deviation is
A0∘B30∘C45∘D60∘A ray of light is incident normally on one face of a right-angled isosceles prism and then it grazes the hypotenuse. The refractive index of the material of the prism is
A1.33B1.414C1.5D1.732
A ray of light incident normally on the first face of a glass prism of refractive index 1.6 just emerges from the other face. Find the angle of the prism.
View SolutionThe cross section of a glass prism μ=2 has the form of an isosceles right-angled triangle. It is submerged in a liquid of refractive index 1.2. A ray is incident on to one of the equal faces perpendicularly. What is the angle between the direction of incident ray and the ray that emerges from the prism ?
View SolutionA ray of light falls on a normally on a refracting face of a prism. Find the angle of prism if the ray just fails to emerge from the prism (μ=3/2).
View SolutionThe principal section of a glass prism is an isosceles Δ(PQR) with PQ=PR. The face PR is silvered. A ray is incident perpendicularly on face PQ and after two reflections it emerges from base QR, normal to it. The angle of the prism is given by πα(rad). Find the value of α.
View SolutionOne face of prism of refracting angle 30∘ and refractive index 1.414 is silvered. At what angle must a ray of light fall on the unsilvered face so that it retraces its path out of the prism ?
View Solution
