A dielectric slab of thickness d is inserted in a parallel plate capacitor whose negative plate is at x=0 and positive plate is at x=3d. The slab is equidistant from the plates. The capacitor is given some charge. As one goes from 0 to 3d:
the magnitude of the electric field remains the same
the direction of the electric field remains the same
the electric potential increases continuously
the electric potential increases at first, then decreases and again increases

In regions I and III there will be electric field →E0 directed from positive to negative in region II due to orientation of dipoles there is an electric field →E0 present in the opposite direction of →E0 but since →E0 is also present the net electric field is E0−Ek in the direction of →E0 as shown in fig. (∵E0>Ek)
Also, please note that when one moves opposite to the direction of electric field the potential always increases, the stronger the electric field the more is the increase in potential sice in region II the electric field is less as comparred to regions I and III the increase in potential will be less but there has to be less but there has to be an increase in potential in all the regions from x=0 to x=3d.
Similar Questions
A dielectric slab of thickness 't' is kept between the plates of a parallel plate capacitor separated by a distance 'd' (t<d) . Derive the expression for the capacity of the capacitor .
View SolutionIf a dielectric slab of thickness t and area A is inserted in between the plates of a parallel plate capacitor of plate area A and distance between the plates d(d>t) then find out capacitance of system. What do you predict about the dependence of capacitance on location of slab?
View Solution
Knowledge Check
A dielectric slab of thickness d is inserted in a parallel plate capacitor whose negative plate is at x=0 and positive plate is at x=3d. The slab is equidistant from the plates. The capacitor is given some charge. As one goes from 0 to 3d:
Athe magnitude of the electric field remains the sameBthe direction of the electric field remains the sameCthe electric potential increases continuouslyDthe electric potential increases at first, then decreases and again increasesA dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
Abecomes zeroBremains the sameCdecreasesDincreasesA positive charge q is given to each plate of a parallel plate air capacitor having plate area A and plate separation d, then
Asince both the plates are identially charged, therefore, capacitance become equal to zeroBenergy stored in the space between the capacitor plates is equal to q2ε0A2Cno charge appears on inner surface of the platesDpotential difference between the plates is equal to 2qdε0A
A conducting slab of thickness ‘t’ is introduced between the plates of a parallel plate capacitor, separated by a distance d (t<d). Derive an expression for the capacitance of the capacitor. What will be its capacitance when t = d ?
View SolutionA parallel plate capacitor having plate area A and separation between the plates d is charged to potential V.The energy stored in capacitor is ....???
View SolutionA conducting slab of thickness 't' is introduced without touching between the plates of a parallel plate capacitor , separated by a distance 'd' (t<d) . Derive an expression for the capacitance of the capacitor .
View SolutionA slab of copper of thickness b is inserted in between the plates of a parallel plate capacitor as shown in figure. The separation of the plates is d. If b=d/2, then the ratio of capacities of the capacitor after and before inserting the slab will be
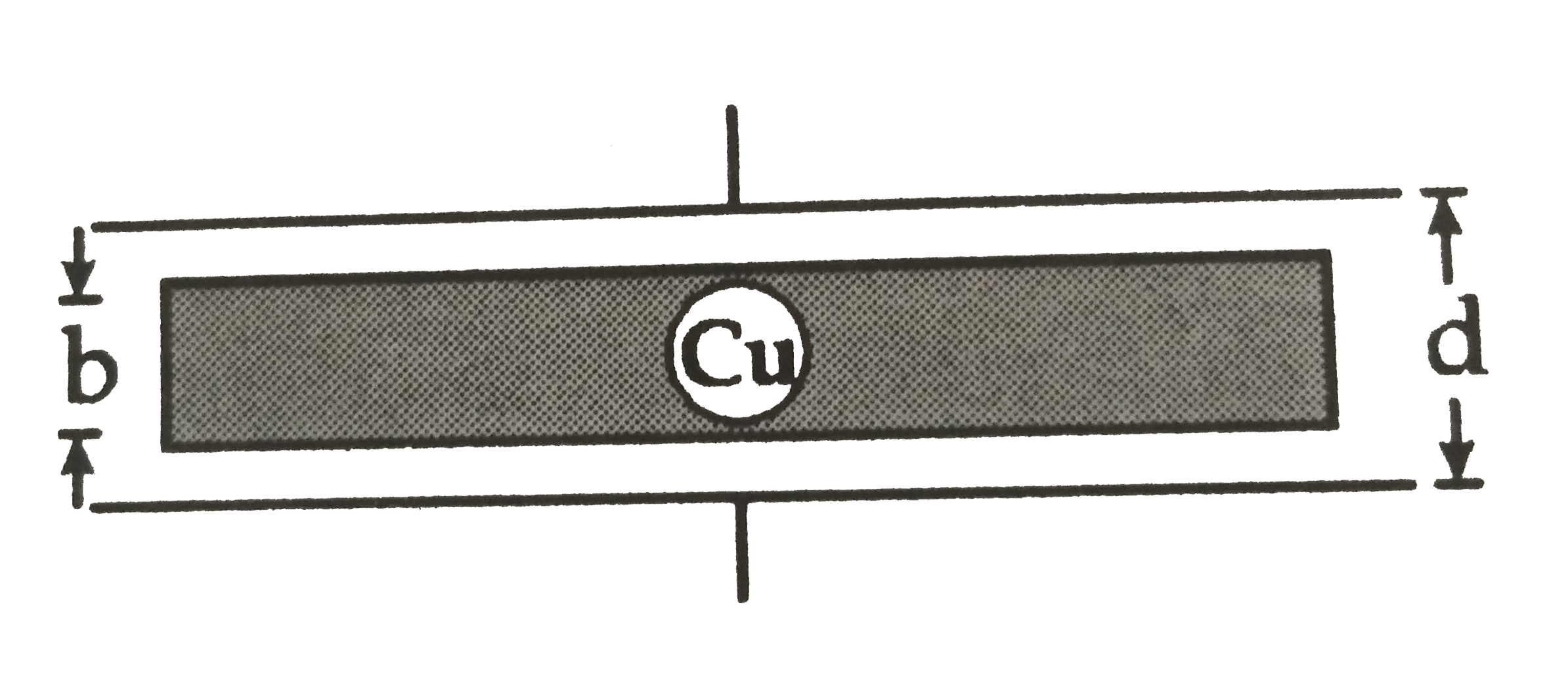
View SolutionA slab of copper of thickness b is inserted in between the plates of parallel plate capacitor as shown in figure. The separation of the plate is d. If b=d/2, then the ratio of capacities of the capacitor after and before inserting that slab will be

View Solution
