If a wire of resistance 20Ω is covered with ice and a voltage of 210V is applied across the wire , then the rate of melting of ice is
8.85gs−1
1.92gs−1
6.56gs−1
none of these
The correct Answer is:C
The rate of heat developed is given by
V2R=(210)220=2205Js−1=22054.2cals−1
Let m be the amount of ice melting per sec and , then
m×80=22054.2orm=6.56gs−1
Topper's Solved these Questions
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICSCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Multiple Correct
5 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICSCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Assertion - Reasoning
6 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICSCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Subjective
8 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank
39 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICSCHAPTER - INDUCTANCEEXERCISE - Concept Based
9 Videos
Similar Questions
A coil of enamelled copper wire of resistance 50Ω is embedded in a block of ice and a potential difference of 210V applied across it. Calculate the rate of which ice melts. Latent heat of ice is 80 cal per gram.
View SolutionA coil of enamelled copper wire of resistance 50Ω is embedded in a block of ice and a potential difference of 210V applied across it. Calculate the rate of which ice melts. Latent heat of ice is 80 cal per gram.
View Solution
Knowledge Check
A coil of wire of resistance 50Ω is embedded in a block of ice. If a potential difference of 210 V is applied across the coil, the amount of ice melted per second will be
A4.12gmB4.12kgC3.68kgD2.625gmA coil of wire of resistance 50Ω is embedded in a block of ice and a potential difference of 210 V is applied across it. The amount of ice which melts in 1 second is [ latent heat of fusion of ice =80calg−1]
A0.262 gB2.62 gC26.2 gD0.0262 gA coil of water of resistance 50Ω is embedded in a block of ice and a potential difference of 210 V is applied across it. The amount of ice which melts in 1 second is [ latent heat of fusion of ice =80calg−1]
A0.262gB2.62gC26.2gD0.0262g
A coil of enamelled copper wire of resistance 50Ω is embedded in a block of ice and a potential difference of 210 V applied across it. Calculate, how much ice will melt in half minute. Latent heat of ice is 180 cal per gram.
View Solution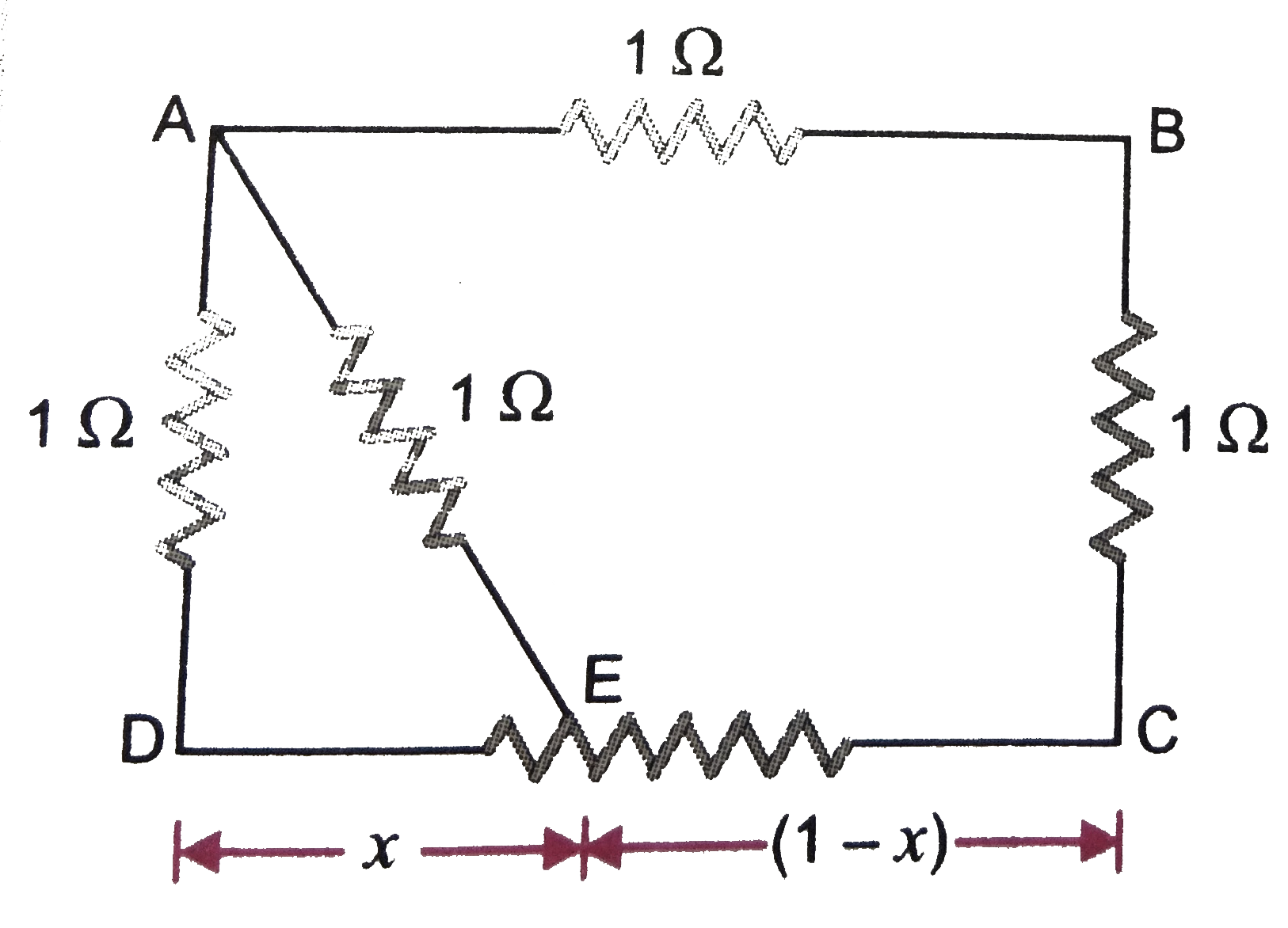
ABCD is a square where each side is a uniform wire of resistance 1Ω. Find a point E on CD such that if a uniform wire of resistance 1Ω is connected across AE and a constant potential is applied across A and C, the points B and E will be equipotential.
View SolutionA wire of resistance 5Ω is bent in the form of a closed circle. Find the resistance across the diameter of the circle.
View SolutionA length of uniform ‘heating wire’ made of nichrome has a resistance 72 Ω. At what rate is the energy dissipated if a potential difference of 120V is applied across (a) full length of wire (b) half the length of wire (wire is cut into two). Why is it not advisable to use the half length of wire ?
View SolutionA 10 m long wire ofresistance 20Ω is connected in series with battery of EMF 3V and negligible internal resistance and a resistance of 10Ω. The potential gradient along the wire is :
View Solution
