Consider 12 resistor arranged symmetrically in shape of bipyraimd ABCDEF. Here ABCD is a square. Point E, point F, and centre of square are in the same straight line perpendicular to the planw of squar. The resistacne of each resistor is R. 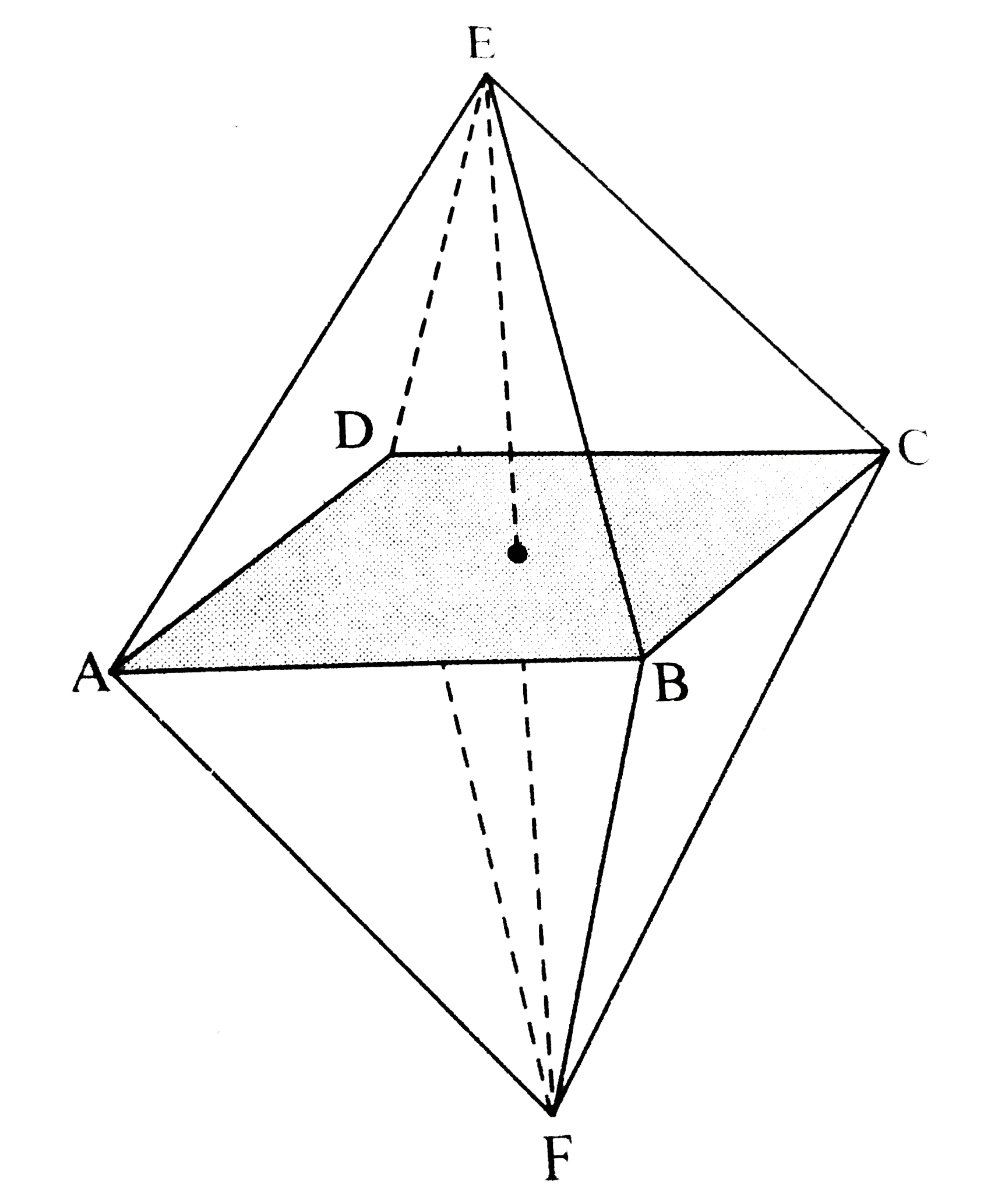
The effective resistance between E and F is
R/2
R/3
R
none of these
The correct Answer is:A
If we cannot battery between E and F, then A, B, C, and D will be
all at the same potential. Req=R4+R4=R2 
If we connect a battery
between A and C, then E and
F will be at the same potential.
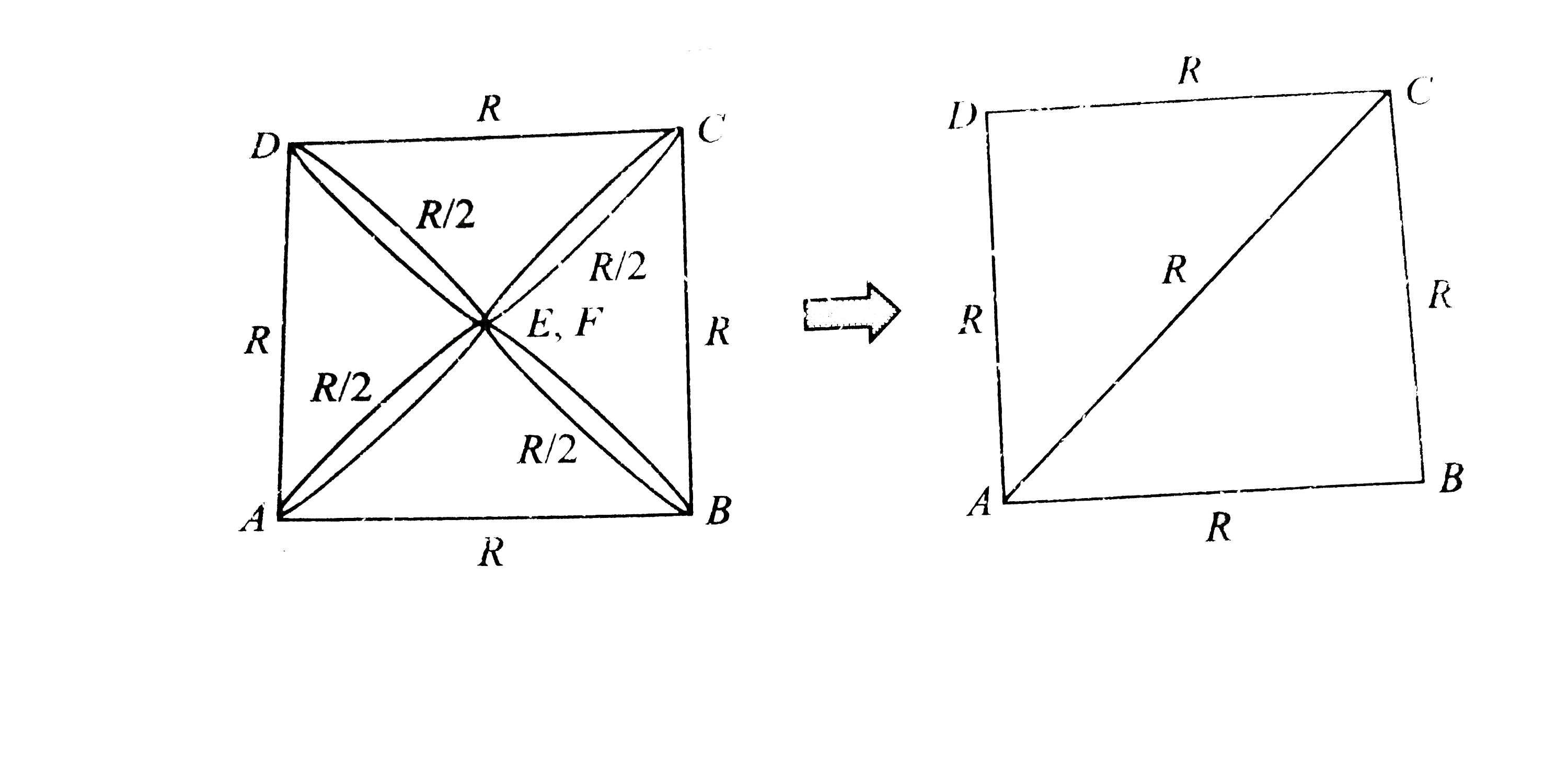 ,
, 
Now resistance between DE and EB will be useless. Thus,
Req=R/2
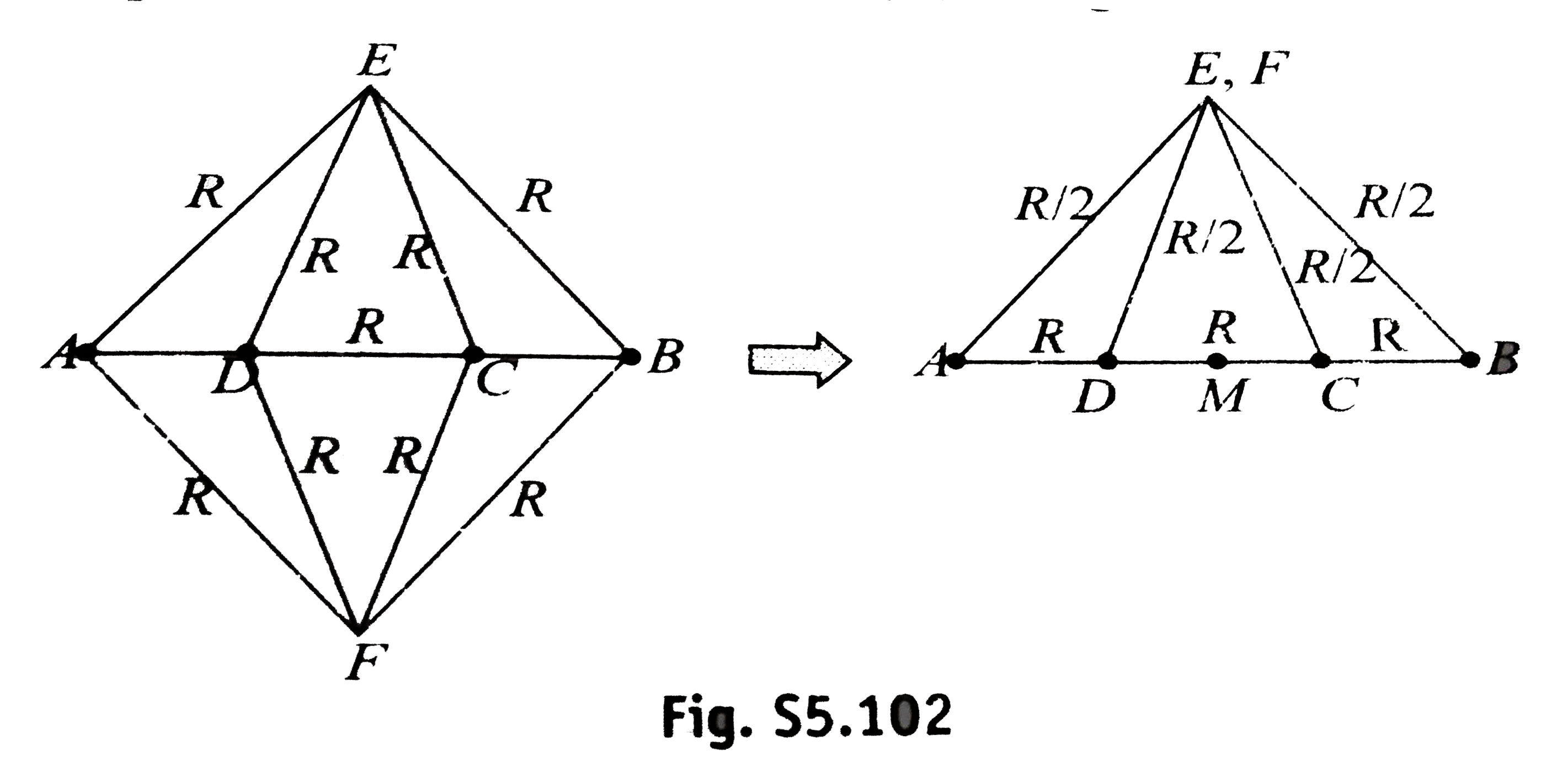
Let us remove the resistance between A and B, then E and F will be at
the same potential, we can draw the remaining as follows:
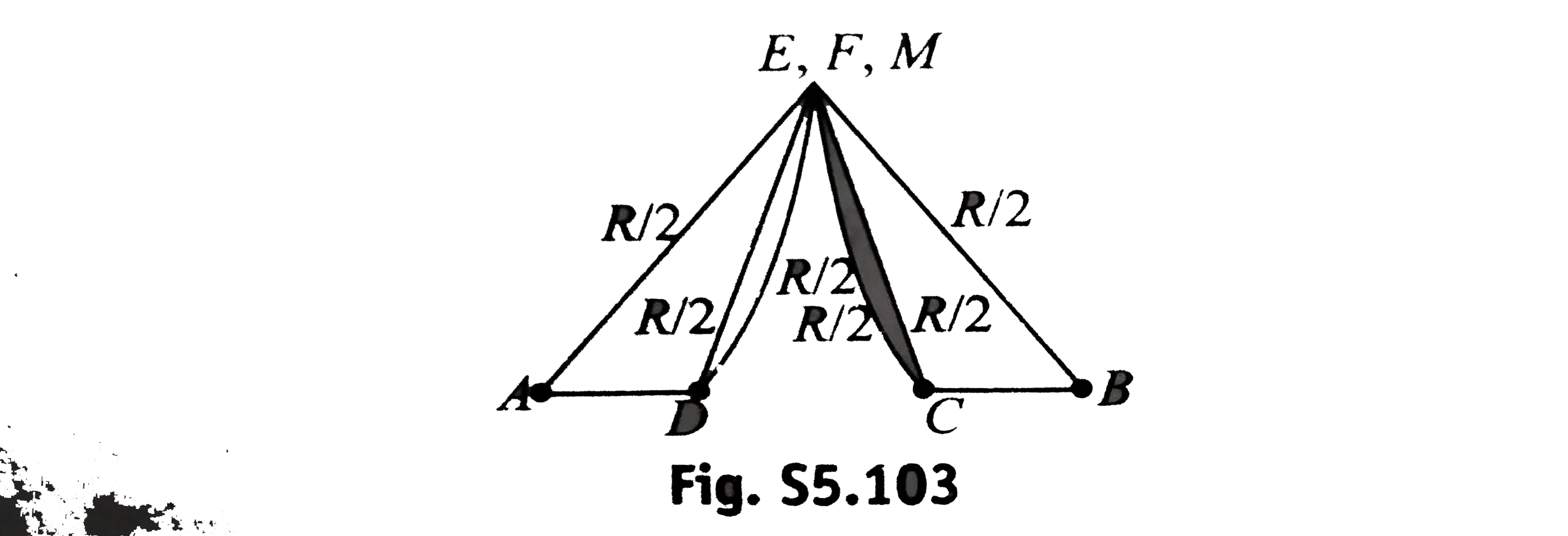 ,
,
M is the midpoint of DC, so M will be at the same spot as E and F.
Now find RAB=5/7R. The resistance that we had removed will be in
parallel to it, so
Req=RABRRAB+R=(5/7)RR(5/7)R+R=512R
Topper's Solved these Questions
- ELECTRIC CURRENT AND CIRCUITBook:CENGAGE PHYSICSChapter:ELECTRIC CURRENT AND CIRCUITExercise:Interger
Explore 8 Videos
- ELECTRIC CURRENT AND CIRCUITBook:CENGAGE PHYSICSChapter:ELECTRIC CURRENT AND CIRCUITExercise:Multiple Correct
Explore 16 Videos
- ELECTRIC CURRENT & CIRCUITSBook:CENGAGE PHYSICSChapter:ELECTRIC CURRENT & CIRCUITSExercise:Kirchhoff s law and simple circuits
Explore 15 Videos
- ELECTRIC FLUX AND GAUSS LAWBook:CENGAGE PHYSICSChapter:ELECTRIC FLUX AND GAUSS LAWExercise:MCQ s
Explore 38 Videos
Similar Questions
A wire of resistance R is bent to form a square ABCD as shown in the figure. The effective resistance between E and C is: (E is mid-point of arm CD)
View SolutionABCD is a square of side length 'a'. Its side AB slides between x and y-axes in first quadrant. The locus of the foot of perpendicular drooped from the point E on the diagonal AC (where E is the midpoint of side AD) is
View Solution
Knowledge Check
Consider 12 resistor arranged symmetrically in shape of bipyraimd ABCDEF. Here ABCD is a square. Point E, point F, and centre of square are in the same straight line perpendicular to the planw of squar. The resistacne of each resistor is R.
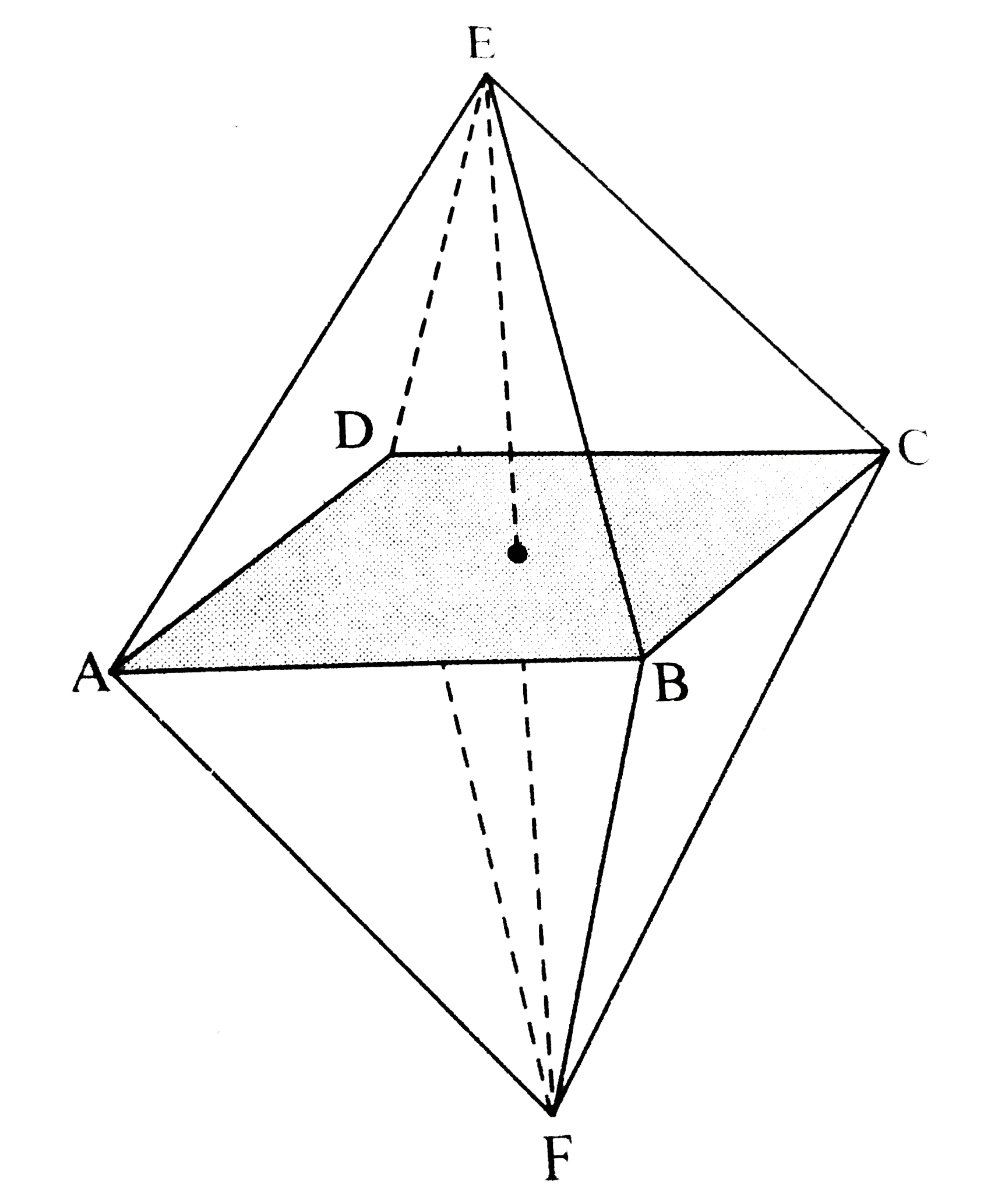
The effective resistance between A and B isA7R/12B5R/7C5R/12Dnone of theseConsider 12 resistor arranged symmetrically in shape of bipyraimd ABCDEF. Here ABCD is a square. Point E, point F, and centre of square are in the same straight line perpendicular to the planw of squar. The resistacne of each resistor is R.
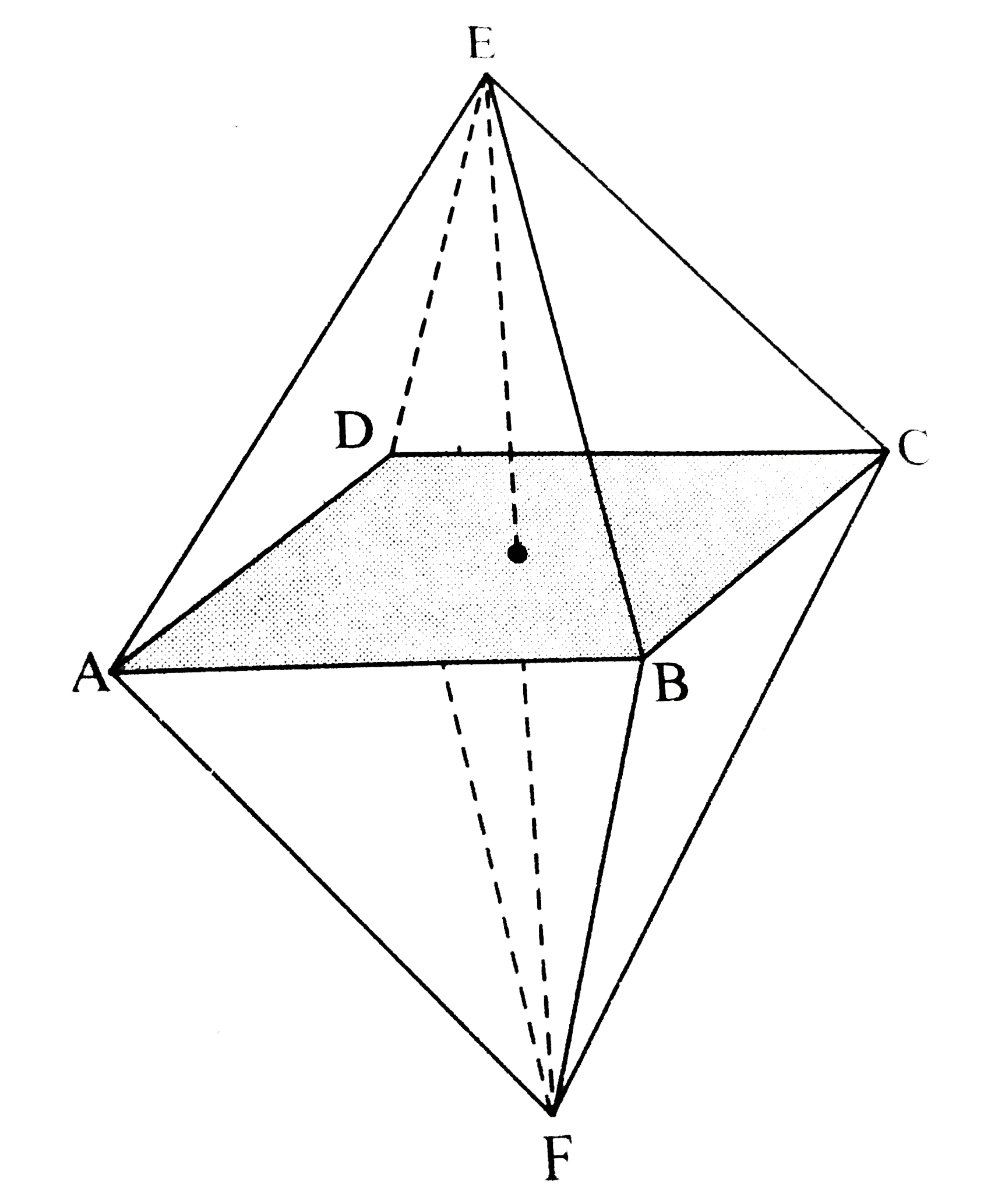
The effective resistance between A and C isAR/2BR/3CRDnone of theseABCD is a square E,F,G,H are the mid-point of the four sides.Then the figure EFGH is
AsquareBrectangleCtrapeziumDparallelogram
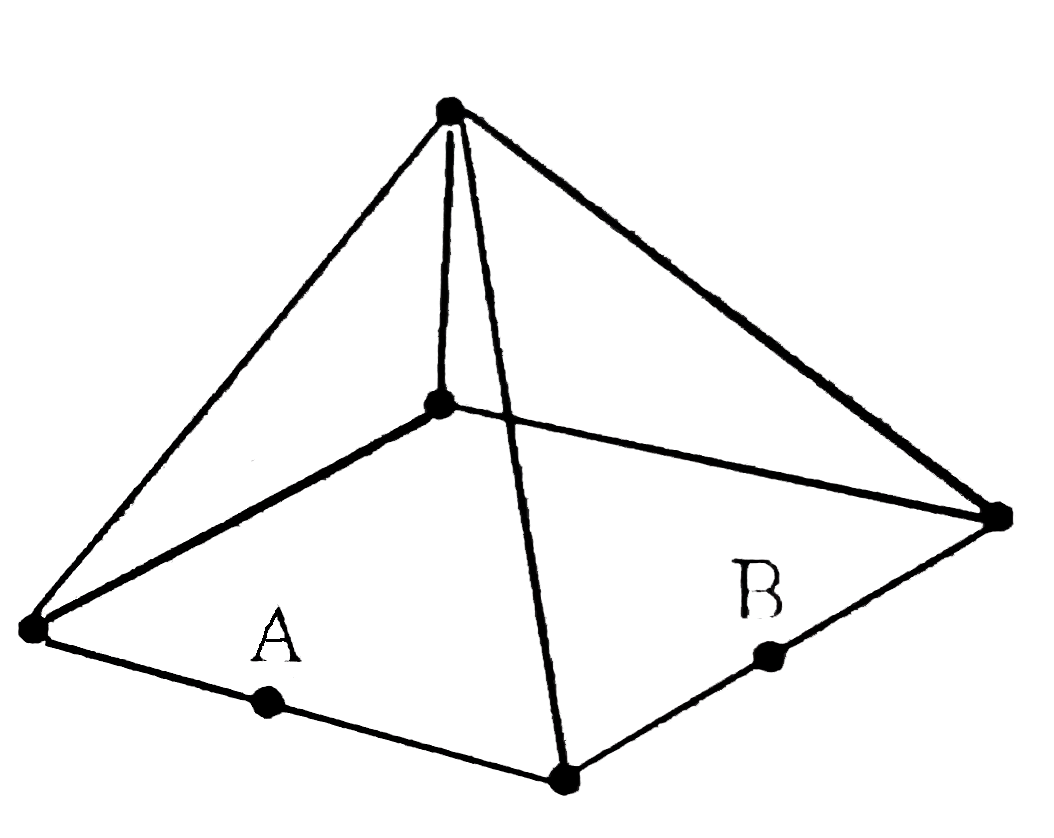
A regular pyramid of square is made of eight identical wires each resistance 12Ω shown in the figure. The terminals A and B are the mid-point of the corresponding sides. Find equivalent resistances between the terminals A and B in ohms.
View SolutionIn the shown wire frame, each side of a square (the smallest square) has a resistance R. The equivalent resistance of the circuit between the points A and B is
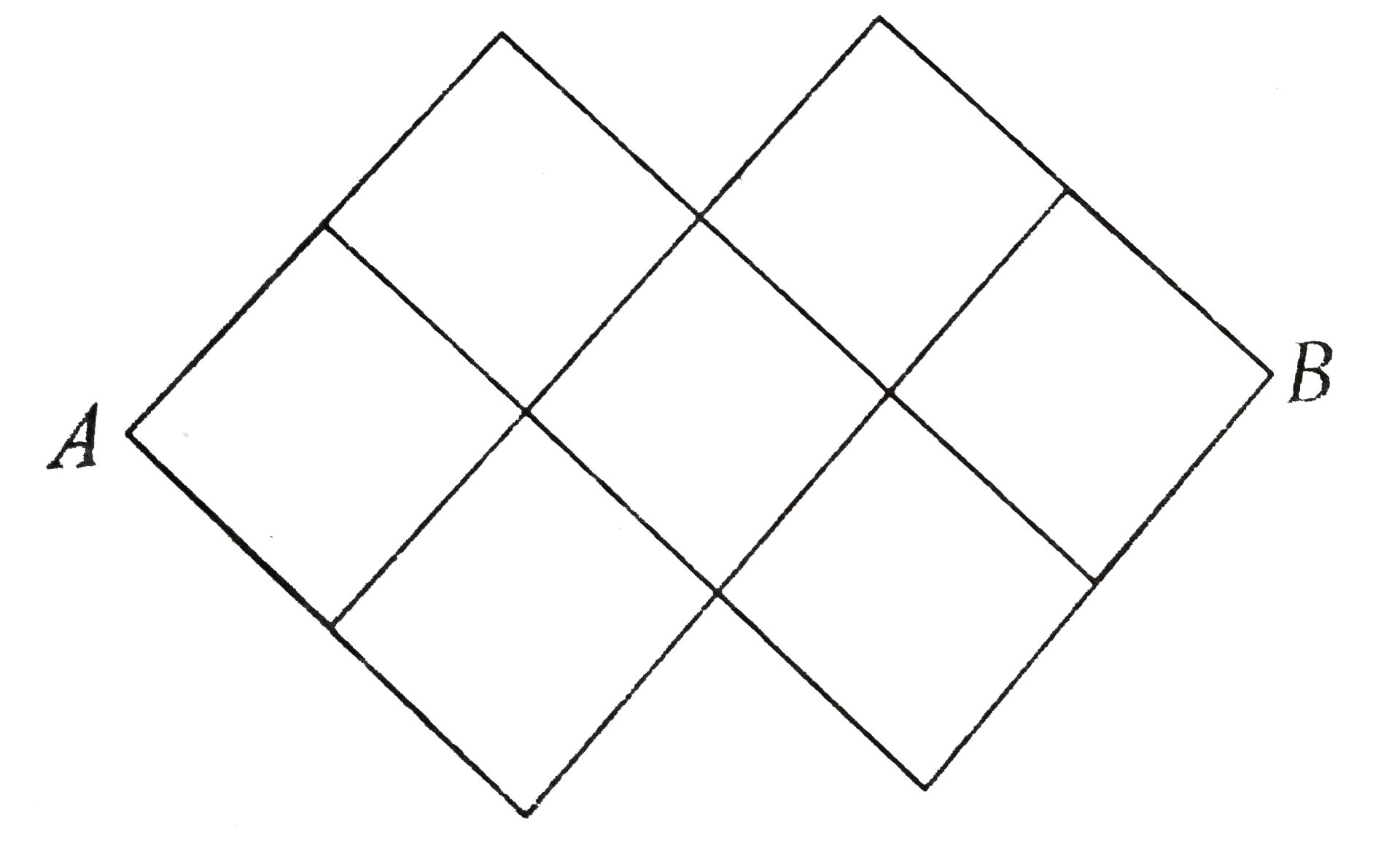
View Solution_2019_CBT3_E01_033_Q01.png)
A wire of length l and resistance R is bent in form of square form of square as shown in figure. If E is a mid point of side D,A, then equivalent resistance between points E& A is:
View SolutionThe equivalent resistance between the points A and B is (R is the resistance of each side of smaller square)
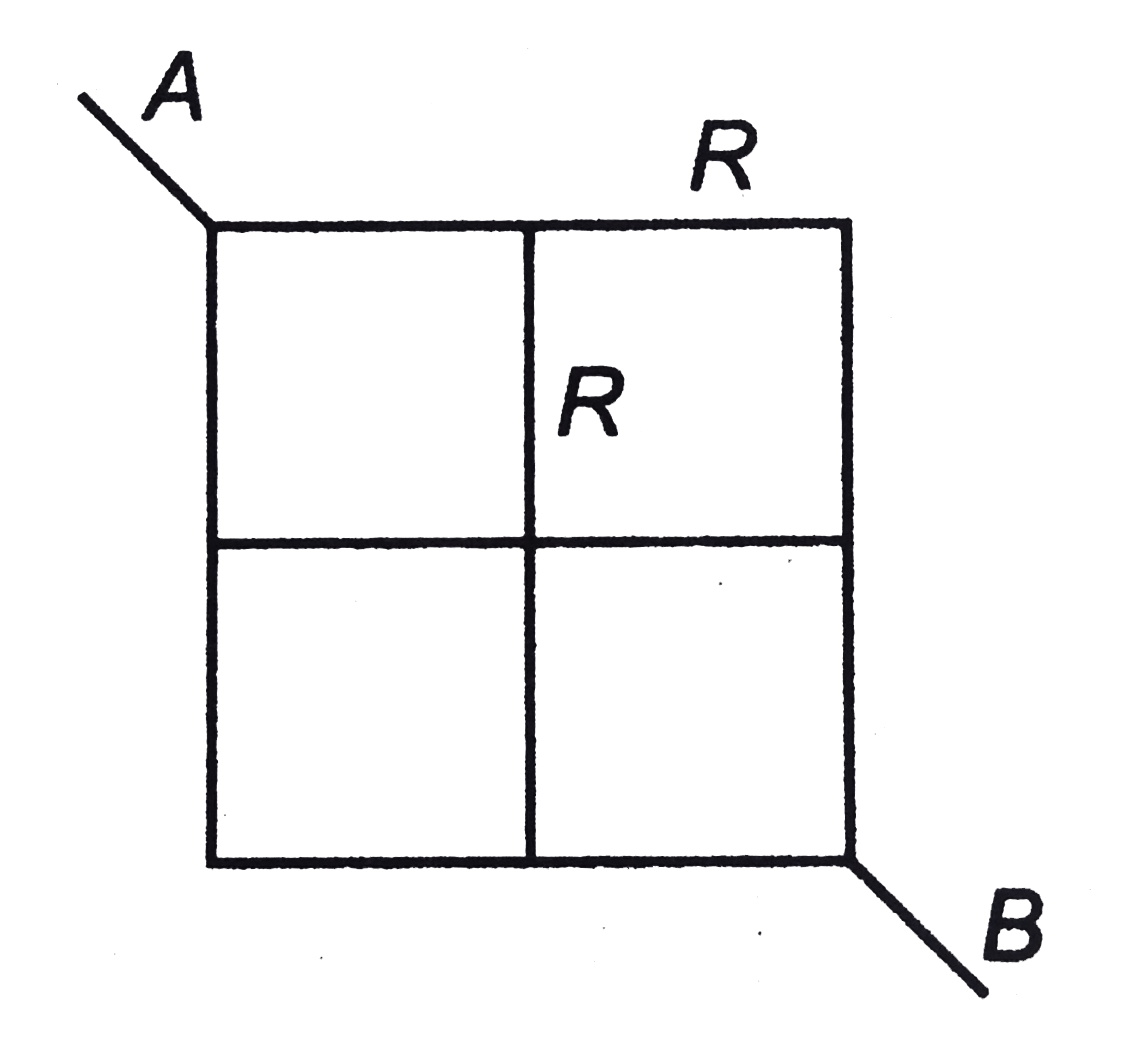
View SolutionA resistor circuit is constructed such that 12 resistor are arranged to form a cube as shown in fig. Each resistor has a resistance of 2Ω. The potential difference of 30V is applied across two of the opposing points as shown
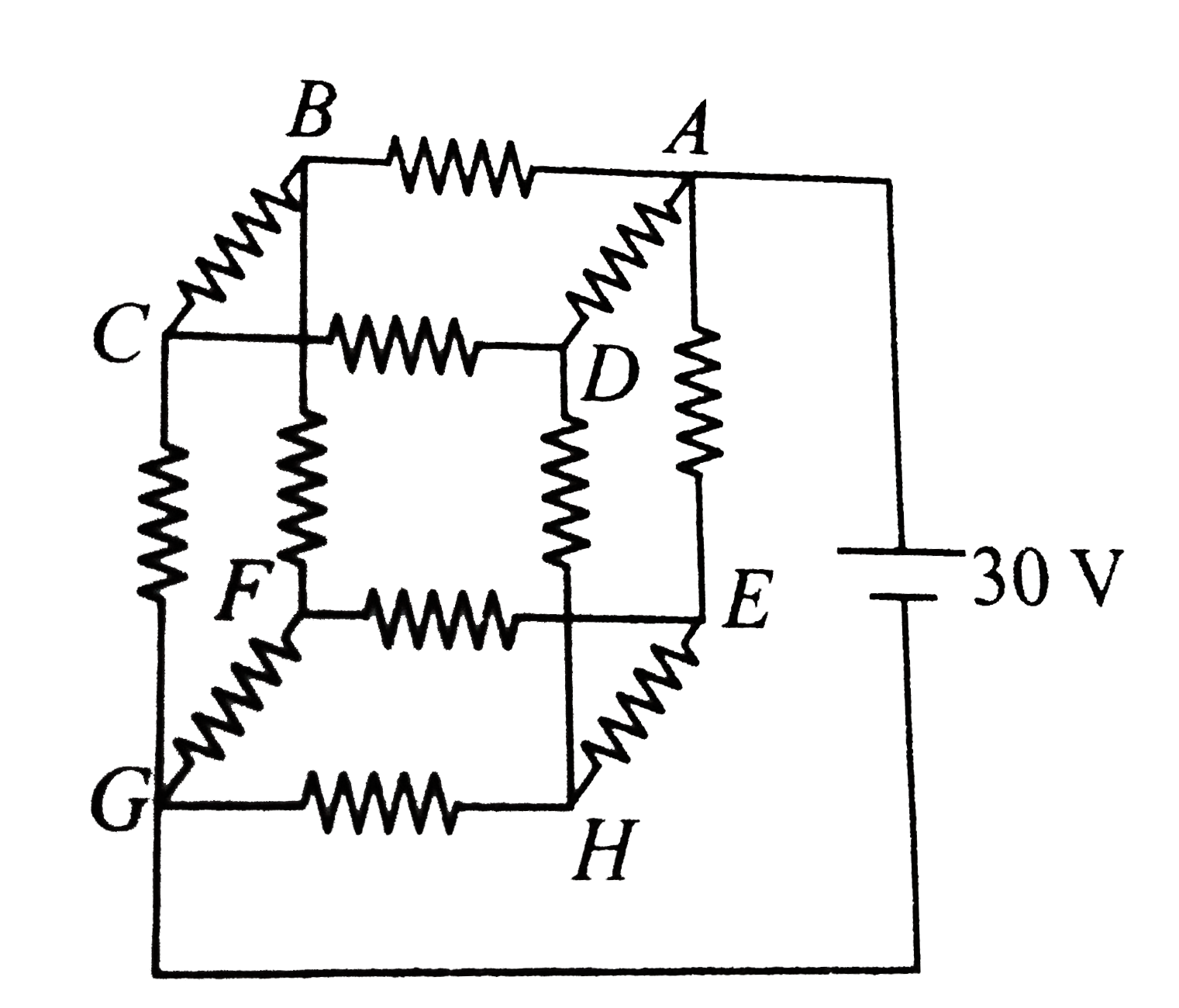
If we replace resistor between A and B and resistors between G and H by wires of zero resistance, then the points having the same potential are
i. D,E,C,F ,ii. A,B ,iii. G,H
View Solution
