A wire has a resistance R. What will be its resistace if (a) it is double on itself and (b) it is stretched so that (i) its length is doubled.

Video Solution

Text Solution

Verified by Experts

In case of a resistance wire,
$R = ρ \frac{L}{S} = ρ \frac{L}{π r^{2}} [a s S = π r^{2}]$
and in changing dimesions without any chage in mass volume
remsins constant $i . e ., V = V' or S L = S' L'$
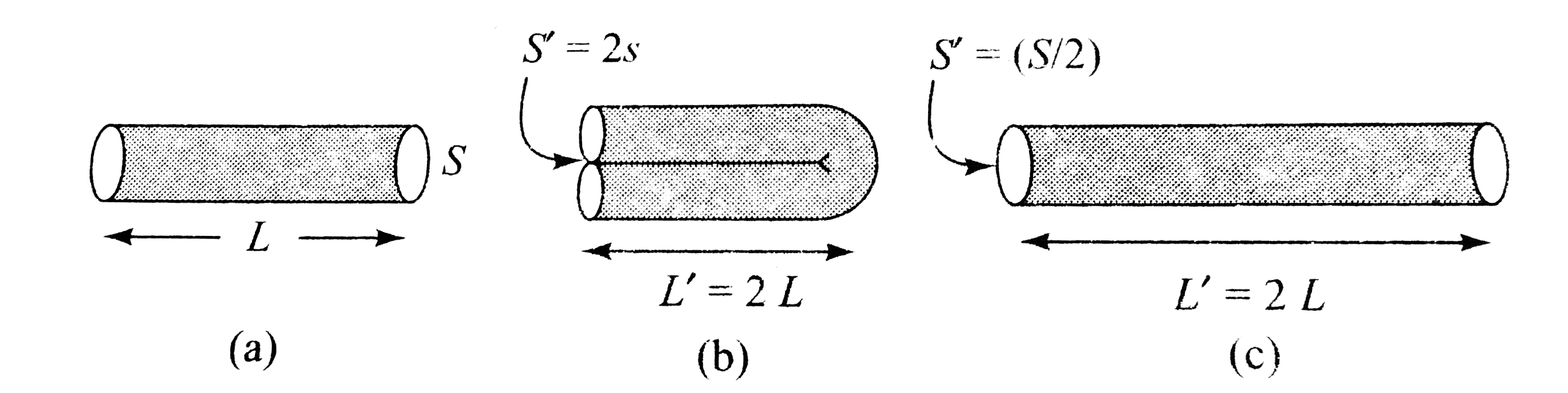
a. when the wire is doubled on itself (as shown in fig. S6 1(b),
its length will be halved, $i . e ., L' = (L / 2)$ .
$S \times L = S' \times (L / 2), i . e ., S' = 2 S$
$∴ R' = ρ [\frac{L'}{S'}] = ρ \frac{(L / 2)}{2 S} = \frac{1}{4} ρ \frac{L}{S} = \frac{1}{4} R$
,
b. When the length of the wire is doubled, i.e., $L' = 2 L < b r > S L = 2 L S', i . e ., S' = S / 2$
$R' = ρ [\frac{L}{S}] = ρ (\frac{2 L}{S / 2}) = 4 ρ [\frac{L}{S}] = 4 R$
ii. when the radis of the wire is halved, i.e., $r' = r / 2$ ,
$S' = π r^{2} = π {(r / 2)}^{2} = π r^{2} / 4 = S / 4$
And hence from $S L = S' L', i . e ., S L = (S / 4) L'$ ,
we have $L' = 4 L, R' = ρ \frac{L}{S} = ρ \frac{4 L}{S / 4} = 16 ρ \frac{L}{S} = 16 R$

Updated on:21/07/2023

If a wire is stretched to its length, then
AStress is double of Young's modulus
BStrain is unity
CYoung's modulus is equal to the half of the elastic energy per unit volume
DIts radius is halved
When a wire is stretched to double its length, then
AStrain is unity
BStress is equal to strain
CIts radius is halved
DYoung's modulus is equal to twice the elastic energy per unit volume
A resistance wire has a resistance R. Half of this wire is stretched to double its length and half is twisted so double its thickness, then its new resistance becomes
A $\frac{17 R}{8}$
B $\frac{17 R}{16}$
C $\frac{65 R}{32}$
D $\frac{65 R}{16}$