When switch S is thrown to the left in figure, the plates of capacitor 1 acquire a potential
difference V0. Capacitors 2 and 3 are initially uncharged. The switch is now thrown to the right.
What are the final charges q1,q2 and q3 on the capacitors?
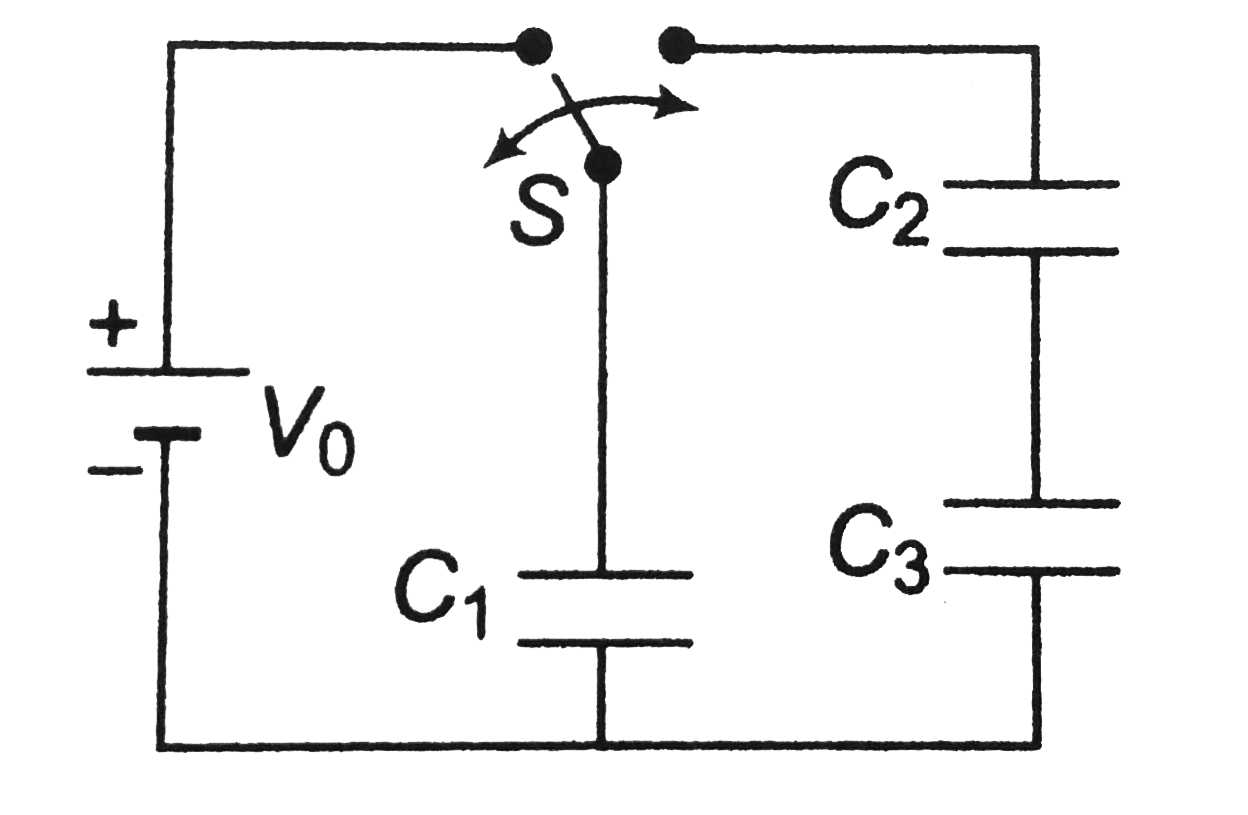
The correct Answer is:q1=C21V(C2+C3)C1C2+C2C3+C1C3,q2=q3=C2C3C1CVC1C2+C1C2+C2C3.
When switch is left
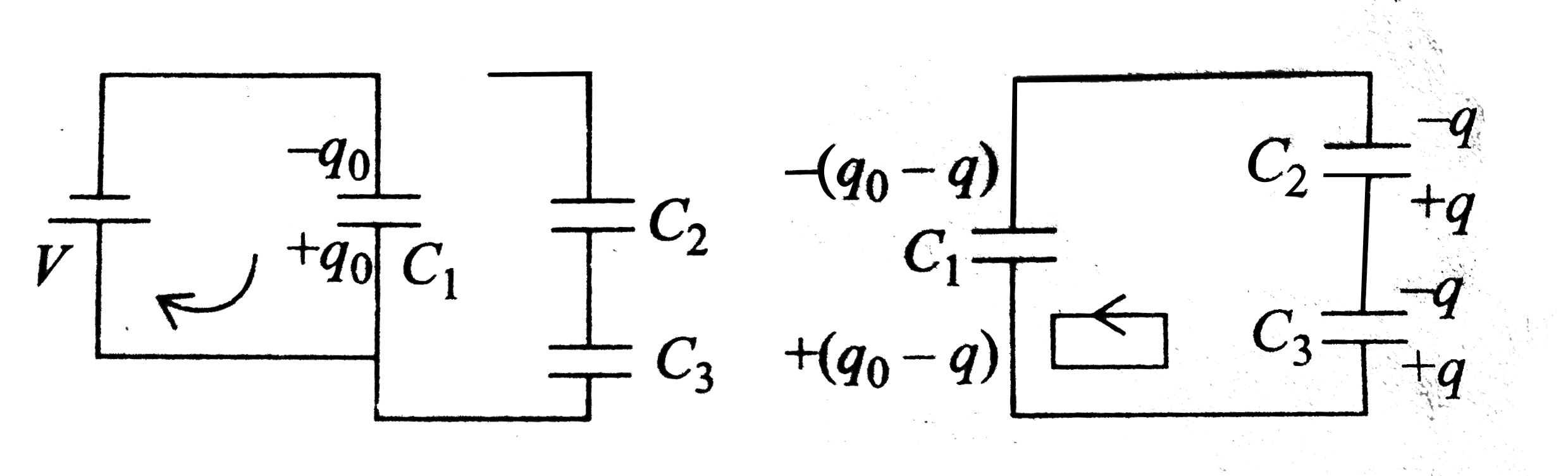 .
.
Appling loop rule:
q0−qC1−qC3−qC2=0
or q0C1−<br>WhenswitchisthrownrightAord∈g→l∞pre–,<br>(q_(0)-q)/C_(1)=q(1/C_(2)+1/C_(3))=2((C_(2)+C_(3))/(C_(2)C_(3)))<br>:. q=((C_(2)C_(3)q_(0))/(C_(1)C_(2)+C_(1)C_(3)+C_(2)C_(3)))<br>:. q1=q0−q=C1V−(C2C3q0C1C2+C1C3+C2C3)
=C21V(C2+C3)C1C2+C2C3+C1C3
Also, q2=q3=q=C2C3q0C1C2+C2C3+C1V3
=C2C3C1VC1C2+C1C3+C2C3.
Topper's Solved these Questions
- CAPACITOR AND CAPACITANCEBook:CENGAGE PHYSICSChapter:CAPACITOR AND CAPACITANCEExercise:Single Correct
Explore 49 Videos
- CAPACITOR AND CAPACITANCEBook:CENGAGE PHYSICSChapter:CAPACITOR AND CAPACITANCEExercise:Multile Correct
Explore 11 Videos
- CAPACITOR AND CAPACITANCEBook:CENGAGE PHYSICSChapter:CAPACITOR AND CAPACITANCEExercise:Exercise 4.2
Explore 32 Videos
- ATOMSBook:CENGAGE PHYSICSChapter:ATOMSExercise:QUESTION BANK
Explore 40 Videos
- CENGAGE PHYSICS DPPBook:CENGAGE PHYSICSChapter:CENGAGE PHYSICS DPPExercise:subjective type
Explore 51 Videos
Similar Questions
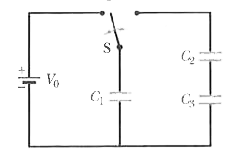
Figure 25-27 displays a 16.0 V battery and 3 uncharged capacitors of capacitances C1=4.00μF,C2=6.00μFandC3=3.00μF The switch is thrown to the left side until capacitor 1 is fully charged. Then the switch is thrown to the right. What is the final charge on (a) capacitor 1, (b) capacitor 2 and (c ) capacitor 3?
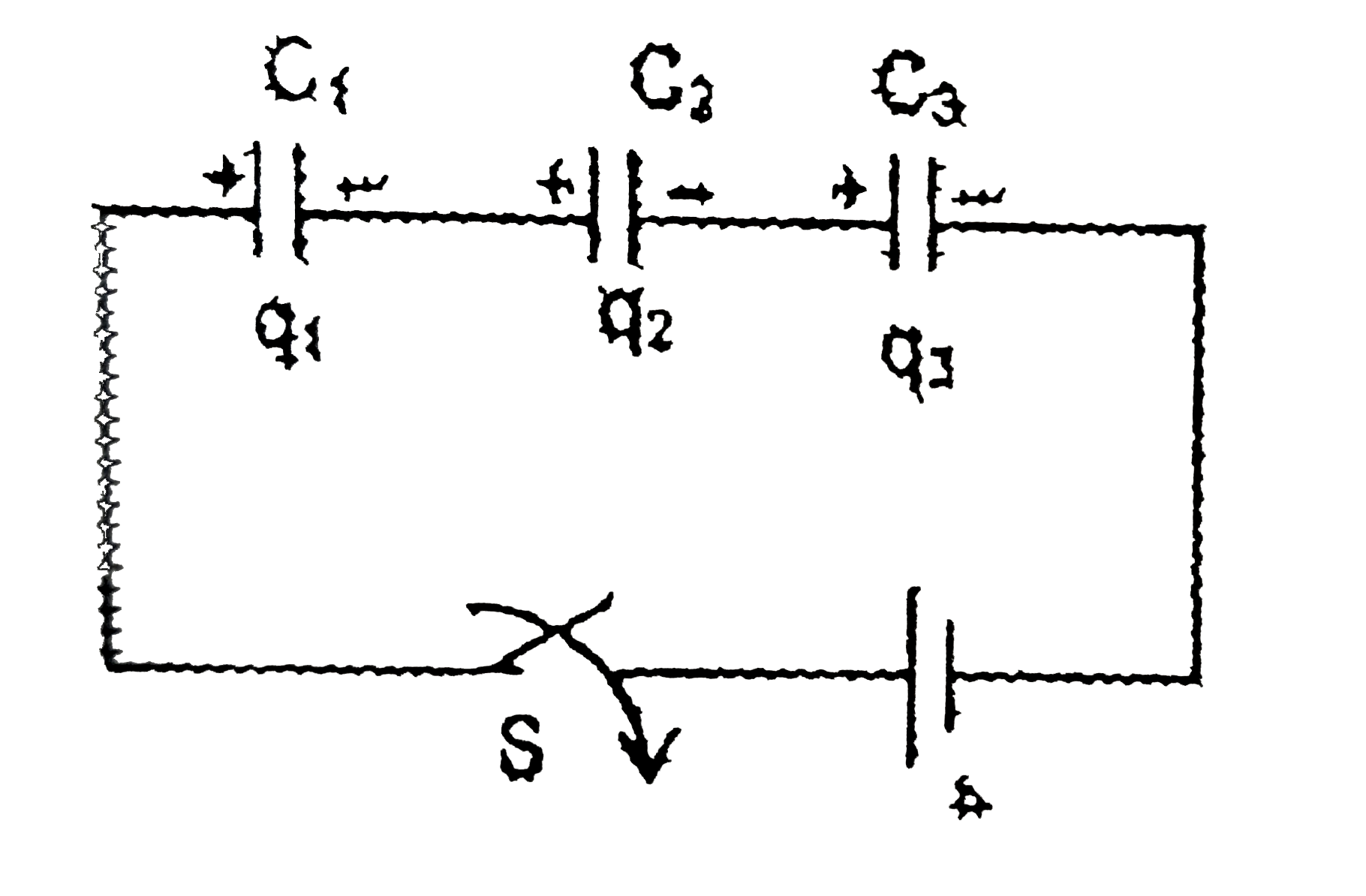
View SolutionCharges q1 , q2 and q3 are placed on capacitors of capacitance C1, C2 and C3, respectively, arranged in series as shown. Switch S is there closed. What are the final charges q'1, q'2 and q'3 on the capacitors?
Given q1=30μC, q2=μC, q3=10μC, C1=10μF, C2=20μF, C3=30μF and ε=12volt
View Solution
Knowledge Check
In the given figure switch is open initially and capacitor C2 is unchanged
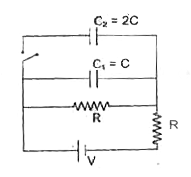
Potential difference on C1just after closing the switch isAV3BV6CV4DV2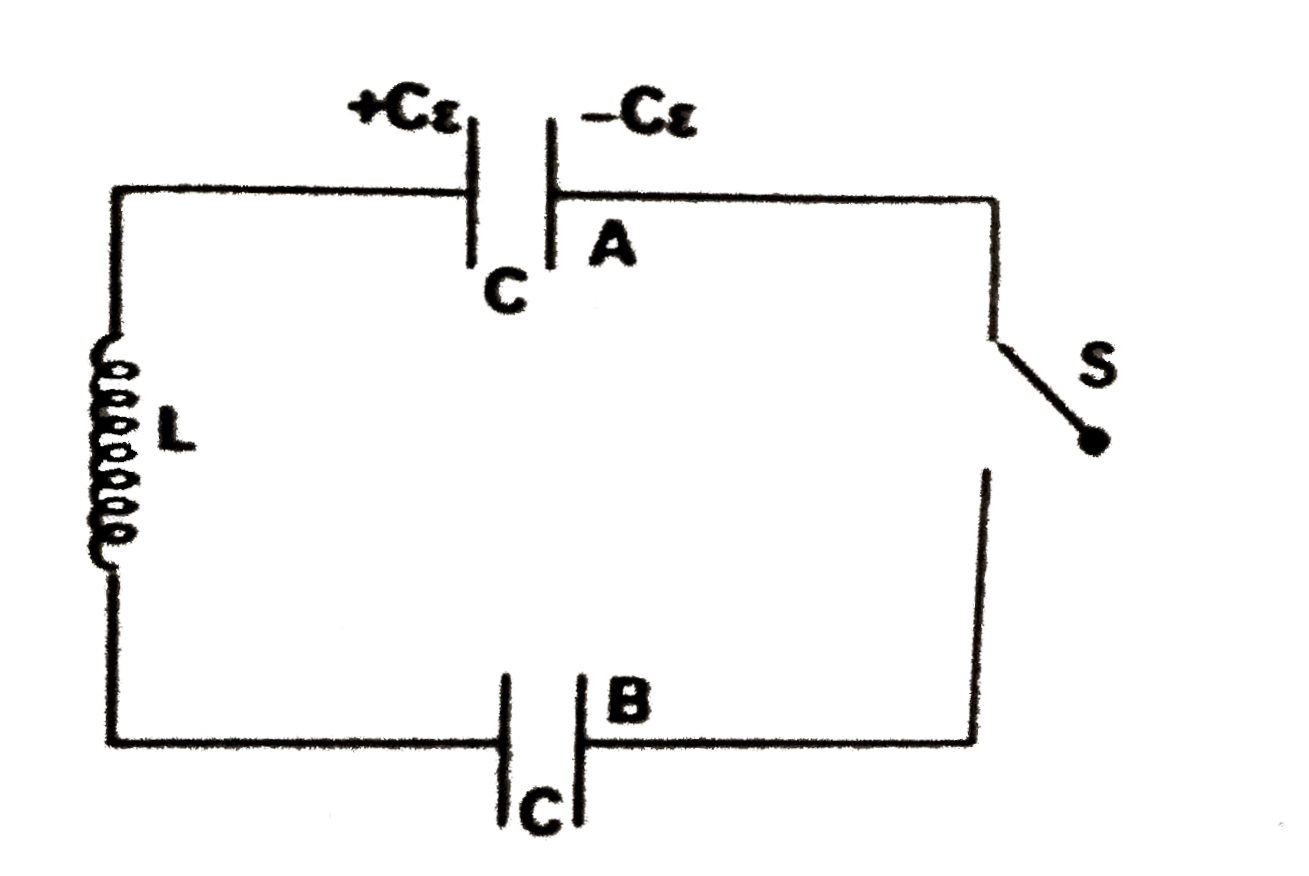
Initially capacitor 'A' is charged to a potential drop ε and capacitor B is uncharged At t=0, switch S is closed, then the maximum current through the inductor isAε√C2LBε√CLC2ε(CL)Dε2√CLA capacitor of capacitance C is charged to a potential difference V from a cell and then disconnected from it. A charge +Q is now given to its positive plate. The potential difference across the capacitor is now
AVBV+QCCV+Q2CDV−QC, if V<CV
The plates of a capacitor of capacitance C are given the charges Q1andQ2 as shwon in figure. Now the switch is closed at t =0. Find the charges on plates after time t.
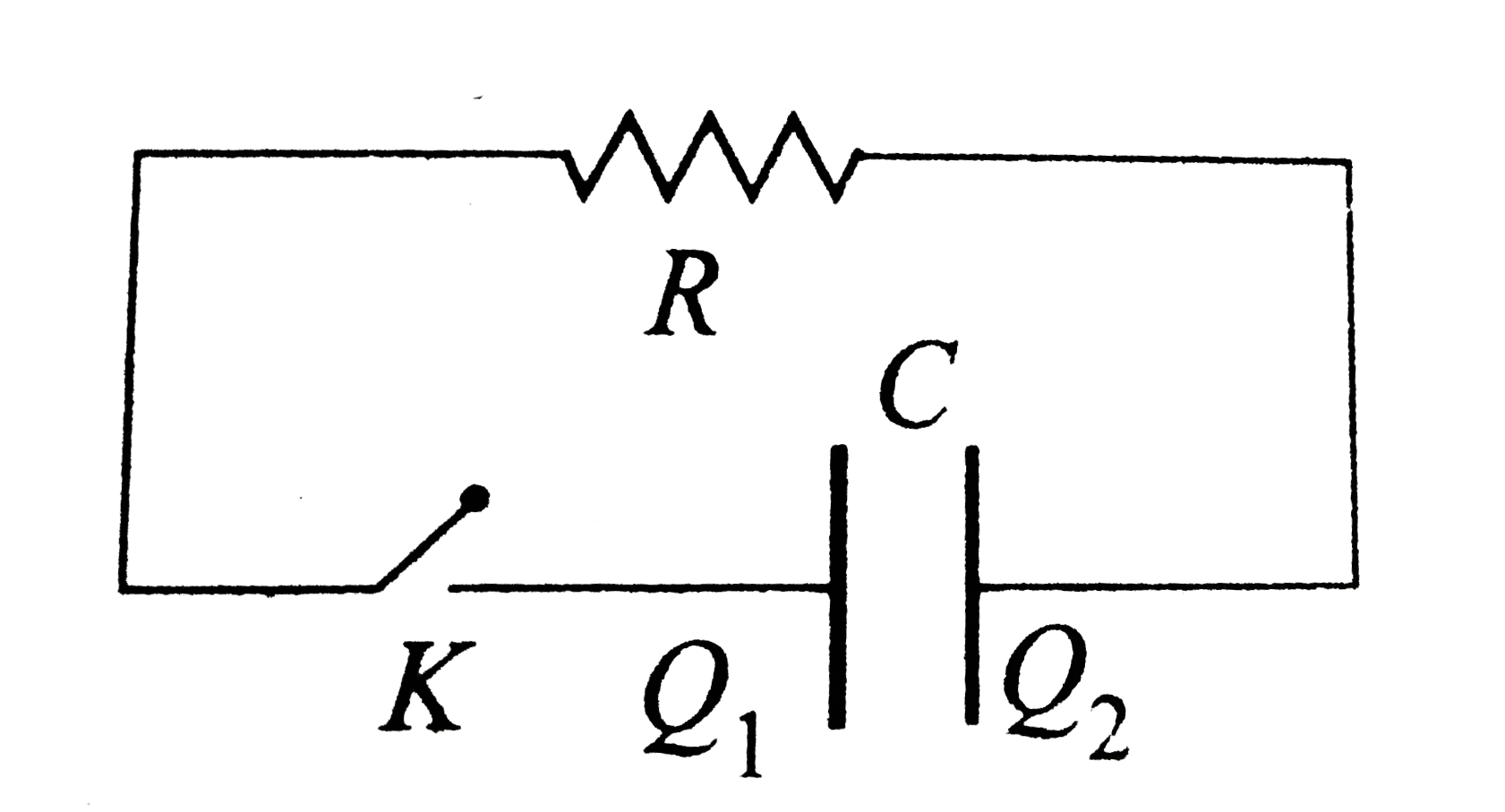
View SolutionA battery is used to charge a parallel plate combination of two identical capacitors. If the potential difference across the battery is V and the total charge Q flows through the battery during the charging process then 1) The charge on each capacitor is Q/2 and the potential of each capacitor is V/2 2) The charge on each capacitor is Q and the potential of each capacitor is V 3) The charge on each capacitor is Q/2 and the potential of each capacitor is V 4) The charge on each capacitor is Q and the potential of each capacitor is V/2
View SolutionA capacitor of capacitance C is charged to a potential difference V from a cell and then disconnected from it. A charge +Q is now given to its positive plate. The potential difference across the capacitor is now
View SolutionIf A is the area of each plate, charge on it is q and potential difference is V then the distance between the parallel plate capacitor is
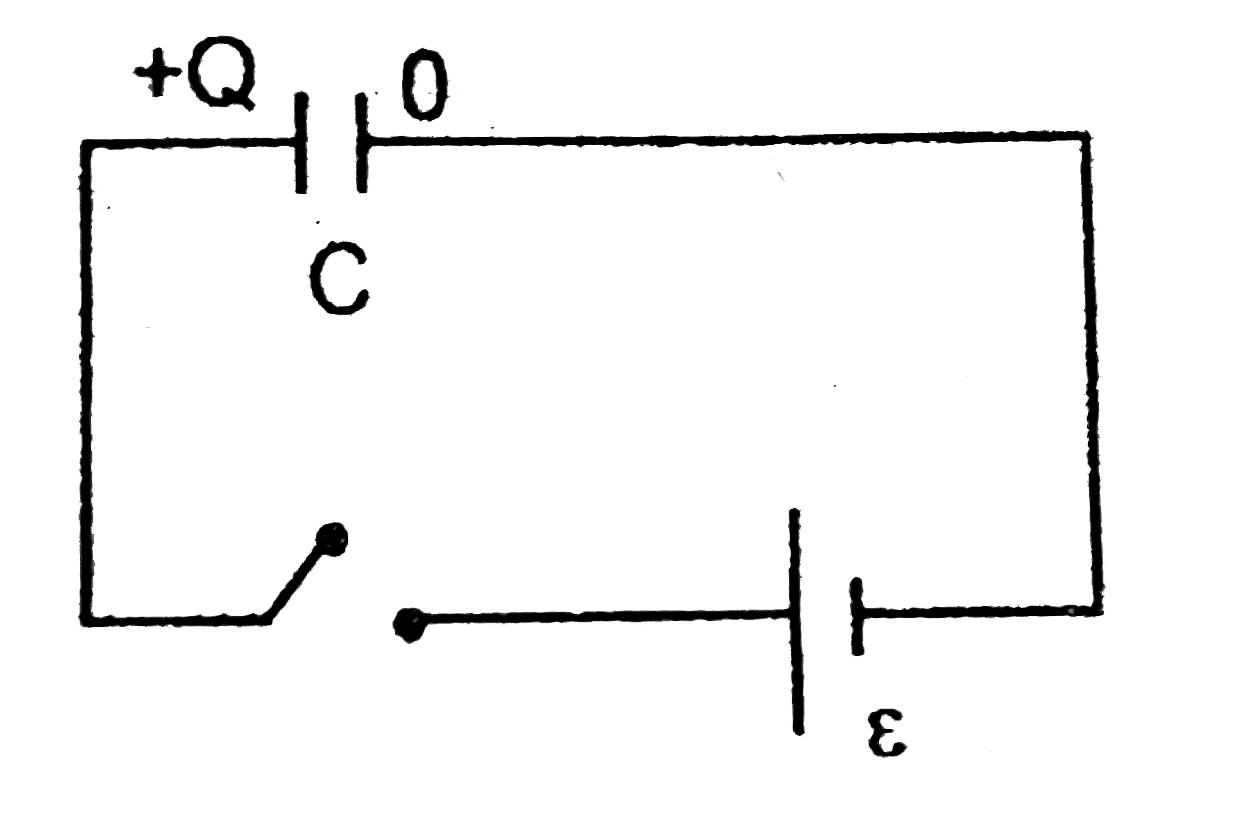
View SolutionThe left plate of the capacitor shown in the figure above carries a charge +Q while the right plate is uncharged at t=0. The total charge on the right plate after closing the switch will be:
View Solution
