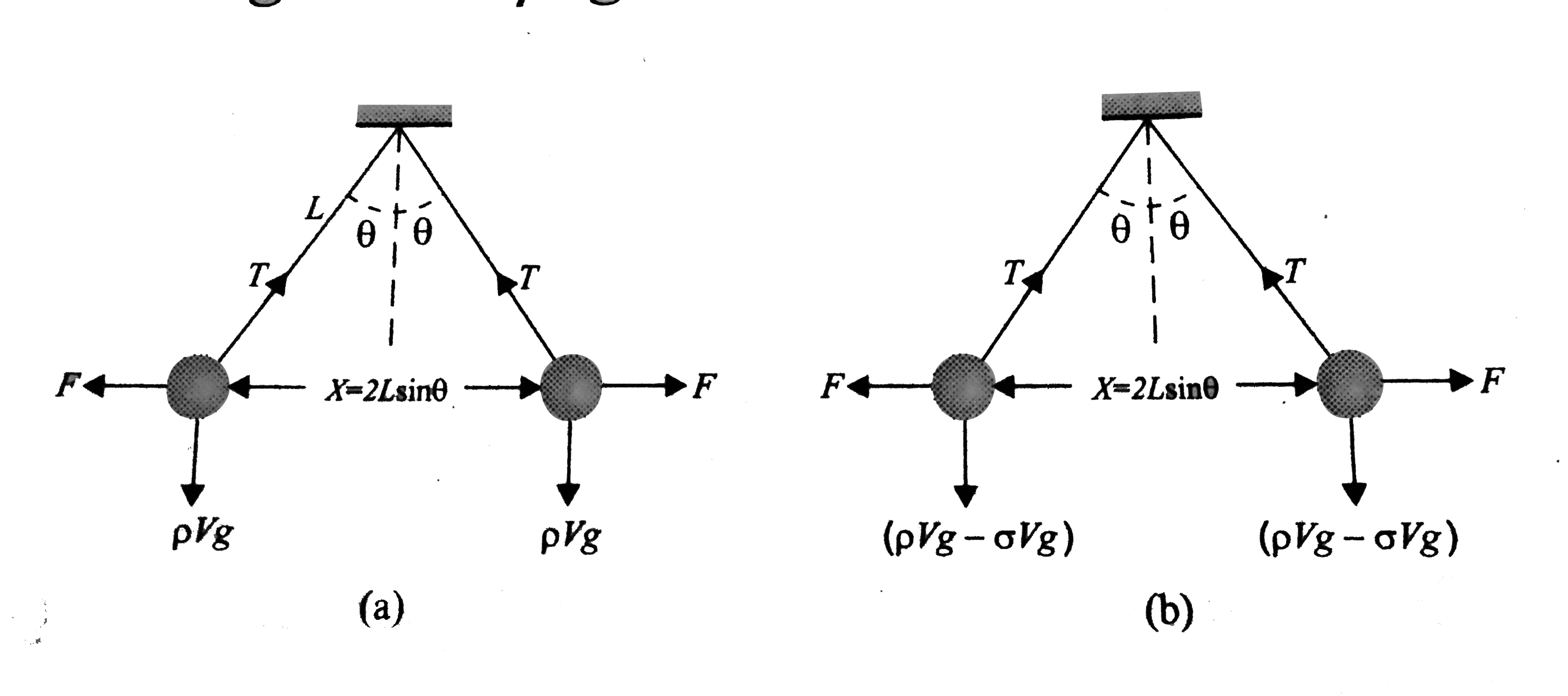
Two identical balls each having a density are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle with vertical. Now, both the balls are immersed in a liquid. As a result the angle does not change. The density of the liquid is . Find the dielectric constant of the liquid.
Let V be the volume of each ball, then the mass of each ball is .When the balls are in air, from the previous problem.
...(i)
 ,
,
when the balls are suspended in liquid, the Coulombic force is reduced to and apparent weight=weight -upthrust, i.e
According to the problem, angle is unchanged therefore.
...(ii)
From Eqs, (i) and (ii) we get
.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Solved examples
Explore 10 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Exercises
Explore 58 Videos
- COMMUNICATION SYSTEMBook:CENGAGE PHYSICSChapter:COMMUNICATION SYSTEMExercise:QUESTION BANK
Explore 19 Videos
- Current ElectricityBook:CENGAGE PHYSICSChapter:Current ElectricityExercise:QUESTION BANK
Explore 40 Videos
Similar Questions
Two identical ball having mass density ρ , mass m and charge q are suspended from a common point by two insulating massless strings of equal length. At equilibrium each string makes an angle theta with the vertical. Now both the balls are immersed in a fluid. At equilibrium, theta remains same. If the mass density of liquid is σ then dielectric constant of liquid will be
View SolutionTwo indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View Solution
Knowledge Check
Two small identical balls are suspened from a common point by two identical strings. When they are given identical charges, they move apar and the strings make with the vertical an angle of q. Now the system is immersed in a liquid and as a result the angle q does not change. If r is the density of the material of the balls and s is the density of the liquid, the dielectric constant of the liquid is
App−σBp−σpCσp−σDp−σσTwo identical tennis balls each having mass 'm' and charge 'q' are suspended from a fixed point by threads of length 'l'. What is the equilibrium separation when each thread makes a small angle 'θ' with the vertical ?
Ax=(q2l2πε0mg)1/2Bx=(q2l2πε0mg)1/3Cx=(q2l22πε0m2g)1/3Dx=(q2l22πε0m2g2)1/3Two path balls carrying eqaul chareges are suspended froom a common point by strings of equal length, the strings are rightly clamped at half the height. The equilibrium separation between the balls, now becomes :
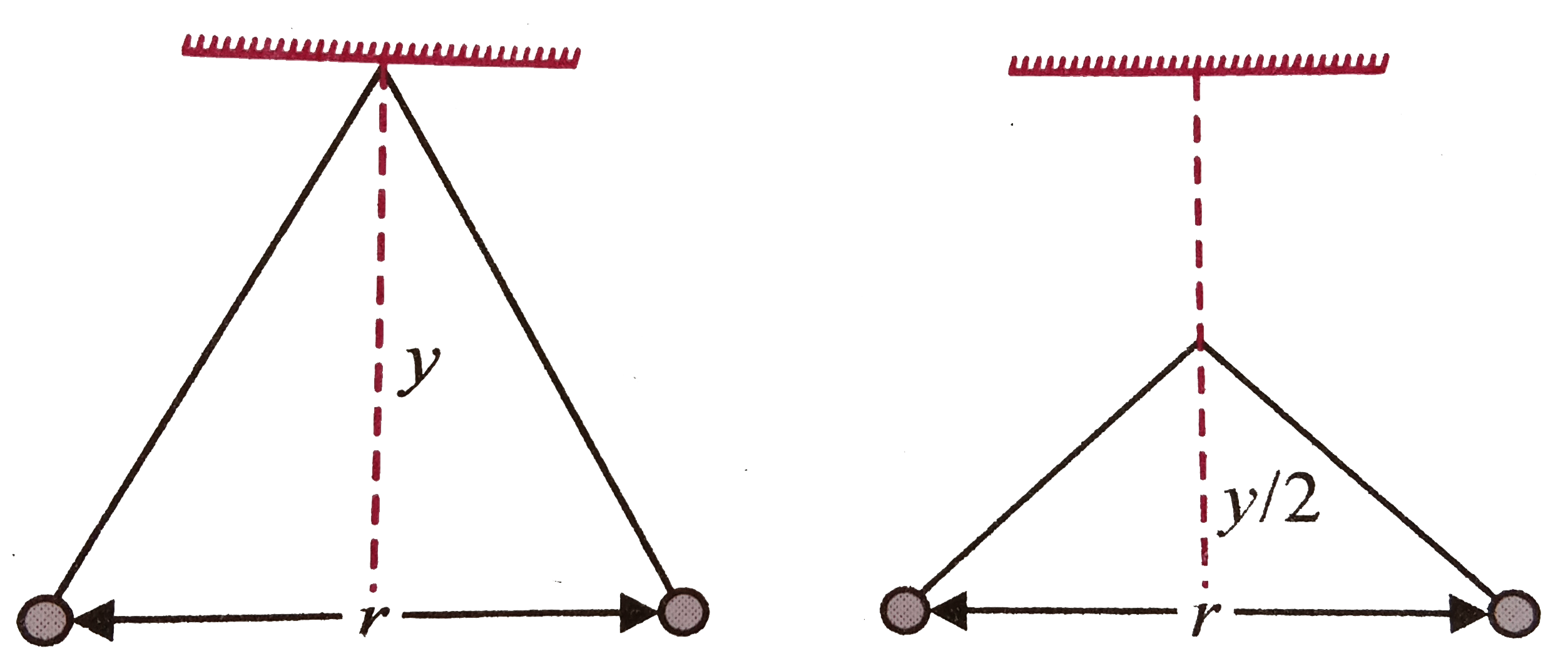 A(2r3)B(1√2)2C(r3√2)D(2r√3)
A(2r3)B(1√2)2C(r3√2)D(2r√3)
Two small spheres, each having a mass of 20 g, are suspended form a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the speration between the balls at equilibrium is found to be 4 cm . Find the charge on each sphere.
View SolutionTwo identical balls , each having a charge q and mass m , are suspended from a common point by two insultating strings each of length L. The balls are held at a separation x and then released. Find
(a) the electric force on each ball
(b) the component of the resultant force on a ball along and perpendicular to string
(c ) the tension in the string
(d) the acceleration of one of the balls. Consider the situation only for the instant just after the release.
View SolutionTwo pitch balls carrying equal charges are suspended from a common point by strings of equal length, the equilibrium separation between them is r. Now the strings are rigidly clamped at half the height. The equilibrium separation between the balls now become
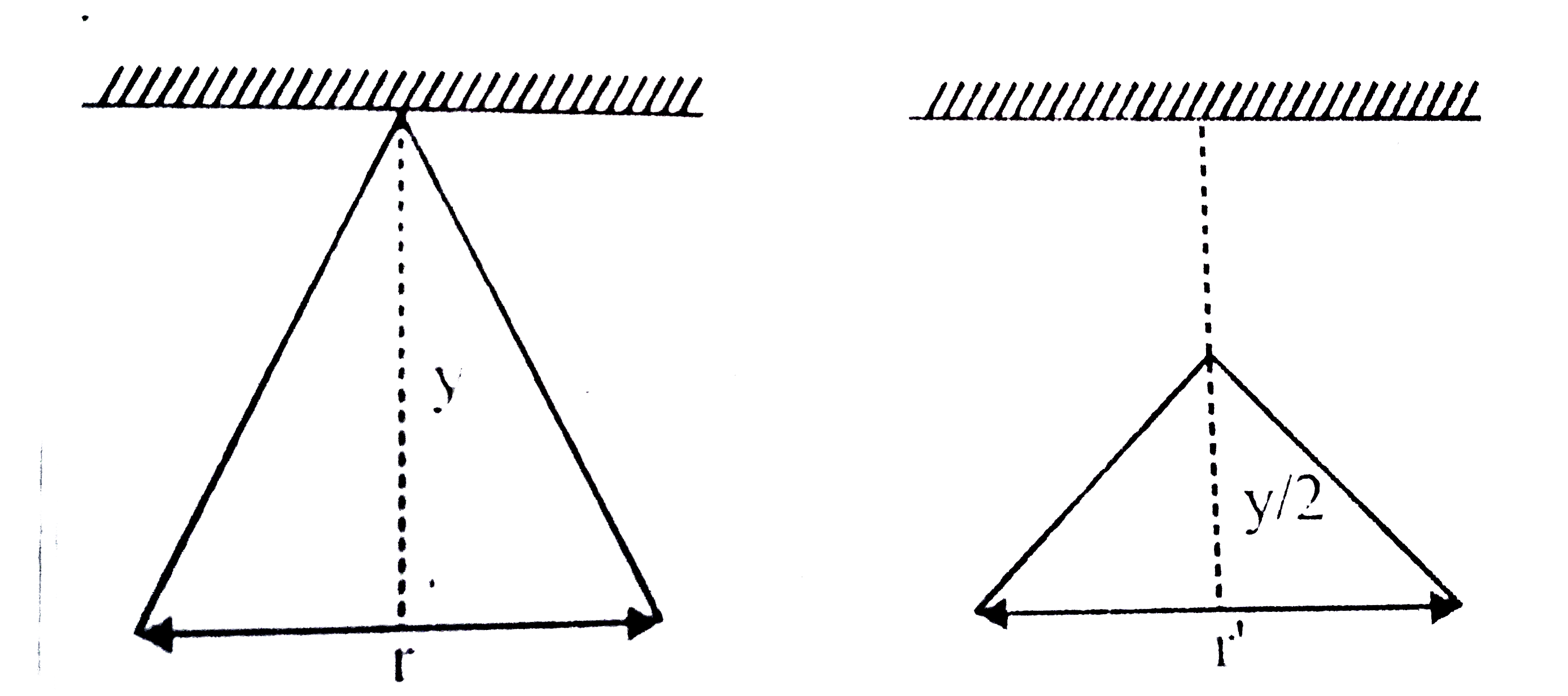
View SolutionTwo identical balls having equal positive charge q coulomb are suspended by two in painting strings of equal length. What would be the effect on the force when a plastic sheet is inserted between the two?
View SolutionTwo small equal point charges of magnitude q are suspended from a common point on the ceiling by insulating mass less strings of equal lengths. They come to equilibrium with each string making angle θ from the vertical. If the mass of each charge is m, then the electrostatic potential at the centre of line joining the will be (14πεlion0=k)
View Solution
