Two small equal point charges of magnitude q are suspended from a common point on the ceiling by insulating mass less strings of equal lengths. They come to equilibrium with each string making angle θ from the vertical. If the mass of each charge is m, then the electrostatic potential at the centre of line joining the will be (14πεlion0=k)
2√km>anθ
√kmgtanθ
4√kmg/tanθ
3√kmg/tanθ
The correct Answer is:C
To solve the problem, we need to find the electrostatic potential at the center of the line joining two equal point charges suspended from a common point. Here’s a step-by-step solution:
Step 1: Understand the Setup
We have two equal point charges q suspended from a common point by massless strings, making an angle θ with the vertical. Each charge has a mass m.
Step 2: Analyze Forces Acting on the Charges
When the charges are in equilibrium, the forces acting on each charge include:
- The gravitational force mg acting downward.
- The tension T in the string acting along the string.
The tension can be resolved into two components:
- Vertical component: Tcosθ
- Horizontal component: Tsinθ
Step 3: Set Up Equations for Equilibrium
For vertical equilibrium:
Tcosθ=mg(1)
For horizontal equilibrium, the electrostatic force F between the two charges must balance the horizontal component of the tension:
F=Tsinθ(2)
Step 4: Calculate the Electrostatic Force
The electrostatic force F between the two charges q separated by a distance r is given by Coulomb's law:
F=14πϵ0q2r2
Step 5: Relate Distance r to Angle θ
The distance r between the two charges can be expressed in terms of the angle θ and the length of the strings L:
r=2Lsinθ
Step 6: Substitute r into the Electrostatic Force Equation
Substituting r into the electrostatic force equation gives:
F=14πϵ0q2(2Lsinθ)2=14πϵ0q24L2sin2θ=q216πϵ0L2sin2θ
Step 7: Equate Forces from Equations (1) and (2)
From equations (1) and (2), we have:
Tsinθ=q216πϵ0L2sin2θ
Substituting T from equation (1):
mgcosθsinθ=q216πϵ0L2sin2θ
This simplifies to:
mgtanθ=q216πϵ0L2sin2θ
Step 8: Solve for q2
Rearranging gives:
q2=16πϵ0L2mgtanθsin2θ
Step 9: Calculate the Electrostatic Potential V
The electrostatic potential V at the center of the line joining the two charges is given by:
V=k(qr1+qr2)
Since r1=r2=r2:
V=2kqr2=4kqr
Step 10: Substitute r and q
Substituting r=2Lsinθ and q2 from earlier:
V=4kq2Lsinθ=2kqLsinθ
Final Expression for Electrostatic Potential
Substituting q from the earlier expression:
V=2k√16πϵ0L2mgtanθsin2θLsinθ
Conclusion
Thus, the electrostatic potential at the center of the line joining the two charges is given by:
V=4√kmgtanθ
We have two equal point charges q suspended from a common point by massless strings, making an angle θ with the vertical. Each charge has a mass m.
When the charges are in equilibrium, the forces acting on each charge include:
- The gravitational force mg acting downward.
- The tension T in the string acting along the string.
- Vertical component: Tcosθ
- Horizontal component: Tsinθ
For vertical equilibrium:
Tcosθ=mg(1)
F=Tsinθ(2)
The electrostatic force F between the two charges q separated by a distance r is given by Coulomb's law:
F=14πϵ0q2r2
The distance r between the two charges can be expressed in terms of the angle θ and the length of the strings L:
r=2Lsinθ
Substituting r into the electrostatic force equation gives:
F=14πϵ0q2(2Lsinθ)2=14πϵ0q24L2sin2θ=q216πϵ0L2sin2θ
From equations (1) and (2), we have:
Tsinθ=q216πϵ0L2sin2θ
Substituting T from equation (1):
mgcosθsinθ=q216πϵ0L2sin2θ
This simplifies to:
mgtanθ=q216πϵ0L2sin2θ
Rearranging gives:
q2=16πϵ0L2mgtanθsin2θ
The electrostatic potential V at the center of the line joining the two charges is given by:
V=k(qr1+qr2)
Since r1=r2=r2:
V=2kqr2=4kqr
Substituting r=2Lsinθ and q2 from earlier:
V=4kq2Lsinθ=2kqLsinθ
Substituting q from the earlier expression:
V=2k√16πϵ0L2mgtanθsin2θLsinθ
Thus, the electrostatic potential at the center of the line joining the two charges is given by:
V=4√kmgtanθ
Topper's Solved these Questions
- BOOK - DISHA PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE 2: CONCEPT APPLICATOR24videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - DISHA PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE 2: CONCEPT APPLICATOR24videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - DISHA PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise - 2 : Concept Applicator30videos
ELECTROMAGNETIC WAVES
- BOOK - DISHA PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - EXERCISE-230videos
GRAVITATION
Similar Questions
Two identical ball having mass density ρ , mass m and charge q are suspended from a common point by two insulating massless strings of equal length. At equilibrium each string makes an angle theta with the vertical. Now both the balls are immersed in a fluid. At equilibrium, theta remains same. If the mass density of liquid is σ then dielectric constant of liquid will be
View SolutionTwo identical balls each having a density ρ are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle θ with vertical. Now, both the balls are immersed in a liquid. As a result the angle θ does not change. The density of the liquid is σ. Find the dielectric constant of the liquid.
View Solution
Knowledge Check
- Question 1 - Select One
Two positively charged sphere of masses m1 and m2 are suspended from a common point at the ceiling by identical insulating massless strings of length l. Charges on the two spheres are q1 and q2 respectively. Equilibrium both strings make the same angle θ with the vertical. then
Aq1m2=q2m2Bm1=m2Cm1=m2sinθDq2m1=q1m2 - Question 2 - Select One
Two positively charged spheres of masses m1, and m2 are suspended from a common point at the ceiling by identical insulating massless strings of length l. Charged on the two spheres are q1 and q2, respectively. At equilibrium both strings make the same angle θ with the vertical. Then
Aq1m1=q2m2Bm1=m2Cm1=m2sinθDq2m1=q1m2 - Question 3 - Select One
Two identical tennis balls each having mass 'm' and charge 'q' are suspended from a fixed point by threads of length 'l'. What is the equilibrium separation when each thread makes a small angle 'θ' with the vertical ?
Ax=(q2l2πε0mg)1/2Bx=(q2l2πε0mg)1/3Cx=(q2l22πε0m2g)1/3Dx=(q2l22πε0m2g2)1/3
Two indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View SolutionTwo identical spheres of mass m and charge q are suspended from a common point by two threads of the same length l and it is found that threads make an angle θ with the vertical when the spheres are in equilibrium . Calculate the value of q.
View SolutionThree paricles, each of mass m and carrying a charge q each, are suspended from a common pointby insulating massless strings each of length L. If the particles are in equilibrium and are located at the corners of an equilateral triangle of side a, calculate the charge q on each particle. Assume L>>a.
View SolutionTwo pitch balls carrying equal charges are suspended from a common point by strings of equal length, the equilibrium separation between them is r. Now the strings are rigidly clamped at half the height. The equilibrium separation between the balls now become
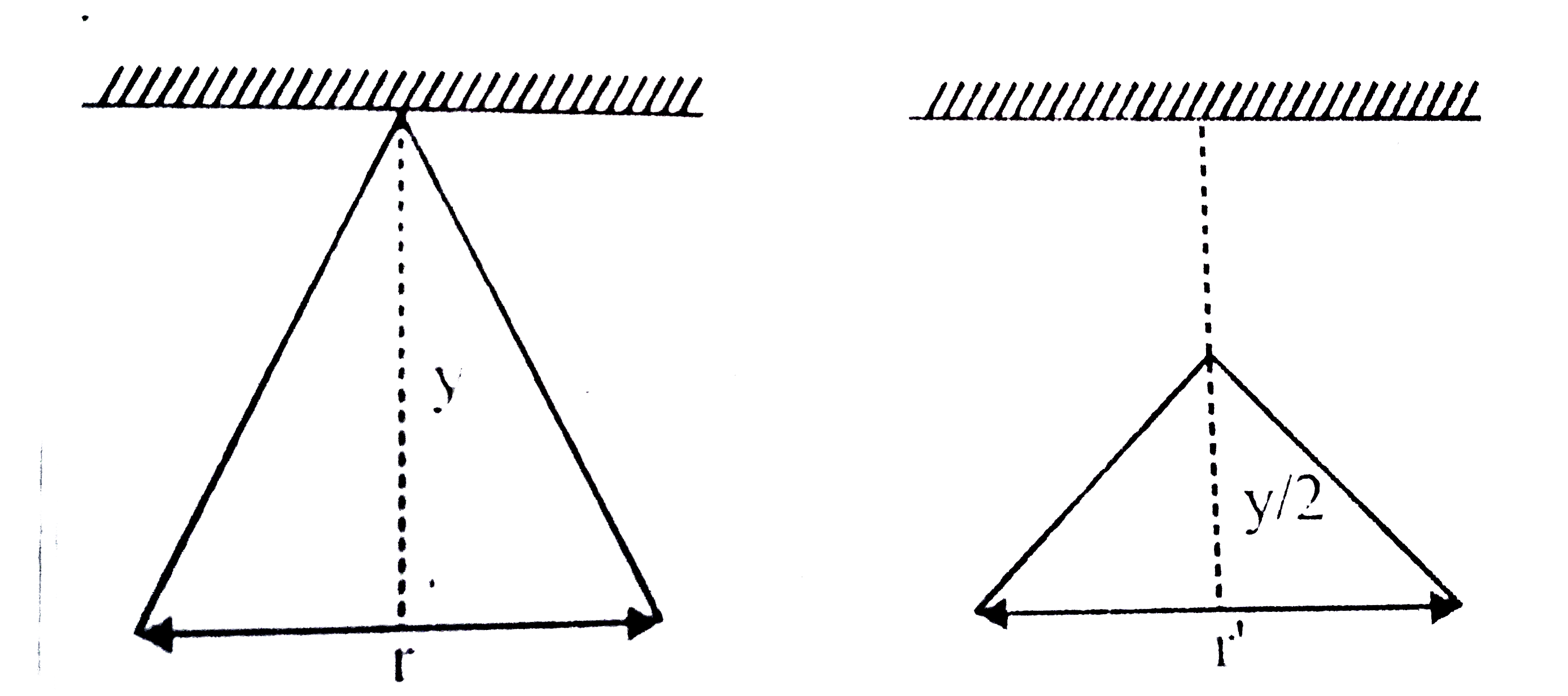 View Solution
View SolutionTwo small conducting spheres each of mass 9×10−4kg are suspended from the same point by non conducting strings of length 100cm,They are given equal and similar charges until the strings are equally inclined at 45∘ each to the vertical,The charge on each spehre is ..........
View Solution
