Two particles move on a circular path (one just inside and the other just outside) with the angular velocities $ω$ and $5 ω$ starting from the same point. Then

the cross each other at regular intervals of time $2 π / 4 ω$ when their angular velocities are oppositely directed

they cross each other at point on the path subtending an angle of $60^{\circ}$ at the centre if their angular velocities are oppositely directed

the cross at intervals of time $π / 3 ω$ if their angular velocities are oppositely directed

they cross each other at points on the path subtendingf $90$ at the centre if their angular velocities are in the same sence

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B, C, D

If the velocities are oppositely directed,

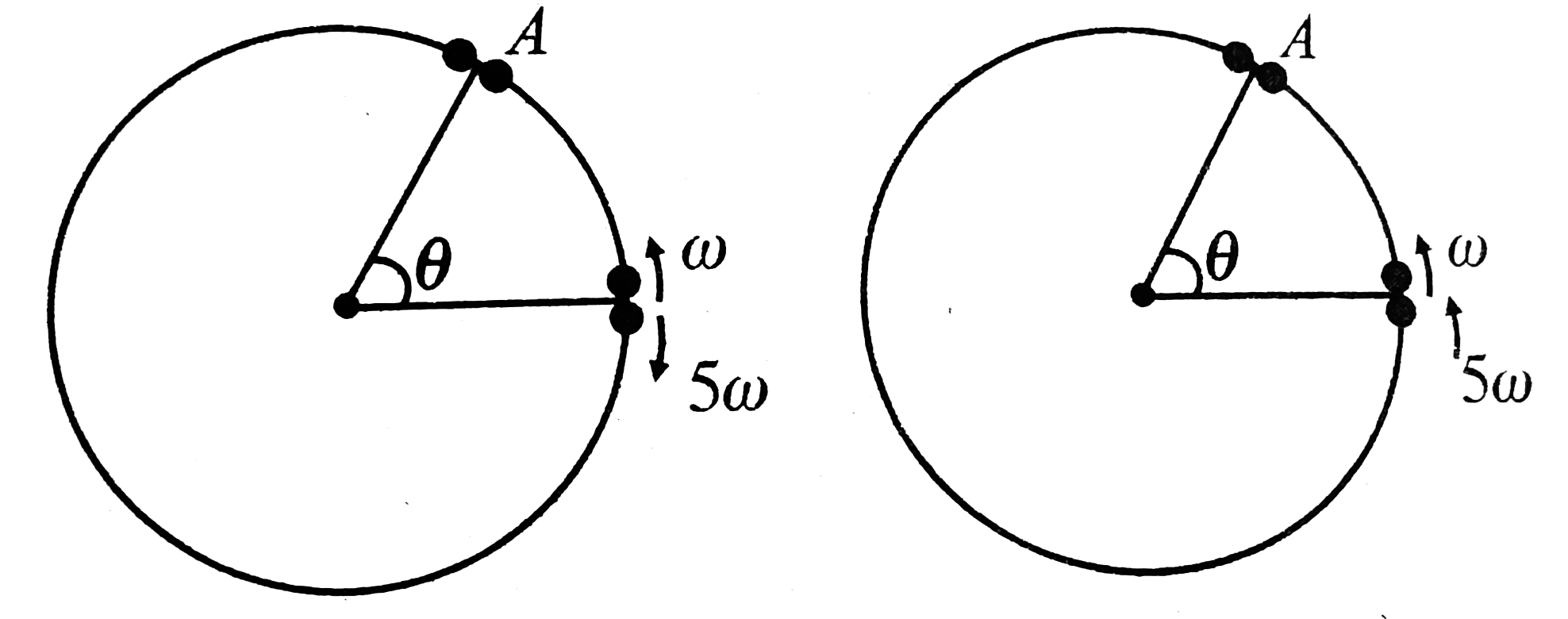
Let they cross A at time t, then
$θ = ω t, 2 π - θ = 5 ω t \Rightarrow 2 π = 6 ω t \Rightarrow t = π / 3 ω$
$θ = ω t, \frac{ω π}{3 ω} = \frac{π}{3} = 60^{\circ}$
If angular velocities are in same sense:
$θ = ω t, 2 π + θ = 5 s, \Rightarrow θ = π / 2$

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One or More
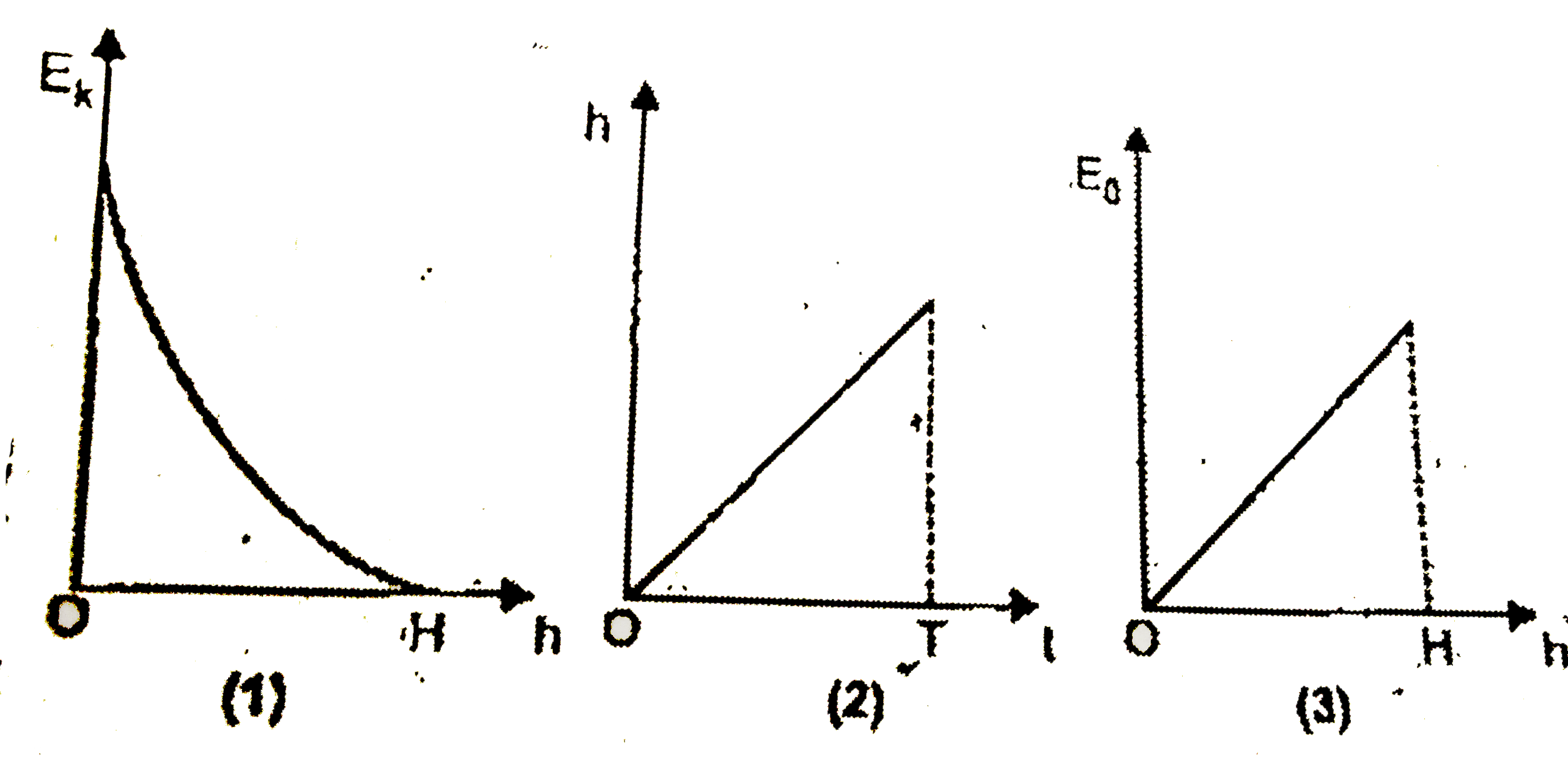
A ball is projected vertically upwards. Air resistance and variation in g may be neglected. The ball rises to its maximum height H in a time T, the height being h after a time t :
(1) The graph of kinetic energy $E_{k}$ of the ball against height h is shown in figure 1
(2) The graph of height h against time t is shown in figure 2
(3) The graph of gravitational energy $E_{k}$ of the ball against height h is shown in figure 3
(1)
Two particles move on a circular path ( one just inside and the other just outside) with angular velocities ro and $5 ω$ starting from the same point. Then :
Athey cross each other at regular intervals of time $\frac{2 π}{4 ω}$ when their angular velocities are oppositely directed
Bthey cross each other at points on the path subtending an angle of $60^{\circ}$ at the centre if their angular velocities are oppositely directed
Cthey cross at intervals of time $\frac{π}{3 ω}$ if their angular velocities are oppositely directed
Dthey cross each other .at points on the path subtending $90^{\circ}$ at the centre if their angular velocities are in the same sense
Question 2 - Select One
A particle moves in a circular path of radius 0.5 m with a linear speed of 2 $m s^{- 1}$ ,its angular speed is
A $2 r a d s^{- 1}$
B $3 r a d s^{- 1}$
C $4 r a d s^{- 1}$
DNone of these
Question 3 - Select One
A particle moving along a circule path of radius 'r' with uniform angular velocity $ω$ . Its angular acceleration is
A $r ω^{2}$
B $r / ω$
Czero
D $ω / r$

CENGAGE PHYSICS-MISCELLANEOUS VOLUME 2-MCQ_TYPE

Home
Ask Doubt
Profile

Two particles move on a circular path (one just inside and the other just outside) with the angular velocities ω and 5ω starting from the same point. Then

The correct Answer is:B, C, D

If the velocities are oppositely directed, Let they cross A at time t, then θ=ωt,2π−θ=5ωt⇒2π=6ωt⇒t=π/3ω θ=ωt,ωπ3ω=π3=60∘ If angular velocities are in same sense: θ=ωt,2π+θ=5s,⇒θ=π/2

Topper's Solved these Questions

Similar Questions

Knowledge Check

A particle moves in a circular path of radius 0.5 m with a linear speed of 2 ms−1 ,its angular speed is

A particle moving along a circule path of radius 'r' with uniform angular velocity ω. Its angular acceleration is

CENGAGE PHYSICS-MISCELLANEOUS VOLUME 2-MCQ_TYPE

Two particles move on a circular path (one just inside and the other just outside) with the angular velocities $ω$ and $5 ω$ starting from the same point. Then

A particle moves in a circular path of radius 0.5 m with a linear speed of 2 $m s^{- 1}$ ,its angular speed is

A particle moving along a circule path of radius 'r' with uniform angular velocity $ω$ . Its angular acceleration is