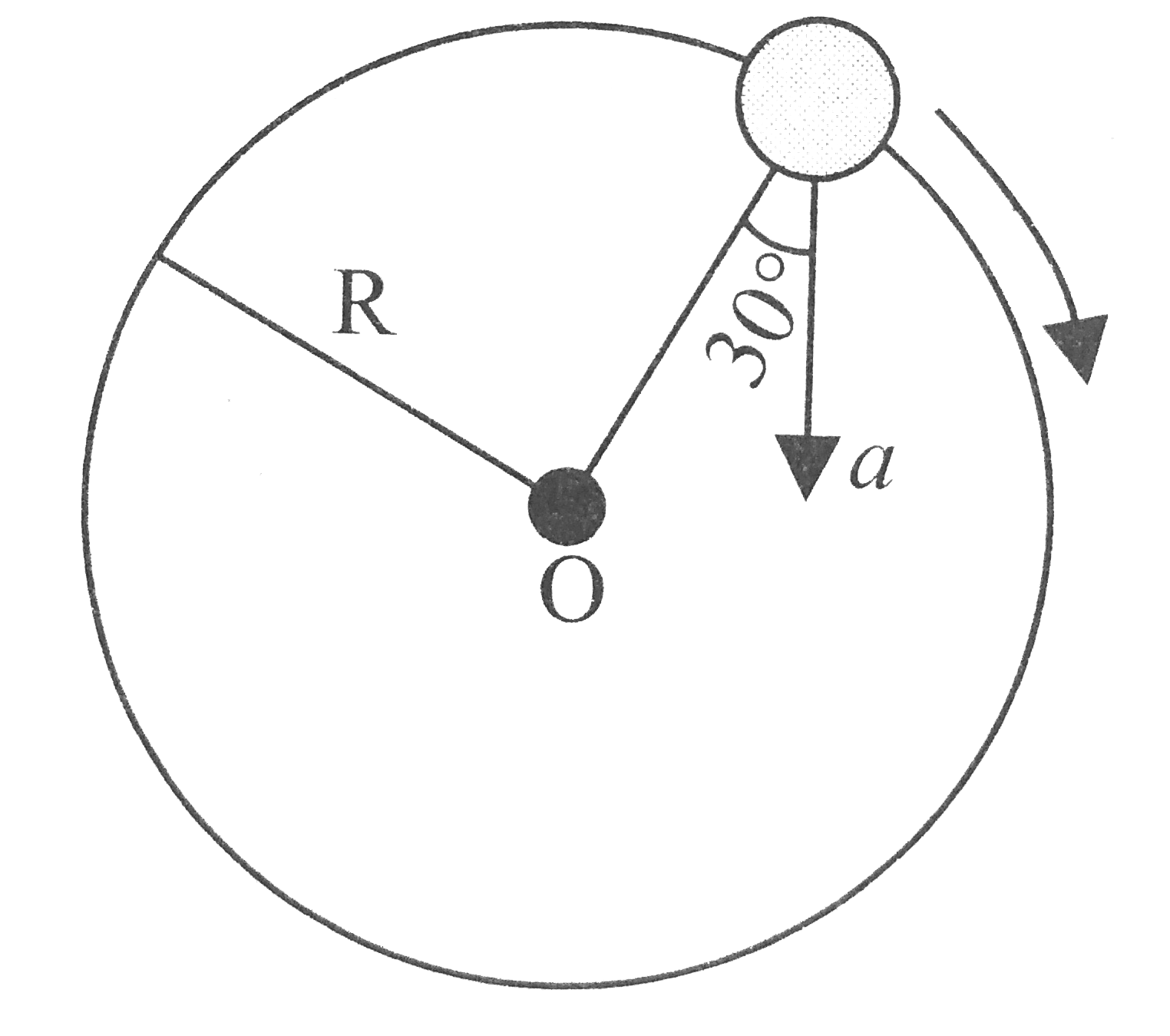
Figure shows the direction of the total acceleration and velocity of a particle moving clockwise in a circle radius m at an instant of time. Tangential acceleration this instant is 5m/s2. Which of the following statements is not correct?

The centripetal aceleration is 5√3ms−2
Particle is speeding up.
The net acceleration is 10ms−2
The particle is slowing down.
The correct Answer is:D
Since at and v are in the same direction, so the particle should be speeding up.

tan30∘=atac
⇒1√3=5ac⇒ac=5√3m/s2
Net acceleration is
a=√a2c+a2t=√(5√3)2+52=10m/s2
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - MCQ_TYPE
20 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - LC_TYPE
34 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
Similar Questions
Figure shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5 m at a given instant of time. At this instant, Find: (i) the radial acceleration, (ii) the speed of the particle and (iii) its tangential acceleration.
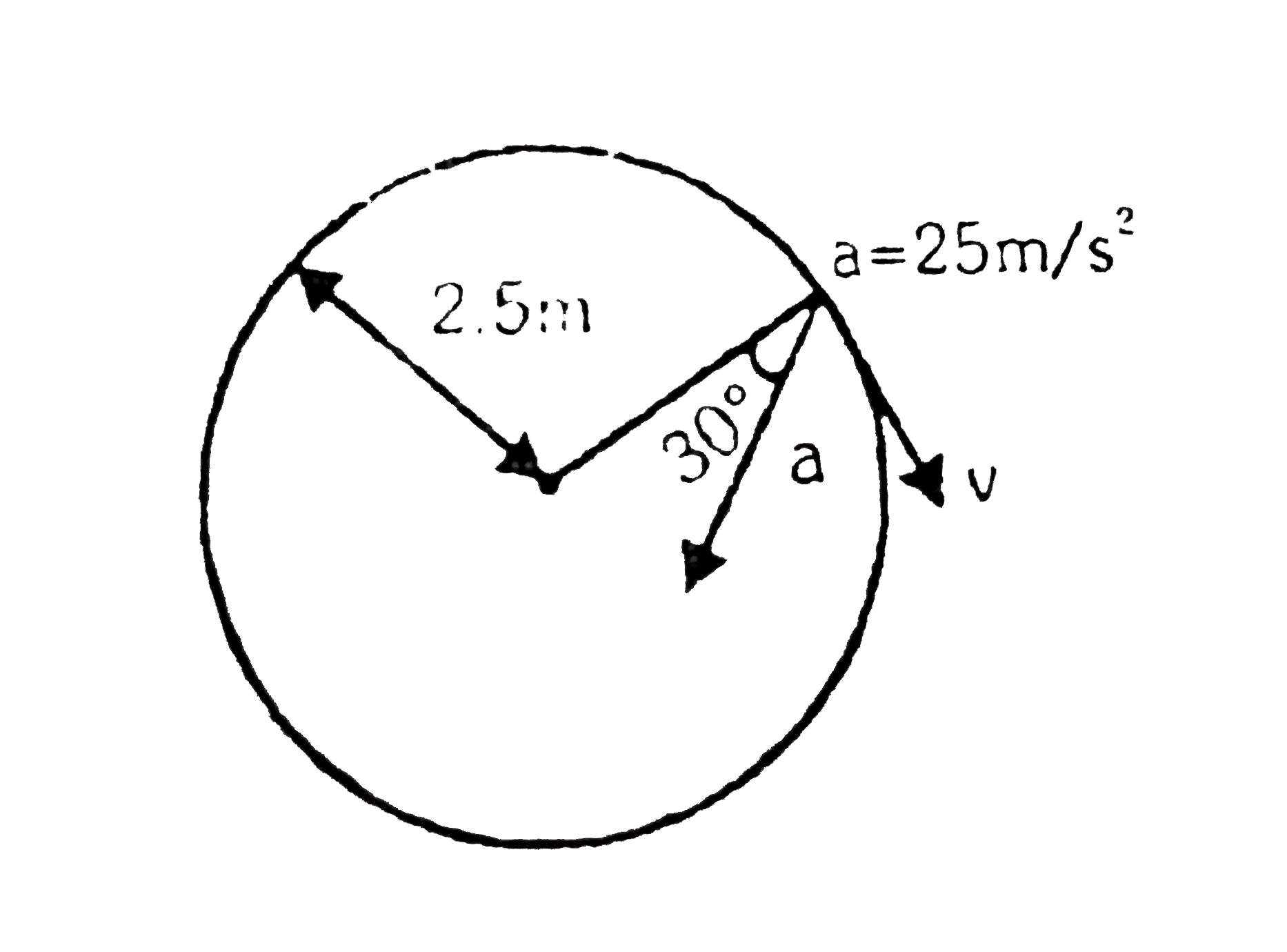
View SolutionFigur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
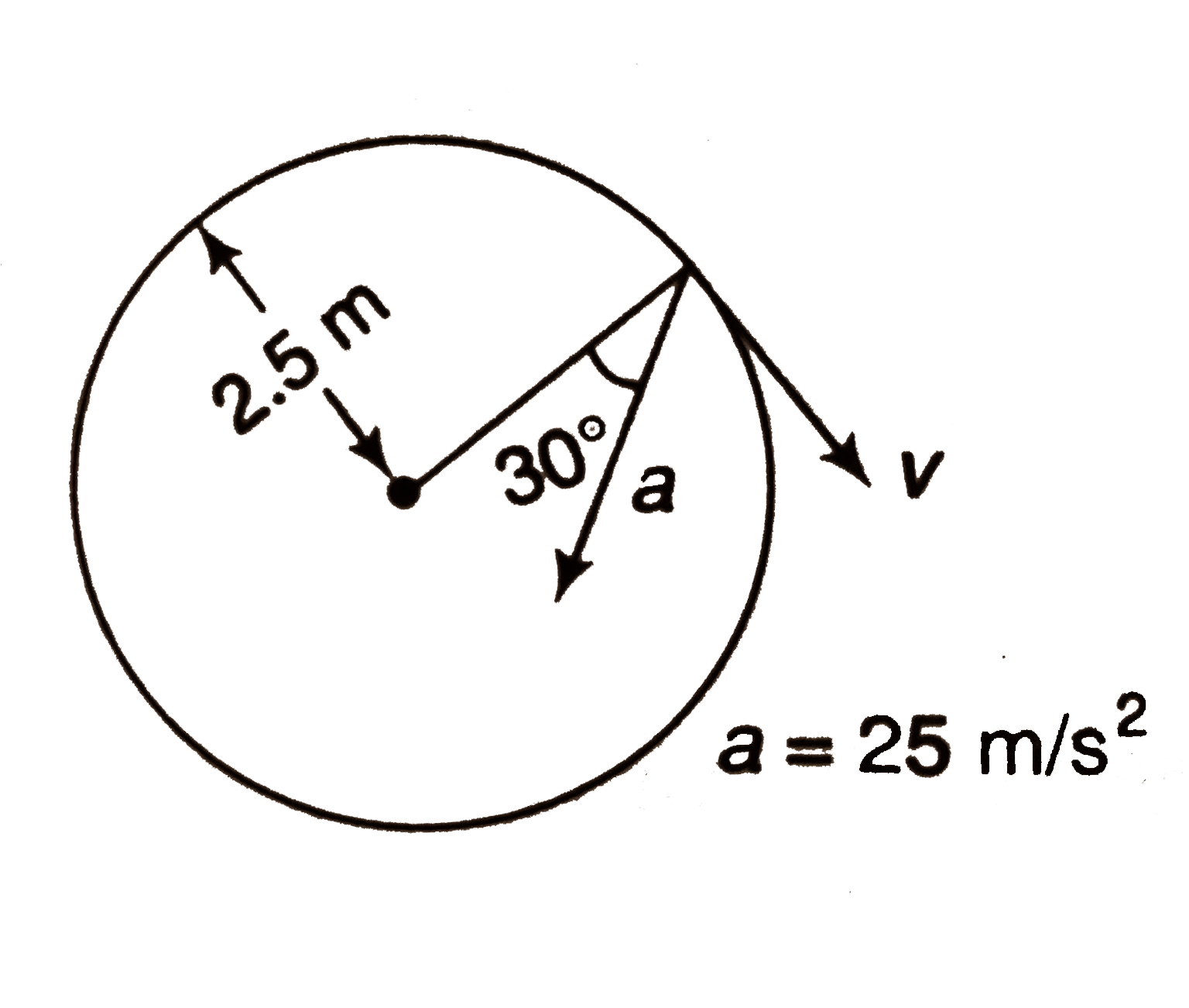
View Solution
Knowledge Check
In the given figure, a=15m/s2 represents the total acceleration of a particle moving in the clockwise dirction in a circle of radius R=2.5m at a given instant of time. The speed of the particle is
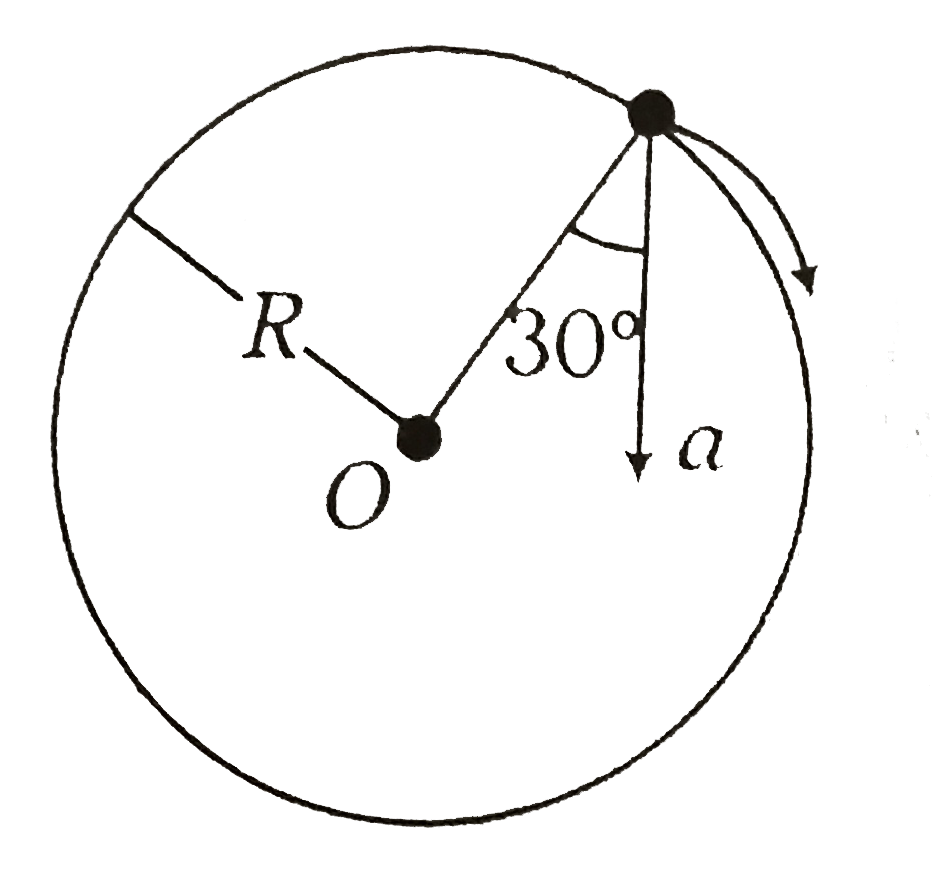 A5.7m/sB6.2m/sC4.5m/sD5.0m/s
A5.7m/sB6.2m/sC4.5m/sD5.0m/sThe figure shows the total acceleration a=32ms−2 of a moving particle moving clockwise in a circle of radius R = 1 m . What are the centripetal acceleration a and speed of the particle at the given instant ?
 A16√3ms−2,4√3ms−1B16√3ms−2,4ms−1C16ms−2,16ms−1D16ms−2,4ms−1
A16√3ms−2,4√3ms−1B16√3ms−2,4ms−1C16ms−2,16ms−1D16ms−2,4ms−1A particle moves in a circle of radius 2 m at a second
what is its resultant (total ) acceleration at time t=1 s ?A8m/s2B2√2m/s2C5√5m/s2D4√5m/s2
The instant of time at which acceleration and velocity are perpendicular is :-
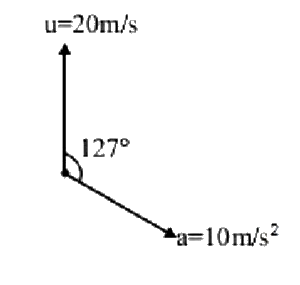
View SolutionIn th egiven figure,a=15m/s2 represents the total acceleration of particle moving in the clockwise direction in a circle of radius R=2.5m at a given instant of time. If square of speed of the particle is 75√3nm2/s2. Then write the value of n.

View SolutionA particle moves in a circle of radius 25 cm at two revolutions per sec. The acceleration of the particle in m/s2 is:
View SolutionIn the given figure, a=15m/s2 represents the total acceleration of a particle moving in the clockwise direction in a circle of radius R=2.5m at a given instant of time. The speed of the particle is.
View SolutionIn the given figure, a=15m/s2 represents the total acceleration of a particle moving in the clockwise dirction in a circle of radius R=2.5m at a given instant of time. The speed of the particle is

View Solution
