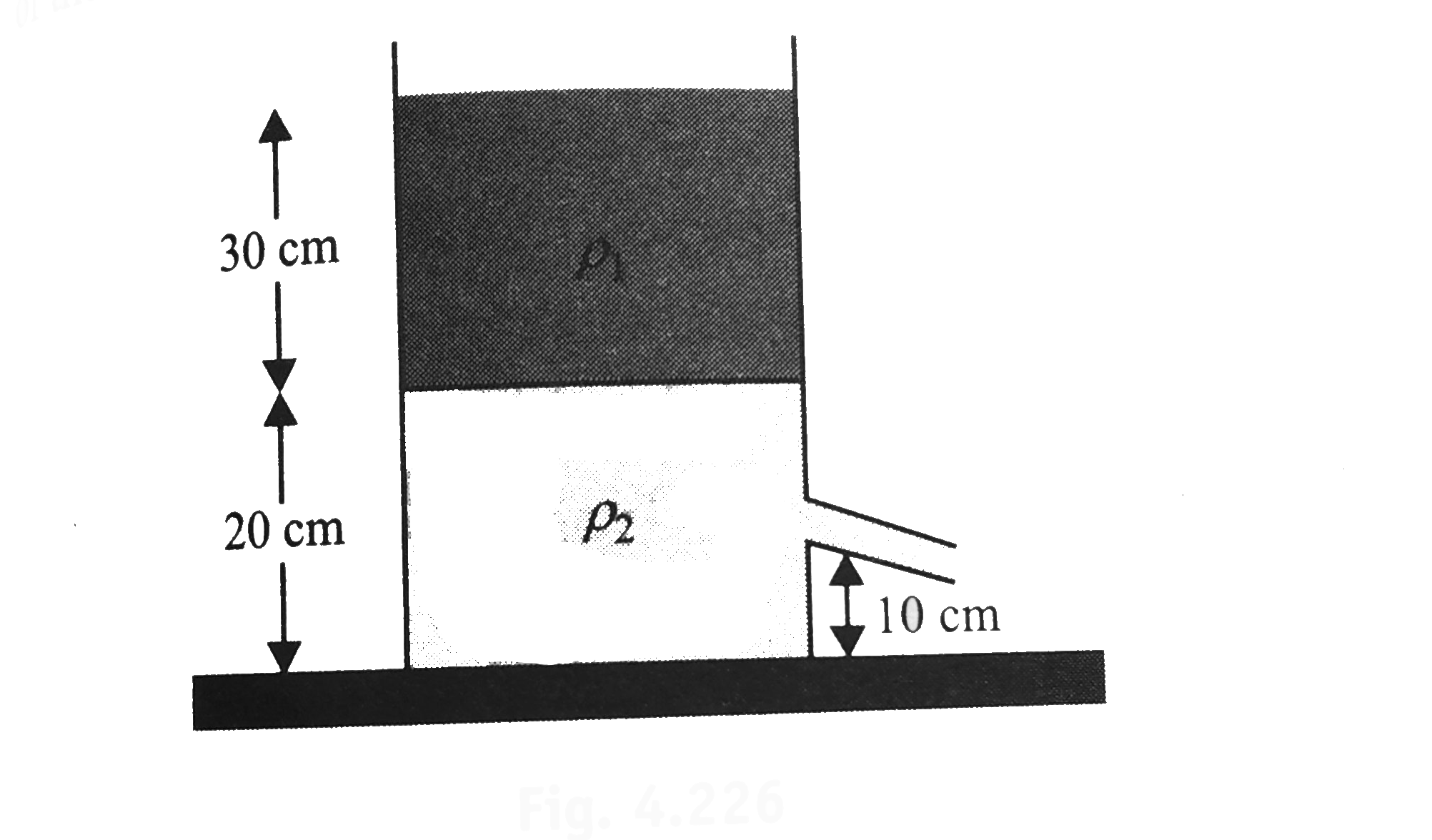
A cylindrical tank having cross-sectional area A=0.5m2 is filled with two liquids of density ρ1=900kgm−3 and ρ2=600kgm−3, to a height h=60cm each as shown in the figure. A small hole having area a=5cm2 is made in right vertical wall at a height y=20cm from the bottom. A horizontal force F is applied on the tank to keep it in static equilibrium. The tank is lying on a horizontal surface. Neglect mass of the cylindrical tank in comparison to mass of the liquids (akeg = 10 ms^(-2)).
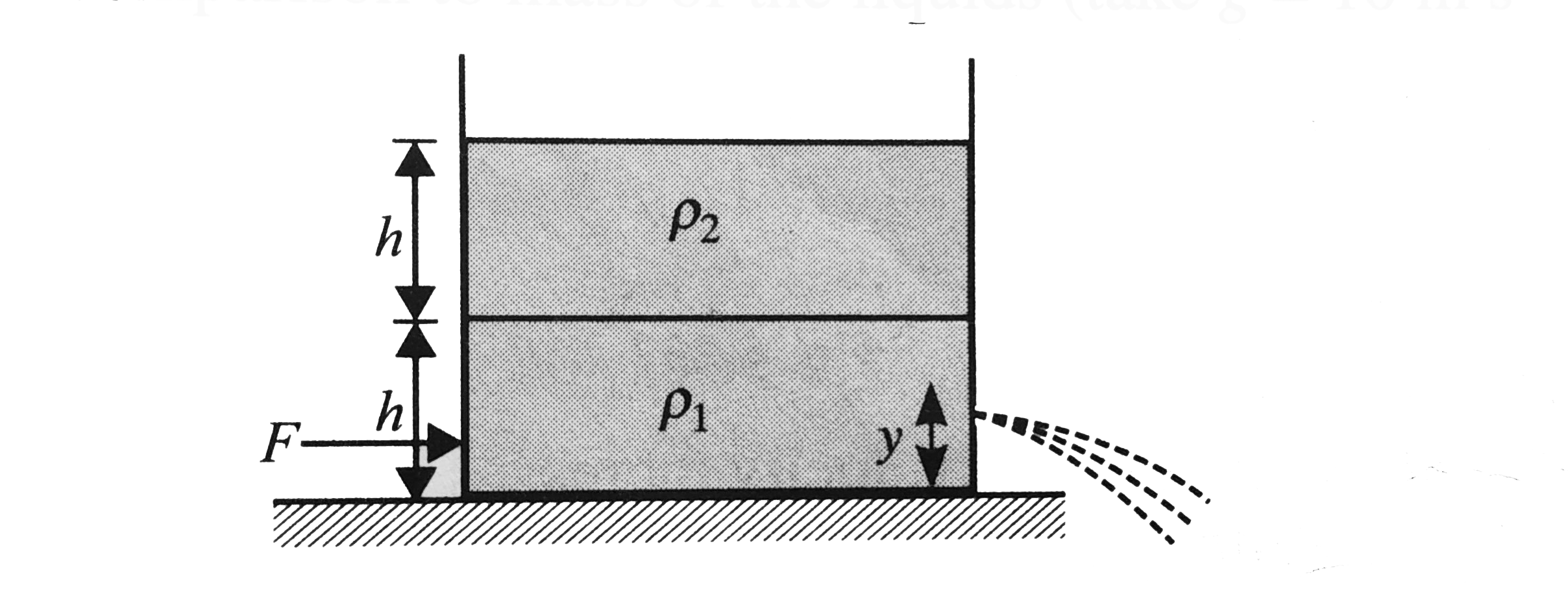
The velocity of efflux is
10ms−1
20ms−1
4ms−1
35ms−1
The correct Answer is:C
Since area of a hole is very small in comparison to base area A of the cylinder, velocity of liquid inside the cylinder is negligible.
Let velocity of effux be v and atmospheric pressure be P0. Consider two points A (inside the cylinder) and B (just outside the hole) in the same horizontal line as shown in the figure in question.
Pressure at A,PA=P0+hρ2g+(h−y)ρ1g
Pressure at B,PB=P0
According to Bernoulli's theorem
Pressuure energy at A= Pressure energy at B+ Kinetic energy at B
∴PA=PB+12ρ1v2
∴v=4ms−1
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - True/False
1 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - SCQ_TYPE
13 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning
8 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
_E01_229_Q01.png)
A cylinderical tank having cross sectional area ∧=0.5m2 is filled liquids of densities ρ1=900kgm3&ρ2=600kgm3 to a height h=60cm as shown in the figure a small hole having area a=5cm2 is made in right vertical wall at a height y=20cm from the bottom calculate.
(i). velocity of efflux
(ii). horizontal force F to keep the cylinder in static equilibrium if it is placed on a smooth horizontal plane
(iii). minimum and maximum value of F to keep the cylinder at rest. The coefficient of friction between cylinder and the plane is μ=0.1
(iv). velocity of the top most layer of the liquid column and also the velocity of the boundary separating the two liquids.
View SolutionA vessel is filled with two different liquids of densities ρand2ρ respectively as shown in the figure. The velocity of flow of liquid through a hole at height h2 from bottom is
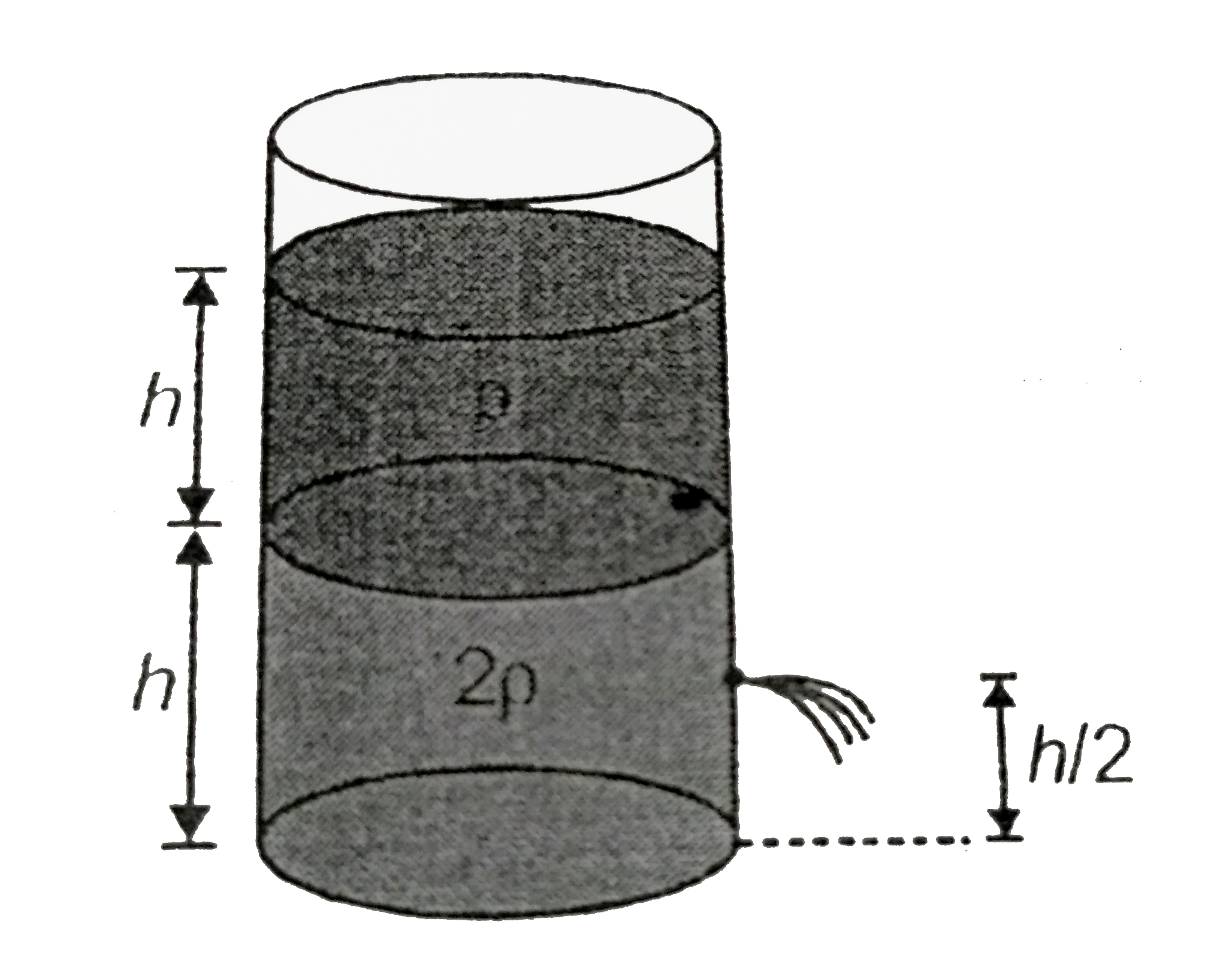
View Solution
Knowledge Check
A cylindrical tank having cross - sectional area A=0.5m2 is filled with two liquids of density ρ1=900 kg m−3andρ2=600 kg m−3 , to a height h =60cm2each.A small hole having area a=5 cm^2 is made in right vertical wall at a height y = 20 cm from the bottom . A horizontal force F is applied on the tank to keep it in static equilibrium . The tank is lying on a horizontal surfaces . Neglect mass of cylindrical tank camparison to mass of liquids ( take g=10ms−2)
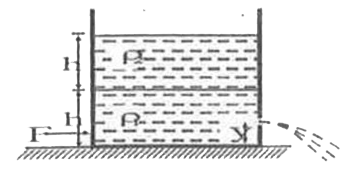
Horizontal force F to keep the cylinder in static equilibrium , if it is placed on a smooth horizontal thrust exerted by fluid jet . But that is equal to mass flowing per second × change in velocity of this mass
∴ F=(avρ)(v−0)=aρv2
or F=7.2NA7.2 NB10NC15.5ND20.4 NA cylindrical tank having cross - sectional area A=0.5m2 is filled with two liquids of density ρ1=900 kg m−3andρ2=600 kg m−3 , to a height a =6cm2 is made in right vertical wall at a height y = 20 cm from the bottom . A horizontal force F is applied on the tank to keep it in static equilibrium . The tank is lying on a horizontal surfaces . Neglect mass of cylindrical tank camparison to mass of liquids ( take g=10ms−2)
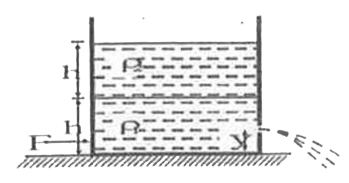
Minimum and maximum values of F to keep the cylinder in static just after the water starts to spill through the hole . If the co - efficient of static friction between contact surfaces is 0.01A0,40 NB5.4N , 52.2 NC0 , 70 ND0, 52.2 NA cylindrical vessel of a very large cross sectional area is containing two immiscible liquids of density ρ1=600kg/m3 and ρ2=1200kg/m3 as shown in the figure.
A small hole having cross sectional area 5cm2 is made in right side vertical wall as shown. Take atmospheric pressure as p0=105N/m2,g=10m/s2. For this situation mark out the correct statement (s). Take cross sectional area of the cylindrical vessel as 1000cm2. Neglect the mass of the vessel.
 AIf the surface on which the vessel is placed is smooth, then a rightward force of magnitude 3N is to be applied on the vessel to maintain its static equilibrium.BIf the surface on which the vessel is placed is smooth, then no force is needed to maintain its static equilibrium.CIf the surface on which the vessel is placed is rough (μ=0.04), then the minimum force (horizontal) needed to be applied on the vessel to maintain its static equilibrium is zero.DIf the surface on which the vessel is placed is rough (=0.04) then the maximum force (horizontal) needed to be applied on the vessel to maintain its static equilibrium is 19.8N.
AIf the surface on which the vessel is placed is smooth, then a rightward force of magnitude 3N is to be applied on the vessel to maintain its static equilibrium.BIf the surface on which the vessel is placed is smooth, then no force is needed to maintain its static equilibrium.CIf the surface on which the vessel is placed is rough (μ=0.04), then the minimum force (horizontal) needed to be applied on the vessel to maintain its static equilibrium is zero.DIf the surface on which the vessel is placed is rough (=0.04) then the maximum force (horizontal) needed to be applied on the vessel to maintain its static equilibrium is 19.8N.
A cube of 1 kg is floating at the interface of two liquids of density ρ1=3/4gm/cm3andρ2=2.5gm/cm3 The cube has (3/5)th part in ρ2 and (2/5)th part in ρ1 .Find the density of the cube .

View SolutionA long cylindrical tank of cross-sectional area 0.5m2 is filled with water. It has a small hole at a height 50cm from the bottom. A movable piston of cross-sectional area almost equal to 0.5m2 is fitted on the top of the tank such that it can slide in the tank freely. A load of 20kg is applied on the top of the water by piston, as shown in the figure. Calculate the speed of the water jet with which it hits the surface when piston is 1m above the bottom. (Ignore the mass of the piston)

View SolutionWater is filled in a container upto height 3m. A small hole of area 'a' is punched in the wall of the container at a height 52.5 cm from the bottom. The cross sectional area of the container is A. If a/A=0.1 then v2 is (where v is the velocity of water coming out of the hole)
View SolutionOil is filled in a cylindrical container up to height 4m. A small hole of area 'p' is punched in the wall of the container at a height 1.52m from the bottom. The cross sectional area of the container is Q. Ifpq=0.1 then v is (where vis the velocity of oil coming out of the hole)
View SolutionA cylindrical tank having cross-sectional area A is filled with water to a height of 2.0 m. A circular hole of corss-section area is opened at a height of 75 cm from the bottom. If a/A=√0.2, then velocity with which waer emerges from the hole is
(g=9.8ms−2)
View Solution
