A small body of density ρ' is dropped from rest at a height h into a lake of density ρ, where ρ>ρ’. Which of the following statements is/are correct if all dissipative effects are neglected (neglect viscosity)?
The speed of the body just entering the lake is √2gh
The body in the lake experiences upward acceleration equal to {(ρ/ρ')−1}g
The maximum depth to which the body sinks in the lake is hρ'(ρ−ρ')
The body does not come back to the surface of the lake.
The correct Answer is:A, B, C
By the principle of conservation of energy, we have
mgh=(12)mv2
or v=√2gh
Since ρ>p', the buoyant force of the lake is greater than the weight of the body. If a is the upward acceleation, then
ma=ρgV−ρ'gV or (ρ'V)a=ρgV−ρ'gV
Cancelling V on the both the sides, we get
a=g(ρ−ρ'ρ)=g(ρρ'−1)
Now v2=v20+2ay
where v0 is the velocity at y=0,v=0 is the velocity at the maximm depth and y is the negative displacement from the surface on the maximum depth. With these get v20=−2ay.
−yv202a=2gh2g((ρ−ρ'))ρ'=hρ'ρ−ρ'
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning
8 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Subjective
25 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A ball of density ρ0 falls from rest from a point P onto the surface of a liquid of density ρ in the time T. It enters the liquid, stops, moves up, and returns to P in a total time 3 T. neglect viscosity, surface tension and splashing find the ratio of ρρ0
View SolutionA small ball of density ρ is placed from the bottom of a tank in which a non viscous liquid of density 2ρ is filled . Find the time taken by the ball to reach the surface of liquid and maximum height reached above the bottom of container . (Size of the ball and any type of drag force acting on the ball are negligible )
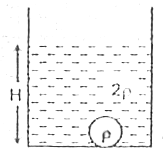
View Solution
Knowledge Check
A body of density d2 is dropped from rest at a height x into a beaker having a liquid of density d1(d2>d1). Which of the following is/are true?
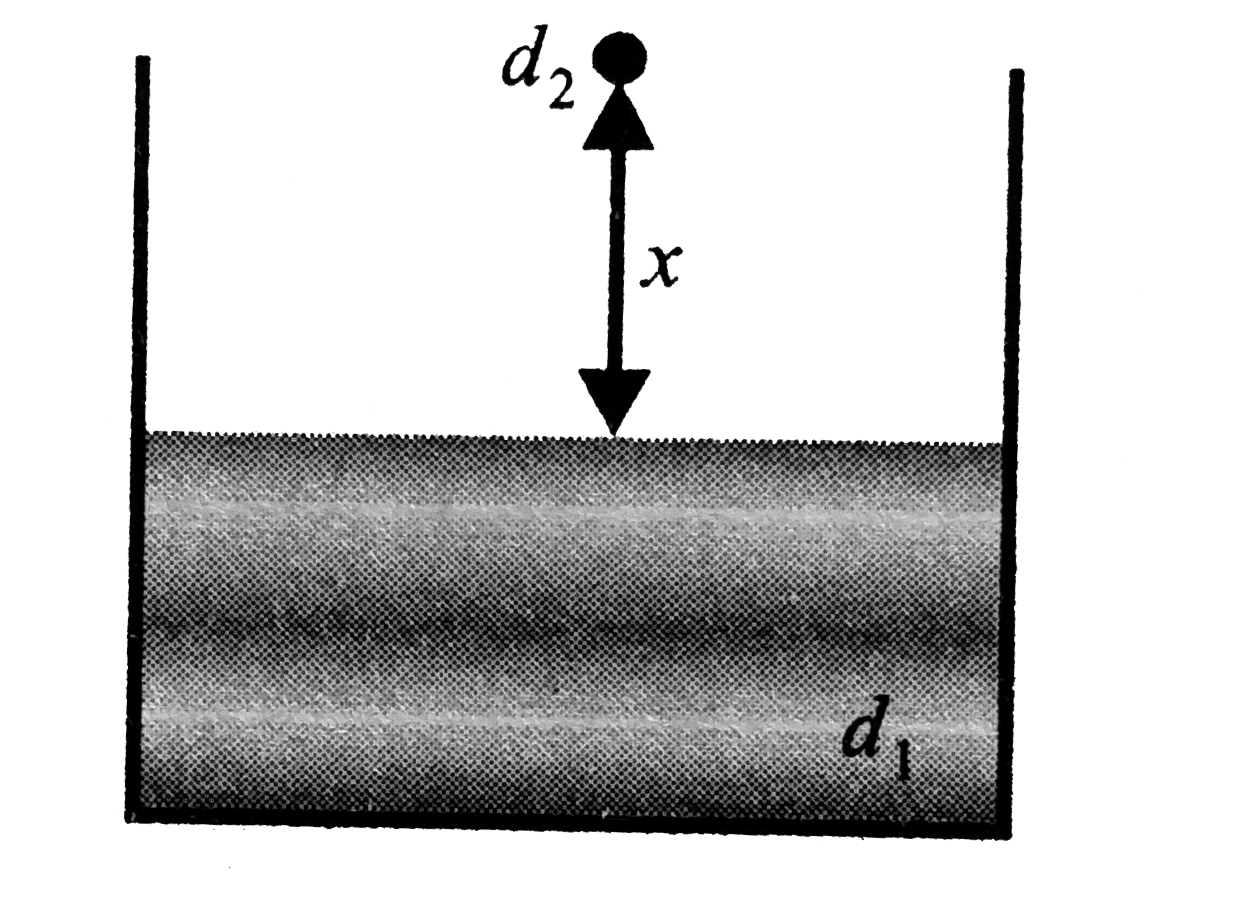 AThe speed with which the body just enters the liquid is √2gxBThe body has an acceleration of g(1−d1d2) downwardsCThe body does not come back to the surface of the liquid (observation made after a long time).Dnone
AThe speed with which the body just enters the liquid is √2gxBThe body has an acceleration of g(1−d1d2) downwardsCThe body does not come back to the surface of the liquid (observation made after a long time).DnoneA body of density ρ is dropped from reat from a height h into a lake of density σ(σ>ρ). The maximum depth the body sinks inside the liquid is (neglect viscous effect of liquid)
Ahρσ−ρBhσσ−ρChρσDhσρA body of density ρ is dropped from rest from height h (from the surface of water) into a lake of density of water σ(σ>ρ). Neglecting all disspative effects, the acceleration of body while it is in the take is
Ag(σρ−1) upwardsBg(σρ−1) downwardsCg(σρ) upwardsDg(σρ) downwards
An object of density σ(<ρ) is dropped from rest at a height h into a liquid of density ρ kept in a tall vertical cylindrical container. Neglect all dissipative effects and assume that there is no spilling of the liquid.
The time taken by the object to reach the starting point (from which it is dropped) is
View SolutionA ball of density ρ is dropped from a height on the suraca of a non-visous liquid of dinsity 2ρ. Choose the correct options.

View Solutionbody of density ρ is dropped from height h into a liquid having density σ(σ>ρ) .If the body just touches the of the container, then the distance of fallen would be proprotional to (Neglect viscous forces)
View SolutionA liquid drop of density ρ , radius r, and surface tension σ oscillates with time period T . Which of the following expressions for T2 is correct?
View SolutionA beaker is filled with a liquid of density ρ upto a height h If the beaker is at rest, the mean pressure at the walls is:
View Solution
