A wooden block is floating in a liquid. About 50% of its volume is inside the liquid when the vessel is stationary, Percentage volume immersed when the vessel moves upwards with acceleration a=g.2 is
75%
25%
50%
33.33%
The correct Answer is:C
To solve the problem of determining the percentage of the volume of a wooden block that is immersed in a liquid when the vessel is moving upwards with an acceleration of a=g/2, we can follow these steps:
Step 1: Understand the Initial Condition
When the vessel is stationary, 50% of the volume of the wooden block is submerged in the liquid. This means that the weight of the block is balanced by the buoyant force acting on it.
Step 2: Establish the Relationship at Rest
Let:
- VB = total volume of the block
- VI = volume of the block immersed in the liquid
- ρB = density of the block
- ρL = density of the liquid
From the problem, we know:
VI=0.5VB
Using the principle of buoyancy:
Weight of block=Buoyant force
VB⋅ρB⋅g=VI⋅ρL⋅g
Substituting VI=0.5VB:
VB⋅ρB⋅g=0.5VB⋅ρL⋅g
Cancelling g and VB (assuming VB≠0):
ρB=0.5ρL
This implies:
ρLρB=2
Step 3: Analyze the Condition When Vessel Moves Upwards
Now, when the vessel accelerates upwards with a=g/2, we need to consider the forces acting on the block.
Step 4: Set Up the Equation of Motion
The forces acting on the block are:
- Buoyant force FB=VI⋅ρL⋅g (upwards)
- Weight of the block W=VB⋅ρB⋅g (downwards)
Using Newton's second law:
FB−W=M⋅a
Where M is the mass of the block:
VB⋅ρB⋅g
Thus:
VI⋅ρL⋅g−VB⋅ρB⋅g=VB⋅ρB⋅g2
Step 5: Rearrange the Equation
Rearranging gives:
VI⋅ρL⋅g=VB⋅ρB⋅g+VB⋅ρB⋅g2
VI⋅ρL=VB⋅ρB(1+12)
VI⋅ρL=VB⋅ρB⋅32
Step 6: Solve for the Immersed Volume
Now, substituting ρB=0.5ρL:
VI⋅ρL=VB⋅(0.5ρL)⋅32
Cancelling ρL (assuming ρL≠0):
VI=VB⋅0.5⋅32
VI=34VB
Step 7: Calculate the Percentage Immersed
The percentage of the volume immersed is:
Percentage immersed=(VIVB)×100=(3/4VBVB)×100=75%
Final Answer
The percentage volume immersed when the vessel moves upwards with acceleration a=g/2 is 75%.
---
When the vessel is stationary, 50% of the volume of the wooden block is submerged in the liquid. This means that the weight of the block is balanced by the buoyant force acting on it.
Let:
- VB = total volume of the block
- VI = volume of the block immersed in the liquid
- ρB = density of the block
- ρL = density of the liquid
VI=0.5VB
Weight of block=Buoyant force
VB⋅ρB⋅g=VI⋅ρL⋅g
Substituting VI=0.5VB:
VB⋅ρB⋅g=0.5VB⋅ρL⋅g
Cancelling g and VB (assuming VB≠0):
ρB=0.5ρL
This implies:
ρLρB=2
Now, when the vessel accelerates upwards with a=g/2, we need to consider the forces acting on the block.
The forces acting on the block are:
- Buoyant force FB=VI⋅ρL⋅g (upwards)
- Weight of the block W=VB⋅ρB⋅g (downwards)
FB−W=M⋅a
Where M is the mass of the block:
VB⋅ρB⋅g
Thus:
VI⋅ρL⋅g−VB⋅ρB⋅g=VB⋅ρB⋅g2
Rearranging gives:
VI⋅ρL⋅g=VB⋅ρB⋅g+VB⋅ρB⋅g2
VI⋅ρL=VB⋅ρB(1+12)
VI⋅ρL=VB⋅ρB⋅32
Now, substituting ρB=0.5ρL:
VI⋅ρL=VB⋅(0.5ρL)⋅32
Cancelling ρL (assuming ρL≠0):
VI=VB⋅0.5⋅32
VI=34VB
The percentage of the volume immersed is:
Percentage immersed=(VIVB)×100=(3/4VBVB)×100=75%
The percentage volume immersed when the vessel moves upwards with acceleration a=g/2 is 75%.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning
8 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Subjective
25 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A wooden block is floating in a liquid, 50% of its volume is inside the liquid when the vessel is stationary. Percentage of volume immersed when the vessel moves upwards with an acceleration a=g2 is
View SolutionA cubical block is floating in a liquid with half of its volume immersed in the liquid.When the whole system accelerates upward with a net acceleration of g/2 the fraction of volume immersed in the liquid will become
View Solution
Knowledge Check
A plank is floating in a liquid as shown, Fraction f of its volume is immersed. Choose the correct options
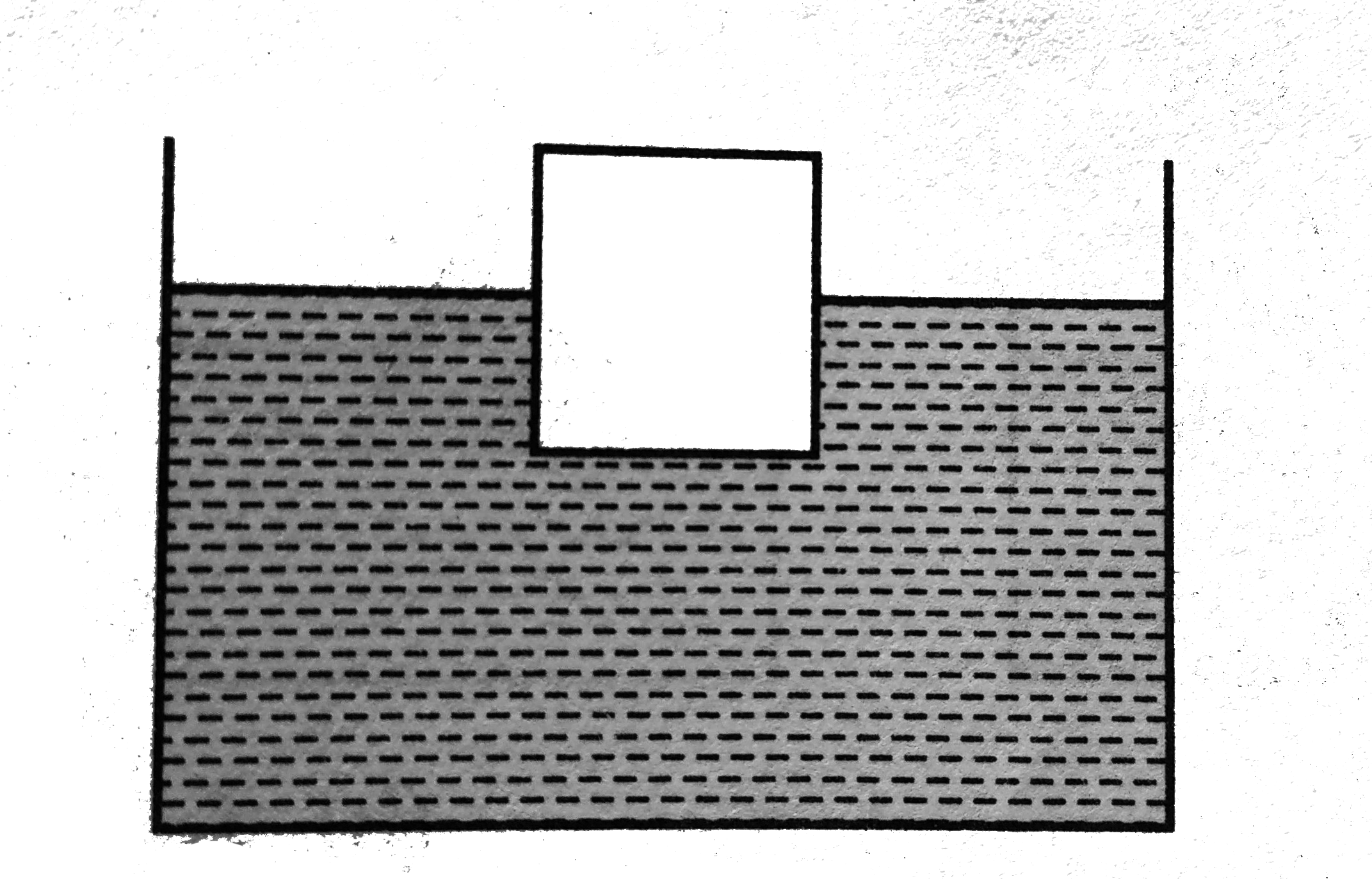 AIf the system is taken to a place where atmospheric pressure is more. F will increaseBIn above condition f will remain unchangedCIf temperature is increasesd and expansion of only liquid is considered f will increasesDIf temperature is increased and expansion of only plank is considered f will decrease
AIf the system is taken to a place where atmospheric pressure is more. F will increaseBIn above condition f will remain unchangedCIf temperature is increasesd and expansion of only liquid is considered f will increasesDIf temperature is increased and expansion of only plank is considered f will decreaseA cubical block is floating in a liquid with half of its volume immersed in the liquid. When the whole system accelerates upwards with acceleration of g/3, the fraction of volume immersed in the liquid will be
 A12B38C23D34
A12B38C23D34A cubical block is floating in a liquid with one fourth o its volume immersed in the liquid. If whole of the system accelerates upward with acceleration g/4, the fraction of volume immersed in the liquid will be :-
A1/4B1/2C3/4D2/3
A cubical block is floating in a liquid with half of its volume immersed in the liquid.When the whole system accelerates upward with a net acceleration of g/2 the fraction of volume immersed in the liquid will become
View SolutionA cubical block is floating in a liquid with half of its volume immersed in the liquid. When the whole system acelerates upwards with a net acceleration of g/3. The fraction of volume immersed in the liquid will be 3x Find x.
View SolutionA body of mass 6 kg is floating in a liquid with 2/3 of its volume inside the liquid.Find ratio between the density of body and density of liquid. Take g=10ms−2.
View SolutionA cubical block is floating in a liquid with half of its volume immersed in the liquid. When the whole system accelerates upwards with a net acceleration of g/4, the fraction of volume immersed in the liquid will be
View SolutionA block of density ρ floats in a liquid with its one third volume immersed. The density of the liquid is
View Solution
